Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a.
\(y=2sinx-\left(1-sin^2x\right)=sin^2x+2sinx-1=\left(sinx+1\right)^2-2\ge-2\)
\(\Rightarrow y_{min}=-2\)
\(y=sin^2x+2sinx-1=\left(sinx-1\right)\left(sinx+3\right)+2\le2\)
\(\Rightarrow y_{max}=2\)
b.
\(1\le3-2sinx\le5\Rightarrow6\le y\le5+\sqrt{5}\)
\(y_{min}=6\) ; \(y_{max}=5+\sqrt{5}\)

a/ \(y=2\left(\frac{\sqrt{3}}{2}sinx-\frac{1}{2}cosx\right)+5=2sin\left(x-\frac{\pi}{6}\right)+5\)
Do \(-1\le sin\left(x-\frac{\pi}{6}\right)\le1\Rightarrow3\le y\le7\)
b/ \(y=2cos\left(x+\frac{\pi}{6}\right)cos\left(-\frac{\pi}{6}\right)=\sqrt{3}cos\left(x+\frac{\pi}{6}\right)\)
Do \(-1\le cos\left(x+\frac{\pi}{6}\right)\le1\Rightarrow-\sqrt{3}\le y\le\sqrt{3}\)
c/ \(y=2\left(\frac{1}{2}sinx+\frac{\sqrt{3}}{2}cosx\right)+12=2sin\left(x+\frac{\pi}{3}\right)+12\)
Do \(-1\le sin\left(x+\frac{\pi}{3}\right)\le1\Rightarrow10\le y\le14\)

áp dụng bất đẳng thức Bunhia ta có :
\(\left(x+4y\right)^2\le\left(5^2+12^2\right)\left(\frac{x^2}{25}+\frac{y^2}{9}\right)=169\)
Vậy \(-13\le x+4y\le13\Rightarrow-8\le P\le18\)
vậy min bằng -8
max bằng 18

a/ \(0\le cos^2x\le1\Rightarrow2\le y\le\sqrt{7}\)
\(y_{min}=2\) khi \(cos^2x=1\)
\(y_{max}=\sqrt{7}\) khi \(cos^2x=0\)
b/ \(y=\frac{2}{1+tan^2x}=\frac{2}{\frac{1}{cos^2x}}=2cos^2x\le2\)
\(\Rightarrow y_{max}=2\) khi \(cos^2x=1\)
\(y_{min}\) ko tồn tại
c/ \(y=1-cos2x+\sqrt{3}sin2x=2\left(\frac{\sqrt{3}}{2}sin2x-\frac{1}{2}cos2x\right)+1\)
\(y=2sin\left(2x-\frac{\pi}{6}\right)+1\)
Do \(-1\le sin\left(2x-\frac{\pi}{6}\right)\le1\Rightarrow-1\le y\le3\)

Ta có:
\(-1\le\sin2x\le1\)
=> \(\sqrt{4-2.\left(1\right)^5}-8\le\sqrt{4-2.\left(\sin2x\right)^5}-8\le\sqrt{4-2.\left(-1\right)^5}-8\)
=> \(\sqrt{2}-8\le\sqrt{4-2.\left(\sin2x\right)^5}-8\le\sqrt{6}-8\)
=> tìm ddc min và max

\(0\le cos^2\left(x-\frac{\pi}{4}\right)\le1\Rightarrow1\le y\le2\)
\(y_{min}=1\) khi \(cos\left(x-\frac{\pi}{4}\right)=0\)
\(y_{max}=2\) khi \(cos^2\left(x-\frac{\pi}{4}\right)=1\)
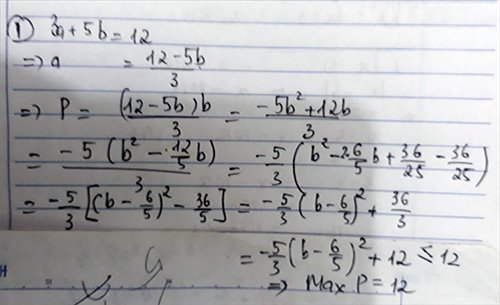



Suy ra: min y= -1; max y= 5
Đáp án C