Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(PT\Leftrightarrow2+\frac{1}{sinxcosx}-cotx=-2-sinx-cosxcotx-tanx\)
\(\Leftrightarrow\frac{1}{sinxcosx}-\frac{cosx}{sinx}=-2sinx-\frac{sinx}{cosx}\)
\(\Leftrightarrow1-cos^2x+2sin^2xcosx+sin^2x=0\)
\(\Leftrightarrow2sin^2x+2sin^2xcosx=0\)
\(\Leftrightarrow2sin^2x\left(1+cosx\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sin^2x=0\\cosx=-1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}cosx=1\\cosx=-1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2k\pi\\x=\pi+2k\pi\end{matrix}\right.\Leftrightarrow x=k\pi\)
Mình sửa lại câu trả lời
ĐK:\(\left\{{}\begin{matrix}sinx\ne0\\cosx\ne0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne k\pi\\x\ne\frac{\pi}{2}+k\pi\end{matrix}\right.\)
\(PT\Leftrightarrow2sin^2x\left(1+cosx\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=0\left(loai\right)\\cosx=-1\end{matrix}\right.\)
\(\Leftrightarrow x=\pi+2k\pi\left(loai\right)\)
Vậy phương trình vô nghiệm

tham khảo:
a)\(y'=xsin2x+sin^2x\)
\(y'=sin^2x+xsin2x\)
b)\(y'=-2sin2x+2cosx\\ y'=2\left(cosx-sin2x\right)\)
c)\(y=sin3x-3sinx\)
\(y'=3cos3x-3cosx\)
d)\(y'=\dfrac{1}{cos^2x}-\dfrac{1}{sin^2x}\)
\(y'=\dfrac{sin^2x-cos^2x}{sin^2x.cos^2x}\)

a.
\(y'=\dfrac{3}{cos^2\left(3x-\dfrac{\pi}{4}\right)}-\dfrac{2}{sin^2\left(2x-\dfrac{\pi}{3}\right)}-sin\left(x+\dfrac{\pi}{6}\right)\)
b.
\(y'=\dfrac{\dfrac{\left(2x+1\right)cosx}{2\sqrt{sinx+2}}-2\sqrt{sinx+2}}{\left(2x+1\right)^2}=\dfrac{\left(2x+1\right)cosx-4\left(sinx+2\right)}{\left(2x+1\right)^2}\)
c.
\(y'=-3sin\left(3x+\dfrac{\pi}{3}\right)-2cos\left(2x+\dfrac{\pi}{6}\right)-\dfrac{1}{sin^2\left(x+\dfrac{\pi}{4}\right)}\)

a. ĐKXĐ: ...
\(\frac{sinx}{cosx}+\frac{sin2x}{cos2x}+\frac{sin3x}{cos3x}=0\)
\(\Leftrightarrow\frac{sin2x.cosx+cos2x.sinx}{cosx.cos2x}+\frac{sin3x}{cos3x}=0\)
\(\Leftrightarrow\frac{sin3x}{cosx.cos2x}+\frac{sin3x}{cos3x}=0\)
\(\Leftrightarrow sin3x\left(\frac{cosx.cos2x+cos3x}{cosx.cos2x.cos3x}\right)=0\)
\(\Leftrightarrow sin3x\left(\frac{cosx\left(2cos^2x-1\right)+4cos^3x-3cosx}{cosx.cos2x.cos3x}\right)=0\)
\(\Leftrightarrow sin3x\left(\frac{6cos^2x-4}{cos2x.cos3x}\right)=0\)
\(\Leftrightarrow sin3x\left(\frac{3cos2x-1}{cos2x.cos3x}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sin3x=0\\cos2x=\frac{1}{3}\end{matrix}\right.\)
b.
\(cos2x\left(2cos^22x-1\right)=\frac{1}{2}\)
\(\Leftrightarrow4cos^32x-2cos2x-1=0\)
Pt bậc 3 này ko giải được, chắc bạn ghi nhầm đề
c. ĐKXĐ: ...
\(\frac{cosx}{sinx}-\frac{sinx}{cosx}=cosx-sinx\)
\(\Leftrightarrow\frac{\left(cosx-sinx\right)\left(cosx+sinx\right)}{sinx.cosx}=cosx-sinx\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx-sinx=0\Rightarrow x=...\\\frac{cosx+sinx}{sinx.cosx}=1\left(1\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow cosx+sinx=sinx.cosx\)
Đặt \(sinx+cosx=t\Rightarrow\left\{{}\begin{matrix}\left|t\right|\le\sqrt{2}\\sinx.cosx=\frac{t^2-1}{2}\end{matrix}\right.\)
\(\Rightarrow t=\frac{t^2-1}{2}\Rightarrow t^2-2t-1=0\Rightarrow\left[{}\begin{matrix}t=1+\sqrt{2}\left(l\right)\\t=1-\sqrt{2}\end{matrix}\right.\)
\(\Rightarrow\sqrt{2}sin\left(x+\frac{\pi}{4}\right)=1-\sqrt{2}\Rightarrow sin\left(x+\frac{\pi}{4}\right)=\frac{1-\sqrt{2}}{\sqrt{2}}\Rightarrow x=...\)

a) Đặt \(u = 3{\rm{x}}\) thì \(y = \sin u\). Ta có: \(u{'_x} = {\left( {3{\rm{x}}} \right)^\prime } = 3\) và \(y{'_u} = {\left( {\sin u} \right)^\prime } = \cos u\).
Suy ra \(y{'_x} = y{'_u}.u{'_x} = \cos u.3 = 3\cos 3{\rm{x}}\).
Vậy \(y' = 3\cos 3{\rm{x}}\).
b) Đặt \(u = \cos 2{\rm{x}}\) thì \(y = {u^3}\). Ta có: \(u{'_x} = {\left( {\cos 2{\rm{x}}} \right)^\prime } = - 2\sin 2{\rm{x}}\) và \(y{'_u} = {\left( {{u^3}} \right)^\prime } = 3{u^2}\).
Suy ra \(y{'_x} = y{'_u}.u{'_x} = 3{u^2}.\left( { - 2\sin 2{\rm{x}}} \right) = 3{\left( {\cos 2{\rm{x}}} \right)^2}.\left( { - 2\sin 2{\rm{x}}} \right) = - 6\sin 2{\rm{x}}{\cos ^2}2{\rm{x}}\).
Vậy \(y' = - 6\sin 2{\rm{x}}{\cos ^2}2{\rm{x}}\).
c) Đặt \(u = \tan {\rm{x}}\) thì \(y = {u^2}\). Ta có: \(u{'_x} = {\left( {\tan {\rm{x}}} \right)^\prime } = \frac{1}{{{{\cos }^2}x}}\) và \(y{'_u} = {\left( {{u^2}} \right)^\prime } = 2u\).
Suy ra \(y{'_x} = y{'_u}.u{'_x} = 2u.\frac{1}{{{{\cos }^2}x}} = 2\tan x\left( {{{\tan }^2}x + 1} \right)\).
Vậy \(y' = 2\tan x\left( {{{\tan }^2}x + 1} \right)\).
d) Đặt \(u = 4 - {x^2}\) thì \(y = \cot u\). Ta có: \(u{'_x} = {\left( {4 - {x^2}} \right)^\prime } = - 2{\rm{x}}\) và \(y{'_u} = {\left( {\cot u} \right)^\prime } = - \frac{1}{{{{\sin }^2}u}}\).
Suy ra \(y{'_x} = y{'_u}.u{'_x} = - \frac{1}{{{{\sin }^2}u}}.\left( { - 2{\rm{x}}} \right) = \frac{{2{\rm{x}}}}{{{{\sin }^2}\left( {4 - {x^2}} \right)}}\).
Vậy \(y' = \frac{{2{\rm{x}}}}{{{{\sin }^2}\left( {4 - {x^2}} \right)}}\).

a) Cách 1: y' = (9 -2x)'(2x3- 9x2 +1) +(9 -2x)(2x3- 9x2 +1)' = -2(2x3- 9x2 +1) +(9 -2x)(6x2 -18x) = -16x3 +108x2 -162x -2.
Cách 2: y = -4x4 +36x3 -81x2 -2x +9, do đó
y' = -16x3 +108x2 -162x -2.
b) y' = .(7x -3) +
(7x -3)'=
(7x -3) +7
.
c) y' = (x -2)'√(x2 +1) + (x -2)(√x2 +1)' = √(x2 +1) + (x -2) = √(x2 +1) + (x -2)
= √(x2 +1) +
=
.
d) y' = 2tanx.(tanx)' - (x2)' =
.
e) y' = sin
=
sin
.
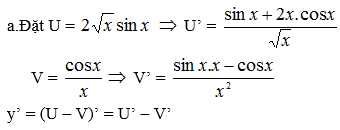
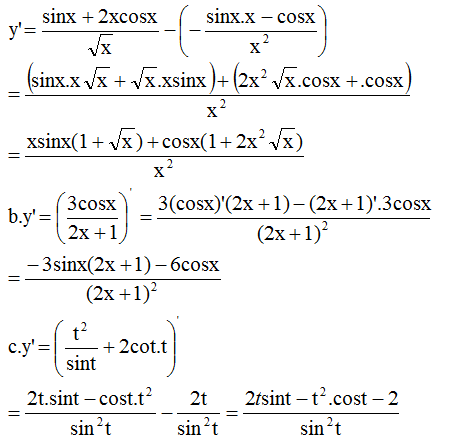
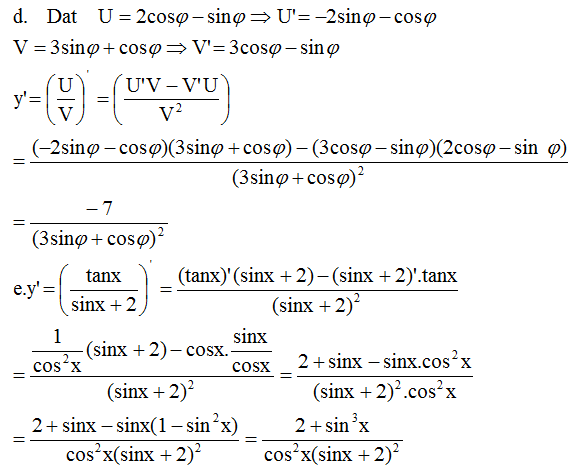


y = tan x + cot x ⇒ y ' = ( tan x + cot x ) ' = ( tan x ) ' + ( cot x ) ' = 1 cos 2 x − 1 sin 2 x
Chọn đáp án D