Tìm giá trị lớn nhất của hàm số y = x...

Khách

Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Mua vip

Pham Trong Bach

5 tháng 7 2018

Tìm giá trị lớn nhất của hàm số y = x + sin 2 x trên đoạn 0 , π

A. 0

B. π

C . 3 π 4 + 1 2

D . 3 π 4

#Toán lớp 11

Cao Minh Tâm

5 tháng 7 2018

Đúng(0)

Các câu hỏi dưới đây có thể giống với câu hỏi trên

Nguyễn Hoàng

7 tháng 6 2019

Giải phương trình

1 : sin2x = cos3x

2 : cos(2x - $\frac{\text{π}}{4}$ ) + sin(x+ $\frac{\text{π}}{4}$) = 0

#Toán lớp 11

Ami Mizuno

7 tháng 6 2019

Bạn tham khảo thử nhé

Đúng(0)

Kim Duyên

23 tháng 8 2016

Tìm nghiệm của các phương trình sau trong khoảng đã cho

a) sin2x = -$\frac{1}{2}$ với 0<x<π ;

b) cos(x-5) = $\frac{\sqrt{3}}{2}$ với -π< x < π.

#Toán lớp 11

Gia Khanh

1 tháng 9 2016

- Giải phương trình : cos ( x - $_{^{ }15}o$) = $\frac{\sqrt{2}}{2}$

- Giải các phương trình sau và tìm các nghiệm trong đoạn [ 0;π ]

1. sin ( 3x+1)=sin(x-2)

2. sin ( x - $^{120^o}$ )+ cos2x=0

3. sin3x + sin ( $\frac{\pi}{4}$ - $\frac{x}{2}$ ) = 0

#Toán lớp 11

Bình Trần Thị

11 tháng 9 2016

vẽ đồ thị hàm số $y=\cot x$ rồi chỉ ra trên đồ thị đó các điểm có hoành độ thuộc khoảng (−π;π)(−π;π) là nghiệm của mỗi phương trình sau : 1) $\cot x=\frac{\sqrt{3}}{3}$ ; 2) \(\cot...

Đọc tiếp

#Toán lớp 11

Triều Trương Quang

21 tháng 9 2019

Tìm đồng biến nghịch biến của y = sin2x trên x ∈ (0; $^{\frac{\text{π}}{2}}$)

Tìm đồng biến nghịch biến của y = cos2x trên x ∈ (-$\frac{\text{π}}{4}$; $\frac{\text{π}}{4}$)

#Toán lớp 11

Nguyễn Việt Lâm

Giáo viên

21 tháng 9 2019

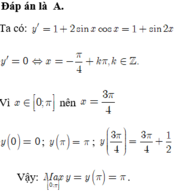
a/ $y'=2cos2x=0\Rightarrow cos2x=0\Rightarrow x=\frac{\pi}{4}+\frac{k\pi}{2}$

Do $x\in\left[0;\frac{\pi}{2}\right]\Rightarrow x=\frac{\pi}{4}$

$cos2x< 0$ khi $\frac{\pi}{4}< x< \frac{\pi}{2}$; $cos2x>0$ khi $0< x< \frac{\pi}{4}$

Hàm số đồng biến trên $\left(0;\frac{\pi}{4}\right)$ nghịch biến trên $\left(\frac{\pi}{4};\frac{\pi}{2}\right)$

b/ $y'=-2sin2x=0\Rightarrow sin2x=0\Rightarrow x=\frac{k\pi}{2}$

Do $x\in\left(-\frac{\pi}{4};\frac{\pi}{4}\right)\Rightarrow x=0$

Hàm số đồng biến trên $\left(-\frac{\pi}{4};0\right)$ nghịch biến trên $\left(0;\frac{\pi}{4}\right)$

Đúng(0)

ly kim

17 tháng 6 2016

$1+\sqrt{2}Sin\left(X+\frac{Π}{4}\right)+sin2x+cos2x=0$

#Toán lớp 11

Nguyễn Thị Anh

17 tháng 6 2016

phương trình tương đương:

sin²x+cos²x+$\sqrt{2}$sin(x+$\frac{\pi}{4}$)+2sinx.cosx+cos²x-sin²x=0

<=> 2cos²x+2sinx.cosx+$\sqrt{2}$sin(x+$\frac{\pi}{4}$)=0

<=> 2cosx(sinx+cosx)+$\sqrt{2}$sin(x+$\frac{\pi}{4}$)=0

<=>(2cosx+1).$\sqrt{2}$sin(x+$\frac{\pi}{4}$)=0

<=>$\left[\begin{array}{nghiempt}sin\left(x+\frac{\pi}{4}\right)=0\\2cosx+1=0\end{array}\right.$

<=> $\left[\begin{array}{nghiempt}x=k\pi-\frac{\pi}{4}\\x=\pm\frac{1}{2}+k2\pi\end{array}\right.$với k$\in$Z

pt có 2 nghiệm như trên

Đúng(0)

Lê Huy Hoàng

24 tháng 7 2020

giải các phương trình sau

a/ $^{tan^2x-\frac{4}{cotx}+=0}$

b/$cos2\left(x+\frac{\text{π}}{3}\right)+4cos\left(\frac{\text{π}}{6}-x\right)=\frac{5}{2}$

c/$\frac{1}{cos^2x}-1+tanx-\sqrt{3}\left(tanx+1\right)=0$

d/tanx-2cotx+1=0

Mọi người ơi giúp mình với <3 cảm ơn mọi người nhìu ạ

#Toán lớp 11

Akai Haruma

Giáo viên

24 tháng 7 2020

Lê Huy Hoàng:

a) ĐK: $x\in\mathbb{R}\setminus \left\{k\pi\right\}$ với $k$ nguyên

PT $\Leftrightarrow \tan ^2x-4\tan x+5=0$

$\Leftrightarrow (\tan x-2)^2+1=0$

$\Leftrightarrow (\tan x-2)^2=-1< 0$ (vô lý)

Do đó pt vô nghiệm.

Đúng(0)

Akai Haruma

Giáo viên

24 tháng 7 2020

ĐK:.............

PT $\Leftrightarrow 1+\frac{\sin ^2x}{\cos ^2x}-1+\tan x-\sqrt{3}(\tan x+1)=0$

$\Leftrightarrow \tan ^2x+\tan x-\sqrt{3}(\tan x+1)=0$

$\Leftrightarrow \tan ^2x+(1-\sqrt{3})\tan x-\sqrt{3}=0$

$\Rightarrow \tan x=\sqrt{3}$ hoặc $\tan x=-1$

$\Rightarrow x=\pi (k-\frac{1}{4})$ hoặc $x=\pi (k+\frac{1}{3})$ với $k$ nguyên

ĐK:.......

PT $\Leftrightarrow \tan x-\frac{2}{\tan x}+1=0$

$\Leftrightarrow \tan ^2x+\tan x-2=0$

$\Leftrightarrow (\tan x-1)(\tan x+2)=0$

$\Rightarrow \tan x=1$ hoặc $\tan x=-2$

$\Rightarrow x=k\pi +\frac{\pi}{4}$ hoặc $x=k\pi +\tan ^{-2}(-2)$ với $k$ nguyên.

Đúng(0)

Bình Trần Thị

30 tháng 8 2016

cho hàm số y = f(x) = 2sin2x .a) chứng minh rằng với số nguyên k tùy ý , luôn có f(x + kπ) = f(x) với mọi x .b) lập bảng biến thiên của hàm số y = 2sin2x trên đoạn $\left[-\frac{\pi}{2};\frac{\pi}{2}\right]$ c) vẽ đồ thị của hàm số y = 2sin2x...

Đọc tiếp

#Toán lớp 11

ly kim

17 tháng 6 2016

$\frac{cos^2X-2cos\left(X+\frac{3Π}{4}\right)Sin\left(3x-\frac{Π}{4}\right)-2}{2cosx-\sqrt{2}}=0$

#Toán lớp 11

Nguyễn Thị Anh

17 tháng 6 2016

điều kiện : cosx$\ne$$\frac{1}{\sqrt{2}}$=> x$\ne$$\pm$$\frac{\pi}{4}$+2k$\pi$, k$\in$Z

pt<=> tử số =0

<=>cos²x-sin(3x-$\frac{\pi}{4}$+x+$\frac{3\pi}{4}$)-sin(3x-$\frac{\pi}{4}$-x-$\frac{3\pi}{4}$)-2=0

<=> cos²x-sin(x+$\frac{\pi}{2}$)-sin(2x-$\pi$)-2=0

<=> cos²x-cosx+sin2x-2sin²x-2cos²x=0

<=>-cos²x-coxs+2sinx.cosx-2sin²x=0

đến đây bạn nhóm lại ra nghiệm rồi kiểm tra đk là xong

Đúng(0)

Bình Trần Thị

4 tháng 9 2016

cho hàm số y = f(x) = $2\sin2x$ .a) lập bảng biến thiên của hàm số y =$2\sin2x$ trên đoạn [−π2;π2][−π2;π2]b) vẽ đồ thị của hàm số y = $2\sin2x$ ...

Đọc tiếp

#Toán lớp 11

Chúng tôi đề xuất

Tài nguyên

Ứng dụng mobile

Bảng xếp hạng

Các khóa học có thể bạn quan tâm

Yêu cầu VIP