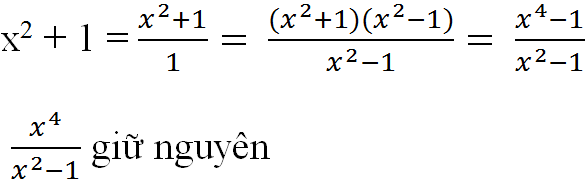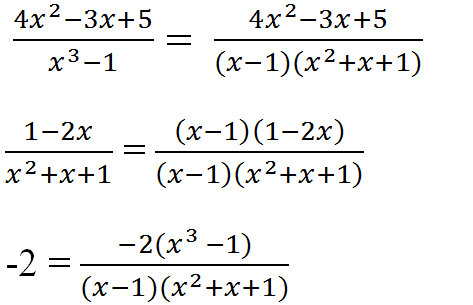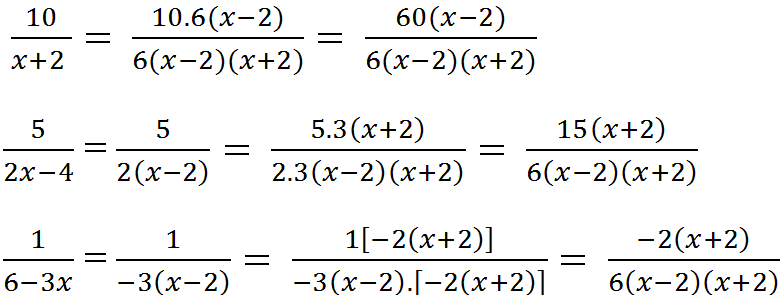Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) \(\dfrac{3x}{2x+4}\) và \(\dfrac{x+3}{x^2-4}\)
Phân tích các mẫu thức thành nhân tử :
\(2x+4 = 2(x+2)\)
\(x^2 - 4 = (x-2)(x+2)\)
MTC : \(2(x+2)(x-2)\)
Nhân tử phụ của mẫu thức : \(2x + 4\) là \((x - 2)\)
\(x^2 - 4\) là \(2\)
QĐ: \(\dfrac{3x}{2x+4}=\dfrac{3x}{2\left(x+2\right)}=\dfrac{3x\left(x-2\right)}{2\left(x+2\right)\left(x-2\right)}\)
\(\dfrac{x+3}{x^2-4}=\dfrac{x+3}{\left(x+2\right)\left(x-2\right)}=\dfrac{2\left(x+3\right)}{2\left(x+2\right)\left(x-2\right)}\)
b) \(\dfrac{x+5}{x^2+4x+4}\) và \(\dfrac{x}{3x+6}\)
Phân tích các mẫu thức thành nhân tử :
\(x^2+4x+4 = (x+2)^2\)
\(3x + 6\) \(= 3(x+2)\)
MTC : \(3(x+2)^2\)
Nhân tử phụ của mẫu thức : \(x^2 + 4x +4 \) là \(3\)
\(3x + 6\) là \((x+2)\)
QĐ : \(\dfrac{x+5}{x^2+4x+4}=\dfrac{\left(x+5\right)}{\left(x+2\right)^2}=\dfrac{3\left(x+5\right)}{3\left(x+2\right)^2}\)
\(\dfrac{x}{3x+6}=\dfrac{x}{3\left(x+2\right)}=\dfrac{x\left(x+2\right)}{3\left(x+2\right)^2}\)

Bài giải
a) \(\dfrac{1}{x+2}=\dfrac{x.\left(x-2\right)}{\left(x+2\right)\left(x-2\right).x}=\dfrac{x^2-2x}{x\left(x+2\right)\left(x-2\right)}\)
\(\dfrac{8}{2x-x^2}=\dfrac{8}{x\left(2-x\right)}=-\dfrac{8}{x\left(x-2\right)}=-\dfrac{8.\left(x+2\right)}{x\left(x-2\right)\left(x+2\right)}\)
b) \(x^2+1=\dfrac{x^2+1}{1}=\dfrac{\left(x^2+1\right)\left(x^2-1\right)}{x^2-1}=\dfrac{x^4-1}{x^2-1}\)
\(\dfrac{x^4}{x^2-1}\) giữ nguyên.
c) \(\dfrac{x^3}{x^3-3x^2y+3xy^2-y^3}=\dfrac{x^3}{\left(x-y\right)^3}=\dfrac{x^3.y}{\left(x-y\right)^3.y}=\dfrac{x^3y}{y\left(x-y\right)^3}\)
\(\dfrac{x}{y^2-xy}=\dfrac{x}{y.\left(y-x\right)}=-\dfrac{x}{y.\left(x-y\right)}=-\dfrac{x\left(x-y\right)^2}{y.\left(x-y\right).\left(x-y\right)^2}=\dfrac{x\left(x-y\right)^2}{y.\left(x-y\right)^3}\)

a) Tìm MTC: x3 – 1 = (x – 1)(x2 + x + 1)
Nên MTC = (x – 1)(x2 + x + 1)
Nhân tử phụ:
(x3 – 1) : (x3 – 1) = 1
(x – 1)(x2 + x + 1) : (x2 + x + 1) = x – 1
(x – 1)(x2+ x + 1) : 1 = (x – 1)(x2 + x + 1)
Qui đồng:
b) Tìm MTC: x + 2
2x – 4 = 2(x – 2)
6 – 3x = 3(2 – x)
MTC = 6(x – 2)(x + 2)
Nhân tử phụ:
6(x – 2)(x + 2) : (x + 2) = 6(x – 2)
6(x – 2)(x + 2) : 2(x – 2) = 3(x + 2)
6(x – 2)(x + 2) : -3(x – 2) = -2(x + 2)
Qui đồng:

a: \(\dfrac{1}{x+2}=\dfrac{2x-x^2}{x\left(2-x\right)\left(2+x\right)}\)
\(\dfrac{8}{2x-x^2}=\dfrac{8}{x\left(2-x\right)}=\dfrac{8x+16}{x\left(2-x\right)\left(2+x\right)}\)
b: \(x^2+1=\dfrac{x^4-1}{x^2-1}\)
\(\dfrac{x^4}{x^2-1}=\dfrac{x^4}{x^2-1}\)
c: \(\dfrac{x^3}{x^3-3x^2y+3xy^2-y^3}=\dfrac{x^3}{\left(x-y\right)^3}=\dfrac{x^3y}{y\cdot\left(x-y\right)^3}\)
\(\dfrac{x}{y^2-xy}=\dfrac{x}{y\left(y-x\right)}=\dfrac{-x}{y\left(x-y\right)}=\dfrac{-x\left(x-y\right)^2}{y\left(x-y\right)^3}\)

a) \(\frac{3x+6}{x^2-4}=\frac{3\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}=\frac{3}{x-2}\)( ĐKXĐ : x ≠ ±2 )
\(\frac{2x+6}{x^3+3x^2-9x-27}=\frac{2\left(x+3\right)}{x^2\left(x+3\right)-9\left(x+3\right)}=\frac{2\left(x+3\right)}{\left(x+3\right)\left(x^2-9\right)}=\frac{2}{\left(x-3\right)\left(x+3\right)}\)( ĐKXĐ : x ≠ ±3 )
MTC : ( x - 2 )( x - 3 )( x + 3 )
=> \(\hept{\begin{cases}\frac{3}{x-2}=\frac{3\left(x-3\right)\left(x+3\right)}{\left(x-2\right)\left(x-3\right)\left(x+3\right)}=\frac{3\left(x^2-9\right)}{\left(x-2\right)\left(x-3\right)\left(x+3\right)}=\frac{3x-27}{\left(x-2\right)\left(x-3\right)\left(x+3\right)}\\\frac{2}{\left(x-3\right)\left(x+3\right)}=\frac{2\left(x-2\right)}{\left(x-2\right)\left(x-3\right)\left(x+3\right)}=\frac{4x-4}{\left(x-2\right)\left(x-3\right)\left(x+3\right)}\end{cases}}\)
b) \(\frac{x^2-4x+4}{2x^2-3x+1}=\frac{\left(x-2\right)^2}{2x^2-2x-x+1}=\frac{\left(x-2\right)^2}{2x\left(x-1\right)-\left(x-1\right)}=\frac{\left(x-2\right)^2}{\left(x-1\right)\left(2x-1\right)}\)( ĐKXĐ : \(\hept{\begin{cases}x\ne1\\x\ne\frac{1}{2}\end{cases}}\))
\(\frac{x+4}{2x-2}=\frac{x+4}{2\left(x-1\right)}\)( ĐKXĐ : x ≠ 1 )
MTC : \(2\left(x-1\right)\left(2x-1\right)\)
=> \(\hept{\begin{cases}\frac{\left(x-2\right)^2}{\left(x-1\right)\left(2x-1\right)}=\frac{2\left(x^2-4x+4\right)}{2\left(x-1\right)\left(2x-1\right)}=\frac{2x^2-8x+8}{2\left(x-1\right)\left(2x-1\right)}\\\frac{x+4}{2\left(x-1\right)}=\frac{\left(x+4\right)\left(2x-1\right)}{2\left(x-1\right)\left(2x-1\right)}=\frac{2x^2+7x-4}{2\left(x-1\right)\left(2x-1\right)}\end{cases}}\)
c) \(\frac{6a}{a-b}\)( ĐKXĐ : a ≠ b ) ; \(\frac{2b}{b-a}=\frac{-2b}{a-b}\)( ĐKXĐ : a ≠ b) ; \(\frac{5}{a^2-b^2}=\frac{5}{\left(a-b\right)\left(a+b\right)}\)( ĐKXĐ : a ≠ ±b )
MTC : \(\left(a-b\right)\left(a+b\right)\)
=> \(\frac{6a}{a-b}=\frac{6a\left(a+b\right)}{\left(a-b\right)\left(a+b\right)}=\frac{6a^2+6ab}{\left(a-b\right)\left(a+b\right)}\)
\(\frac{-2b}{a-b}=\frac{-2b\left(a+b\right)}{\left(a-b\right)\left(a+b\right)}=\frac{-2ab-2b^2}{\left(a-b\right)\left(a+b\right)}\)
\(\frac{5}{a^2-b^2}=\frac{5}{\left(a-b\right)\left(a+b\right)}\)
d) \(\frac{x}{x^2+11x+30}=\frac{x}{x^2+5x+6x+30}=\frac{x}{x\left(x+5\right)+6\left(x+5\right)}=\frac{x}{\left(x+5\right)\left(x+6\right)}\)( ĐKXĐ : x ≠ -5 ; x ≠ -6 )
\(\frac{5}{x^2+9x+20}=\frac{5}{x^2+4x+5x+20}=\frac{5}{x\left(x+4\right)+5\left(x+4\right)}=\frac{5}{\left(x+4\right)\left(x+5\right)}\)( ĐKXĐ : x ≠ -4 ; x ≠ -5 )
MTC : \(\left(x+4\right)\left(x+5\right)\left(x+6\right)\)
=> \(\hept{\begin{cases}\frac{x}{\left(x+5\right)\left(x+6\right)}=\frac{x\left(x+4\right)}{\left(x+4\right)\left(x+5\right)\left(x+6\right)}=\frac{x^2+4x}{\left(x+4\right)\left(x+5\right)\left(x+6\right)}\\\frac{5}{\left(x+4\right)\left(x+5\right)}=\frac{5\left(x+6\right)}{\left(x+4\right)\left(x+5\right)\left(x+6\right)}=\frac{5x+30}{\left(x+4\right)\left(x+5\right)\left(x+6\right)}\end{cases}}\)
Sai chỗ nào bạn bỏ qua nhé