Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Bài giải:
a) x2 – 3x + 2 = a) x2 – x - 2x + 2 = x(x - 1) - 2(x - 1) = (x - 1)(x - 2)
Hoặc x2 – 3x + 2 = x2 – 3x - 4 + 6
= x2 - 4 - 3x + 6
= (x - 2)(x + 2) - 3(x -2)
= (x - 2)(x + 2 - 3) = (x - 2)(x - 1)
b) x2 + x – 6 = x2 + 3x - 2x – 6
= x(x + 3) - 2(x + 3)
= (x + 3)(x - 2).
c) x2 + 5x + 6 = x2 + 2x + 3x + 6
= x(x + 2) + 3(x + 2)
= (x + 2)(x + 3)
a) x2 – 4x + 3 = x2 – x - 3x + 3
= x(x - 1) - 3(x - 1) = (x -1)(x - 3)
b) x2 + 5x + 4 = x2 + 4x + x + 4
= x(x + 4) + (x + 4)
= (x + 4)(x + 1)
c) x2 – x – 6 = x2 +2x – 3x – 6
= x(x + 2) - 3(x + 2)
= (x + 2)(x - 3)
d) x4+ 4 = x4 + 4x2 + 4 – 4x2
= (x2 + 2)2 – (2x)2
= (x2 + 2 – 2x)(x2 + 2 + 2x)
Bài giải:
a) x2 – 4x + 3 = x2 – x - 3x + 3
= x(x - 1) - 3(x - 1) = (x -1)(x - 3)
b) x2 + 5x + 4 = x2 + 4x + x + 4
= x(x + 4) + (x + 4)
= (x + 4)(x + 1)
c) x2 – x – 6 = x2 +2x – 3x – 6
= x(x + 2) - 3(x + 2)
= (x + 2)(x - 3)
d) x4+ 4 = x4 + 4x2 + 4 – 4x2
= (x2 + 2)2 – (2x)2
= (x2 + 2 – 2x)(x2 + 2 + 2x)
\(x^2-\text{5}xy-14y^2\)
\(=x^2+2xy-7xy-14y^2\)
\(=x\left(x+2y\right)-7y\left(x+2y\right)\)
\(=\left(x+2y\right)\left(x-7y\right)\)
a) \(x^2-5xy-14y^2=x^2-7xy+2xy-14y^2\)
\(=\left(x-7y\right)\left(x+2y\right)\)
b) \(x^2-5x+6=x^2-2x-3x+6=\left(x-2\right)\left(x-3\right)\)
c) \(x^4+4=x^4+4x^2+4-\left(2x\right)^2\)
\(=\left(x^2+2\right)^2-\left(2x\right)^2\)
\(=\left(x^2-2x+2\right)\left(x^2+2x+2\right)\)
d)
Bài giải:
a) x2 – xy + x – y = (x2 – xy) + (x - y)
= x(x - y) + (x -y)
= (x - y)(x + 1)
b) xz + yz – 5(x + y) = z(x + y) - 5(x + y)
= (x + y)(z - 5)
c) 3x2 – 3xy – 5x + 5y = (3x2 – 3xy) - (5x - 5y)
= 3x(x - y) -5(x - y) = (x - y)(3x - 5).
\(a) x^2 - xy+x-y\) \(= (x^2 - xy) + ( x- y) \)
\(=x(x-y) + (x-y)\)
\(= (x-y) (x+1)\)
\(b) xz + yz - 5(x+y)\) \(= (xz + yz) - 5(x+y)\)
\(= z(x+y) - 5(x+y)\)
\(= (x+y) (z-5)\)
\(c) 3x^2 - 3xy - 5x +5y = (3x^2-3xy) - (5x-5y)\)
\(= 3x(x-y) - 5(x-y)\)
\(= (x-y)(3x-5)\)
\(g,x^2-2xy+y^2-9z^2=\left(x-y\right)^2-\left(3z\right)^2\)\(=\left(x-y+3z\right)\left(x-y-3z\right)\)
\(h,5x^4-20x^2=5x^2\left(x^2-4\right)=5x^2\left(x-2\right)\left(x+2\right)\)
\(i,7x^2-7y^2-14x+14y=7\left(x-y\right)\left(x+y\right)-14\left(x-y\right)\)
\(=\left(x-y\right)\left(7x+7y-14\right)=7\left(x-y\right)\left(x+y-2\right)\)
\(k,x^2+8x+3x+24=x\left(x+8\right)+3\left(x+8\right)=\left(x+8\right)\left(x+3\right)\)
\(m,x^4-y^4=\left(x^2-y^2\right)\left(x^2+y^2\right)=\left(x-y\right)\left(x+y\right)\left(x^2+y^2\right)\)
\(n,x^6-y^6=\left(x^2-y^2\right)\left(x^4+x^2y^2+y^4\right)=\left(x-y\right)\left(x+y\right)\left(x^4+x^2y^2+y^4\right)\)
a) x2 – 3x + 2 = a) x2 – x - 2x + 2 = x(x - 1) - 2(x - 1) = (x - 1)(x - 2)
Hoặc x2 – 3x + 2 = x2 – 3x - 4 + 6
= x2 - 4 - 3x + 6
= (x - 2)(x + 2) - 3(x -2)
= (x - 2)(x + 2 - 3) = (x - 2)(x - 1)
b) x2 + x – 6 = x2 + 3x - 2x – 6
= x(x + 3) - 2(x + 3)
= (x + 3)(x - 2).
\(x^3+5x^2+3x-9\)
\(=x^3-x^2+6x^2-6x+9x-9\)
\(=x^2\left(x-1\right)+6x\left(x-1\right)+9\left(x-1\right)\)
\(=\left(x-1\right)\left(x^2+6x+9\right)=\left(x-1\right)\left(x+3\right)^2\)
\(x^{16}+x^8-2\)
\(=\left(x^{16}-1\right)+\left(x^8-1\right)\)
\(=\left(x^8-1\right)\left(x^8+1\right)+\left(x^8-1\right)\)
\(=\left(x^8-1\right)\left(x^8+2\right)\)
\(=\left(x^4-1\right)\left(x^4+1\right)\left(x^8+2\right)\)
\(=\left(x-1\right)\left(x+1\right)\left(x^2+1\right)\left(x^4+1\right)\left(x^8+2\right)\)
\(c,x^3+5x^2+3x-9\)
\(=x^3+6x^2+9-x^2-6x-9\)
\(=x\left(x^2+6x^2+9\right)-\left(x^2+6x^2+9\right)\)
\(=x.\left(x+3\right)^2-\left(x+3\right)^2\)
\(=\left(x+3\right)^2\left(x-1\right)\)
\(d,x^{16}+x^8-2\)
\(=\left(x^8+2x^4+1\right)-x^4\)
\(=\left(x^4+1\right)^2-x^4\)
\(=\left(x^4+1+x^4\right)\left(x^4+1-x^4\right)\)
a) \(3x^2-9x+30=3\left(x^2-3x+10\right)\)
b) \(3x^2-5x-2=3x^2-6x+x-2\)
\(=3x\left(x-2\right)+\left(x-2\right)=\left(3x+1\right)\left(x-2\right)\)
c) \(x^4+4y^4\)
\(=x^4+4y^4+2x^2y^2+2x^2y^2-4x^2y^2+4xy^3-4xy^3+2x^3y-2x^3y\)
\(=\left(4y^4-4xy^3+2x^2y^2\right)+\left(4xy^3-4x^2y^2+2x^3y\right)\)
\(+\left(2x^2y^2-2x^3y+x^4\right)\)
\(=2y^2\left(2y^2-2xy+x^2\right)+2xy\left(2y^2-2xy+x^2\right)\)
\(+x^2\left(2y^2-2xy+x^2\right)\)
\(=\left(2y^2+2xy+x^2\right)\left(2y^2-2xy+x^2\right)\)
d) \(x^5+x+1\)
\(=x^5+x+1+x^4-x^4+x^3-x^3+x^2-x^2\)
\(=\left(x^5-x^4+x^2\right)+\left(x^4-x^3+x\right)+\left(x^3-x^2+1\right)\)
\(=x^2\left(x^3-x^2+1\right)+x\left(x^3-x^2+1\right)+\left(x^3-x^2+1\right)\)
\(=\left(x^2+x+1\right)\left(x^3-x^2+1\right)\)
Cách 1: Tách một hạng tử thành tổng hai hạng tử để xuất hiện nhân tử chung.
a) x2 – 3x + 2
= x2 – x – 2x + 2 (Tách –3x = – x – 2x)
= (x2 – x) – (2x – 2)
= x(x – 1) – 2(x – 1) (Có x – 1 là nhân tử chung)
= (x – 1)(x – 2)
Hoặc: x2 – 3x + 2
= x2 – 3x – 4 + 6 (Tách 2 = – 4 + 6)
= x2 – 4 – 3x + 6
= (x2 – 22) – 3(x – 2)
= (x – 2)(x + 2) – 3.(x – 2) (Xuất hiện nhân tử chung x – 2)
= (x – 2)(x + 2 – 3) = (x – 2)(x – 1)
b) x2 + x – 6
= x2 + 3x – 2x – 6 (Tách x = 3x – 2x)
= x(x + 3) – 2(x + 3) (có x + 3 là nhân tử chung)
= (x + 3)(x – 2)
c) x2 + 5x + 6 (Tách 5x = 2x + 3x)
= x2 + 2x + 3x + 6
= x(x + 2) + 3(x + 2) (Có x + 2 là nhân tử chung)
= (x + 2)(x + 3)
Cách 2: Đưa về hằng đẳng thức (1) hoặc (2)
a) x2 – 3x + 2
(Vì có x2 và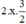
nên ta thêm bớt 
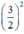để xuất hiện HĐT)
= (x – 2)(x – 1)
b) x2 + x - 6
= (x – 2)(x + 3).
c) x2 + 5x + 6
= (x + 2)(x + 3).