
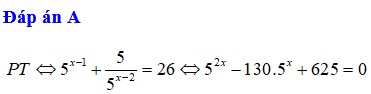
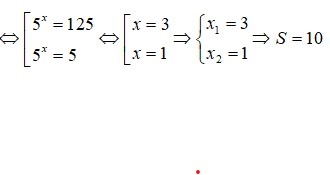
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

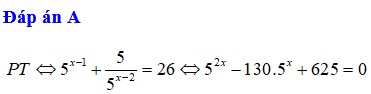
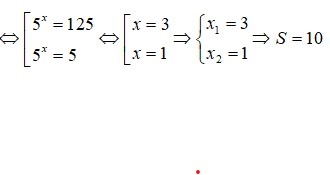

a) -2x+14=0
<=>-2x= - 14
<=>x = 7
Vậy phương trình có tập nghiệm x={7}
b)(4x-10) (x+5)=0
<=>4x-10=0 <=>4x=10 <=>x=5/2
<=>x+5=0 <=>x=-5
Vậy phương trình có tập nghiệm x={5/2;- 5}
c)\(\frac{1-x}{x+1}\) + 3=\(\frac{2x+3}{x+1}\)
ĐKXD: x+1 #0<=>x#-1(# là khác)
\(\frac{1-x}{x+1}\)+3=\(\frac{2x+3}{x+1}\)
<=>\(\frac{1-x}{x+1}\)+\(\frac{3.\left(x+1\right)}{x+1}\)=\(\frac{2x+3}{x+1}\)
<=>\(\frac{1-x}{x+1}\)+\(\frac{3x+3}{x+1}\)=\(\frac{2x+3}{x+1}\)
=>1-x+3x+3=2x+3
<=>-x+3x-2x=-1-3+3
<=>0x = -1 (vô nghiệm)
Vâyj phương trình vô nghiệm
d) 1,2-(x-0,8)=-2(0,9+x)
<=> 1,2-x+0,8=-1,8-2x
<=>-x+2x=-1,2-0,8-1,8
<=>x=-4
Vậy phương trình có tập nghiệm x={-4}

a)\(\Delta'=\left[\frac{-2.\left(m-1\right)}{2}\right]^2-m^2=m^2-2m+1-m^2=-2m+1\)
b)Để PT có hai nghiệm phân biệt thì \(\Delta'=-2m+1>0\Rightarrow m<\frac{1}{2}\)
Để PT có nghiệm kép thì: \(\Delta'=-2m+1=0\Rightarrow m=\frac{1}{2}\)
Để PT vô nghiệm thì: \(\Delta'=-2m+1<0\Rightarrow m>\frac{1}{2}\)

a) \(4x-7>0\Leftrightarrow4x>7\)\(\Leftrightarrow x>\frac{7}{4}\)
b) \(-5x+8>0\Leftrightarrow5x<8\Leftrightarrow x<\frac{8}{5}\)
c)\(9x-10\le0\Leftrightarrow9x\le10\)\(\Leftrightarrow x\le\frac{10}{9}\)
d) \(\left(x+1\right)^2+4\le x^2+3x+10\)\(\Leftrightarrow x^2-2x+1+4\le x^2+3x+10\)
\(\Leftrightarrow5x\ge-5\Leftrightarrow x\ge-1\)
a,
4x - 7 > 0
↔ 4x > 7
↔ x > \(\dfrac{7}{4}\)
Vậy tập nghiệm của bất phương trình là S = { x / x>\(\dfrac{7}{4}\) }
b,
-5x + 8 > 0
↔ 8 > 5x
↔ \(\dfrac{8}{5}\) > x
Vậy tập nghiệm của bất phương trình là S = { x / \(\dfrac{8}{5}\) > x }
c,
9x - 10 ≤ 0
↔ 9x ≤ 10
↔ x ≤ \(\dfrac{10}{9}\)
Vậy tập nghiệm của bất phương trình là S = { x / x ≤ \(\dfrac{10}{9}\) }
d,
( x - 1 )\(^2\) + 4 ≤ x\(^2\) + 3x + 10
↔ x\(^2\) - 2x +1 +4 ≤ x\(^2\) + 3x + 10
↔ 1 + 4 - 10 ≤ x \(^2\) - x\(^2\) + 3x + 2x
↔ -5 ≤ 5x
↔ -1 ≤ x
Vậy tập nghiệm của bất phương trình là S = { x / -1 ≤ x}

a) ĐK: x-1 khác 0 và x+1 khác 0
<=> x khác 1 và x khác -1
b) ĐK: x-2 khác 0
<=> x khác 2

Bài 1:
\(\Delta=\left(-3\right)^2-4\left(m-1\right)=-4m+4+9=-4m+13\)
Để phương trình có hai nghiệm phân biệt thì -4m+13>0
=>-4m>-13
hay m<13/4
Áp dụng hệ thức Vi-et, ta được:
\(\left\{{}\begin{matrix}x_1+x_2=3\\x_1x_2=m-1\end{matrix}\right.\)
Theo đề, ta có hệ phương trình:
\(\left\{{}\begin{matrix}x_1+x_2=3\\2x_1-5x_2=-8\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x_1+2x_2=6\\2x_1-5x_2=-8\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}7x_2=14\\x_1+x_2=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_2=2\\x_1=1\end{matrix}\right.\)
Ta có: \(x_1\cdot x_2=m-1\)
nên m-1=2
hay m=3
Bài 2:
\(\Delta=\left(2m-4\right)^2-4\cdot\left(-2m+1\right)\)
\(=4m^2-16m+16+8m-4\)
\(=4m^2-8m+12\)
\(=4m^2-8m+4+8=\left(2m-2\right)^2+8>0\)
Do đó: Phương trình luôn có hai nghiệm phân biệt
Để phương trình có hai nghiệm dương thì \(\left\{{}\begin{matrix}-2\left(m-2\right)>0\\-2m+1>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m< 2\\m< \dfrac{1}{2}\end{matrix}\right.\Leftrightarrow m< \dfrac{1}{2}\)

Bài 1:
\(\Delta=\left(-3\right)^2-4\left(m-1\right)=-4m+4+9=-4m+13\)
Để phương trình có hai nghiệm phân biệt thì -4m+13>0
=>-4m>-13
hay m<13/4
Áp dụng hệ thức Vi-et, ta được:
\(\left\{{}\begin{matrix}x_1+x_2=3\\x_1x_2=m-1\end{matrix}\right.\)
Theo đề, ta có hệ phương trình:
\(\left\{{}\begin{matrix}x_1+x_2=3\\2x_1-5x_2=-8\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x_1+2x_2=6\\2x_1-5x_2=-8\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}7x_2=14\\x_1+x_2=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_2=2\\x_1=1\end{matrix}\right.\)
Ta có: \(x_1\cdot x_2=m-1\)
nên m-1=2
hay m=3
Bài 2:
\(\Delta=\left(2m-4\right)^2-4\cdot\left(-2m+1\right)\)
\(=4m^2-16m+16+8m-4\)
\(=4m^2-8m+12\)
\(=4m^2-8m+4+8=\left(2m-2\right)^2+8>0\)
Do đó: Phương trình luôn có hai nghiệm phân biệt
Để phương trình có hai nghiệm dương thì \(\left\{{}\begin{matrix}-2\left(m-2\right)>0\\-2m+1>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m< 2\\m< \dfrac{1}{2}\end{matrix}\right.\Leftrightarrow m< \dfrac{1}{2}\)