
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

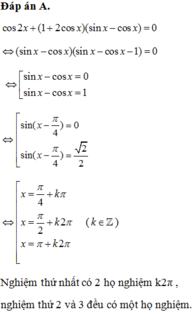

a.
\(\Leftrightarrow sin2x+cos2x=3sinx+cosx+2\)
\(\Leftrightarrow2sinx.cosx-3sinx+2cos^2x-cosx-3=0=0\)
\(\Leftrightarrow sinx\left(2cosx-3\right)+\left(cosx+1\right)\left(2cosx-3\right)=0\)
\(\Leftrightarrow\left(sinx+cosx+1\right)\left(2cosx-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx+cosx=-1\\2cosx-3=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}sin\left(x+\frac{\pi}{4}\right)=-\frac{\sqrt{2}}{2}\\cosx=\frac{3}{2}\left(vn\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\frac{\pi}{4}=-\frac{\pi}{4}+k2\pi\\x+\frac{\pi}{4}=\frac{5\pi}{4}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow...\)
b.
\(\Leftrightarrow1+sinx+cosx+2sinx.cosx+2cos^2x-1=0\)
\(\Leftrightarrow sinx\left(2cosx+1\right)+cosx\left(2cosx+1\right)=0\)
\(\Leftrightarrow\left(sinx+cosx\right)\left(2cosx+1\right)=0\)
\(\Leftrightarrow\sqrt{2}sin\left(x+\frac{\pi}{4}\right)\left(2cosx+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sin\left(x+\frac{\pi}{4}\right)=0\\cosx=-\frac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\frac{\pi}{4}+k\pi\\x=\frac{2\pi}{3}+k2\pi\\x=-\frac{2\pi}{3}+k2\pi\end{matrix}\right.\)

1.
\(\Leftrightarrow sin^2x\left(sinx+1\right)-2\left(1-cosx\right)=0\)
\(\Leftrightarrow\left(1-cos^2x\right)\left(sinx+1\right)-2\left(1-cosx\right)=0\)
\(\Leftrightarrow\left(1-cosx\right)\left(1+cosx\right)\left(sinx+1\right)-2\left(1-cosx\right)=0\)
\(\Leftrightarrow\left(1-cosx\right)\left(sinx+cosx+sinx.cosx-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=1\Leftrightarrow...\\sinx+cosx+sinx.cosx-1=0\left(1\right)\end{matrix}\right.\)
Xét (1):
Đặt \(sinx+cosx=t\Rightarrow\left[{}\begin{matrix}\left|t\right|\le\sqrt{2}\\sinx.cosx=\frac{t^2-1}{2}\end{matrix}\right.\)
\(\Leftrightarrow t+\frac{t^2-1}{2}-1=0\)
\(\Leftrightarrow t^2+2t-3=0\Rightarrow\left[{}\begin{matrix}t=1\\t=-3\left(l\right)\end{matrix}\right.\)
\(\Leftrightarrow sin\left(x+\frac{\pi}{4}\right)=\frac{\sqrt{2}}{2}\)
\(\Leftrightarrow...\)
2.
\(\Leftrightarrow\sqrt{3}sinx.cosx+\sqrt{2}cos^2x+\sqrt{6}cosx=0\)
\(\Leftrightarrow cosx\left(\sqrt{3}sinx+\sqrt{2}cosx+\sqrt{6}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\Leftrightarrow...\\\sqrt{3}sinx+\sqrt{2}cosx=-\sqrt{6}\left(1\right)\end{matrix}\right.\)
Xét (1):
Do \(\sqrt{3}^2+\sqrt{2}^2< \left(-\sqrt{6}\right)^2\) nên (1) vô nghiệm

1.
Hàm tuần hoàn với chu kì \(2\pi\) nên ta chỉ cần xét trên đoạn \(\left[0;2\pi\right]\)
\(y'=\frac{-4}{\left(cosx-2\right)^2}.sinx=0\Leftrightarrow x=k\pi\)
\(\Rightarrow x=\left\{0;\pi;2\pi\right\}\)
\(y\left(0\right)=-3\) ; \(y\left(\pi\right)=\frac{1}{3}\) ; \(y\left(2\pi\right)=-3\)
\(\Rightarrow\left\{{}\begin{matrix}M=\frac{1}{3}\\m=-3\end{matrix}\right.\)
\(\Rightarrow9M+m=0\)
2.
\(\Leftrightarrow y.cosx+y.sinx+2y=2k.cosx+k+1\)
\(\Leftrightarrow y.sinx+\left(y-2k\right)cosx=k+1-2y\)
Theo điều kiện có nghiệm của pt lượng giác bậc nhất:
\(\Rightarrow y^2+\left(y-2k\right)^2\ge\left(k+1-2y\right)^2\)
\(\Leftrightarrow2y^2-4k.y+4k^2\ge4y^2-4\left(k+1\right)y+\left(k+1\right)^2\)
\(\Leftrightarrow2y^2-4y-3k^2+2k+1\le0\)
\(\Leftrightarrow2\left(y-1\right)^2\le3k^2-2k+1\)
\(\Leftrightarrow y\le\sqrt{\frac{3k^2-2k+1}{2}}+1\)
\(y_{max}=f\left(k\right)=\frac{1}{\sqrt{2}}\sqrt{3k^2-2k+1}+1\)
\(f\left(k\right)=\frac{1}{\sqrt{2}}\sqrt{3\left(k-\frac{1}{3}\right)^2+\frac{2}{3}}+1\ge\frac{1}{\sqrt{3}}+1\)
Dấu "=" xảy ra khi và chỉ khi \(k=\frac{1}{3}\)
Đáp án A

a.\(\frac{k\Pi}{2}+\frac{\alpha}{2}\)
b.\(\left\{{}\begin{matrix}x=\frac{1}{4}arcsin\left(\frac{1}{3}\right)+\frac{k\Pi}{2}-\frac{1}{8}\\x=\Pi-\frac{1}{4}arcsin\left(\frac{1}{3}\right)+\frac{k\Pi}{2}-\frac{1}{8}\end{matrix}\right.\)

d/ ĐKXĐ: ...
\(\Leftrightarrow\frac{\left(cosx-sinx\right)\left(cos^2x+sin^2x+sinx.cosx\right)}{2cosx+3sinx}=cos^2x-sin^2x\)
\(\Leftrightarrow\frac{\left(cosx-sinx\right)\left(1+sinx.cosx\right)}{2cosx+3sinx}=\left(cosx-sinx\right)\left(cosx+sinx\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx-sinx=0\Leftrightarrow x=\frac{\pi}{4}+k\pi\\\frac{1+sinx.cosx}{2cosx+3sinx}=sinx+cosx\left(1\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow1+sinx.cosx=\left(sinx+cosx\right)\left(2cosx+3sinx\right)\)
\(\Leftrightarrow1+sinx.cosx=2sin^2x+3cos^2x+5sinx.cosx\)
\(\Leftrightarrow2sin^2x+3cos^2x+4sinx.cosx-1=0\)
Nhận thấy \(cosx=0\) ko phải nghiệm, chia 2 vế cho \(cos^2x\)
\(2tan^2x+3+4tanx-1-tan^2x=0\)
\(\Leftrightarrow tan^2x+4tanx+2=0\)
\(\Leftrightarrow tanx=-2\pm\sqrt{2}\)
\(\Rightarrow x=arctan\left(-2\pm\sqrt{2}\right)+k\pi\)
c/
\(\Leftrightarrow\left(sinx-cosx\right)\left(sinx+4cosx\right)=4\left(sinx-cosx\right)\)
\(\Leftrightarrow\left(sinx-cosx\right)\left(sinx+4cosx-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx-cosx=0\left(1\right)\\sinx+4cosx-4=0\left(2\right)\end{matrix}\right.\)
Xét (1) \(\Leftrightarrow sin\left(x-\frac{\pi}{4}\right)=0\Leftrightarrow x=\frac{\pi}{4}+k\pi\)
Xét (2) \(\Leftrightarrow\frac{1}{\sqrt{17}}sinx+\frac{4}{\sqrt{17}}cosx=\frac{4}{\sqrt{17}}\)
Đặt \(\frac{4}{\sqrt{17}}=cosa\) với \(a\in\left(0;\pi\right)\)
\(\Rightarrow cosx.cosa+sinx.sina=cosa\)
\(\Leftrightarrow cos\left(x-a\right)=cosa\)
\(\Leftrightarrow\left[{}\begin{matrix}x-a=a+k2\pi\\x-a=-a+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2a+k2\pi\\x=k2\pi\end{matrix}\right.\)

e/
\(\Leftrightarrow\left(sin^2x+4sinx.cosx+3cos^2x\right)-\left(sinx+3cosx\right)=0\)
\(\Leftrightarrow\left(sinx+cosx\right)\left(sinx+3cosx\right)-\left(sinx+3cosx\right)=0\)
\(\Leftrightarrow\left(sinx+3cosx\right)\left(sinx+cosx-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx+3cosx=0\\sinx+cosx-1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=-3cosx\\\sqrt{2}sin\left(x+\frac{\pi}{4}\right)=1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}tanx=-3\\sin\left(x+\frac{\pi}{4}\right)=\frac{\sqrt{2}}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=arctan\left(-3\right)+k\pi\\x=k2\pi\\x=\frac{\pi}{2}+k2\pi\end{matrix}\right.\)
d/
\(\Leftrightarrow2sinx+2sinx.cos2x-\left(1-sin2x\right)-2cosx=0\)
\(\Leftrightarrow2\left(sinx-cosx\right)+2sinx\left(cos^2x-sin^2x\right)-\left(sinx-cosx\right)^2=0\)
\(\Leftrightarrow2\left(sinx-cosx\right)-2sinx\left(sinx-cosx\right)\left(sinx+cosx\right)-\left(sinx-cosx\right)^2=0\)
\(\Leftrightarrow\left(sinx-cosx\right)\left(2-2sin^2x-2sinx.cosx-sinx+cosx\right)=0\)
\(\Leftrightarrow\left(sinx-cosx\right)\left[2cos^2x-2sinx.cosx-sinx+cosx\right]=0\)
\(\Leftrightarrow\left(sinx-cosx\right)\left[2cosx\left(cosx-sinx\right)+cosx-sinx\right]=0\)
\(\Leftrightarrow-\left(sinx-cosx\right)^2\left(2cosx+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx-cosx=0\\2cosx+1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}sin\left(x-\frac{\pi}{4}\right)=0\\cosx=-\frac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{4}+k\pi\\x=\pm\frac{2\pi}{3}+k2\pi\end{matrix}\right.\)