Cho biểu thức: C = x 3...

Cho biểu thức: C =...

NP

nguyễn phương thùy

8 tháng 5 2019

cho biểu thức P =\(\left(\frac{x-3\sqrt{x}}{x-6\sqrt{x}+9}-\frac{2\sqrt{x}-1}{x-3\sqrt{x}}\right).\frac{x-9}{\sqrt{x}+3}\)

tìm điều kiện xác định và rút gọn biểu thức P

#Toán lớp 9

1

TN

tran nguyen bao quan

8 tháng 5 2019

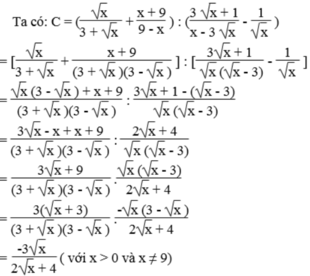
ĐKXĐ: \(x>0\),\(x\ne3\)

\(P=\left(\frac{x-3\sqrt{x}}{x-6\sqrt{x}+9}-\frac{2\sqrt{x}-1}{x-3\sqrt{x}}\right).\frac{x-9}{\sqrt{x}+3}=\left[\frac{\sqrt{x}\left(\sqrt{x}-3\right)}{\left(\sqrt{x}-3\right)^2}-\frac{2\sqrt{x}-1}{\sqrt{x}\left(\sqrt{x}-3\right)}\right].\frac{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}{\sqrt{x}+3}=\left[\frac{x}{\sqrt{x}\left(\sqrt{x}-3\right)}-\frac{2\sqrt{x}-1}{\sqrt{x}\left(\sqrt{x}-3\right)}\right].\left(\sqrt{x}-3\right)=\frac{x-2\sqrt{x}+1}{\sqrt{x}}\)

Đúng(1)

NT

Nguyễn Thị Thanh Thảo

25 tháng 5 2017 - olm

C=\(\left(\frac{\sqrt{x}}{3+\sqrt{x}}+\frac{x+9}{9-x}\right):\left(\frac{3\sqrt{x}+1}{x-3\sqrt{x}}-\frac{1}{\sqrt{x}}\right)\)

a)Rút gọn biểu thức
b) Tìm x sao cho C<-1

#Toán lớp 9

5

CV

Cao Van Minh

25 tháng 5 2017

em ko biết em mới học lớp 1

Đúng(0)

TB

Theu Bui

4 tháng 8 2018

Thế mà cùng nói

Đúng(0)

MT

Minh Thảo

1 tháng 10 2019

Cho biểu thức C=\(\left(\frac{\sqrt{x}}{3+\sqrt{x}}+\frac{x+9}{9-x}\right):\left(\frac{3\sqrt{x}+1}{x-3\sqrt{x}}-\frac{1}{\sqrt{x}}\right)\) với x>0 và x≠9

a)Rút gọn C

b)Tìm x để C<-1

#Toán lớp 9

1

NH

Ngọc Hưng

2 tháng 10 2019

a, C = \(\left(\frac{\sqrt{x}}{3+\sqrt{x}}-\frac{x+9}{9-x}\right):\left(\frac{3\sqrt{x}+1}{x-3\sqrt{x}}-\frac{1}{\sqrt{x}}\right)\)

\(=\left[\frac{\sqrt{x}}{3+\sqrt{x}}-\frac{x+9}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}\right]:\left[\frac{3\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-3\right)}-\frac{1}{\sqrt{x}}\right]\)

\(=\frac{\sqrt{x}\left(3-\sqrt{x}\right)+x+9}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}:\frac{3\sqrt{x}+1-\left(\sqrt{x}-3\right)}{\sqrt{x}\left(\sqrt{x}-3\right)}\)

\(=\frac{3\sqrt{x}-x+x+9}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}:\frac{3\sqrt{x}+1-\sqrt{x}+3}{\sqrt{x}\left(\sqrt{x}-3\right)}\)

\(=\frac{3\sqrt{x}+9}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}:\frac{2\sqrt{x}+4}{\sqrt{x}\left(\sqrt{x}-3\right)}\)

\(=\frac{-3\left(\sqrt{x}+3\right)}{\left(\sqrt{x}-3\right)\left(3+\sqrt{x}\right)}\cdot\frac{\sqrt{x}\left(\sqrt{x}-3\right)}{2\sqrt{x}+4}=\frac{-3\sqrt{x}}{2\sqrt{x}+4}\)

Đúng(0)

TN

Trần Ngọc Thảo

24 tháng 9 2019

Cho biểu thức

a) rút gọn C

b) tìm x sao cho C < -1

C =

\(\left(\frac{\sqrt{x}}{3+\sqrt{x}}+\frac{x+9}{9-x}\right):\left(\frac{3\sqrt{x}+1}{x-3\sqrt{x}}-\frac{1}{\sqrt{x}}\right)\)

#Toán lớp 9

1

NH

Nguyễn Hoàng Nhi

24 tháng 9 2019

Hỏi đáp Toán

Đúng(0)

NL

nagisyaw le

22 tháng 12 2022

sao 3x\(\sqrt{x}\) = -3\(\sqrt{x}\) ạ???

Đúng(0)

P

Phương

20 tháng 10 2018

Cho biểu thức

\(C=\left(\dfrac{\sqrt{x}}{3+\sqrt{x}}+\dfrac{x-9}{9-x}\right):\left(\dfrac{3\sqrt{x}+1}{x-3\sqrt{x}}-\dfrac{1}{\sqrt{x}}\right)\) với \(x\ge0,x\ne9\)

a, Rút gọn C

b,Tìm x sao cho C < -1

#Toán lớp 9

1

NL

Nguyễn Lê Phước Thịnh

26 tháng 10 2022

a:

Sửa đề: \(C=\left(\dfrac{\sqrt{x}}{3+\sqrt{x}}+\dfrac{x+9}{9-x}\right):\left(\dfrac{3\sqrt{x}+1}{x-3\sqrt{x}}-\dfrac{1}{\sqrt{x}}\right)\)

\(C=\dfrac{\sqrt{x}\left(\sqrt{x}-3\right)-x-9}{x-9}:\dfrac{3\sqrt{x}+1-\sqrt{x}+3}{\sqrt{x}\left(\sqrt{x}-3\right)}\)

\(=\dfrac{x-3\sqrt{x}-x-9}{x-9}\cdot\dfrac{\sqrt{x}\left(\sqrt{x}-3\right)}{2\sqrt{x}+4}\)

\(=\dfrac{-3\left(\sqrt{x}+3\right)}{\sqrt{x}+3}\cdot\dfrac{\sqrt{x}}{2\sqrt{x}+4}\)

\(=-\dfrac{3\sqrt{x}}{2\sqrt{x}+4}\)

b: Để C<-1 thì C+1<0

=>-3 căn x+2 căn x+4<0

=>-căn x<-4

=>x>16

Đúng(0)

NT

Nguyễn Thị Thanh Hoà

1 tháng 2 2020

cho biểu thức M = \(\frac{1}{3-\sqrt{x}}+\frac{\sqrt{x}}{3+\sqrt{x}}-\frac{x+9}{x-9}\)

a) tìm điều kiện của x để biểu thức M có nghĩa. Rút gọn biểu thức M

b) tìm các giá trị của x để M > 1

#Toán lớp 9

1

LT

Lê Thị Thục Hiền

1 tháng 2 2020

Đk: \(x\ge0,x\ne3,x\ne9\)

Rút gọn chưa hết nè

(tiếp)\(=\frac{4\left(3+\sqrt{x}\right)}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}=\frac{4}{3-\sqrt{x}}\)

b,Để M>1<=> \(\frac{4}{3-\sqrt{x}}>1\)

<=>\(\frac{4}{3-\sqrt{x}}-1>0\) <=> \(\frac{4-3+\sqrt{x}}{3-\sqrt{x}}>0\) <=>\(\frac{1+\sqrt{x}}{3-\sqrt{x}}>0\) => \(3-\sqrt{x}>0\) (do \(1+\sqrt{x}>0\))

<=>\(\sqrt{x}< 3\) <=> x<9 kết hợp đk của x => \(0\le x< 9,x\ne3\)

Đúng(0)

D

✿✿❑ĐạT̐®ŋɢย❐✿✿

1 tháng 2 2020

a) \(ĐKXĐ:x\ne\pm3,x\ne9\)

Rút gọn :

Ta có : \(M=\frac{1\left(3+\sqrt{x}\right)+\sqrt{x}\left(3-\sqrt{x}\right)+x+9}{9-x}\)

\(=\frac{3+\sqrt{x}+3\sqrt{x}-x+x+9}{9-x}\)

\(=\frac{12+4\sqrt{x}}{9-x}\)

Vậy : ...

P/s : E chưa học căn mới lớp 8, có gì sai sót thì mong chỉ bảo ạ !

Đúng(0)

ML

Mai Linh

9 tháng 7 2019

Cho biểu thức A = \(\frac{\sqrt{4+2\sqrt{3}}}{\sqrt{3}+1}+\frac{5+3\sqrt{5}}{\sqrt{5}}-\left(\sqrt{5}+3\right)\)

B = \(\frac{1}{3-\sqrt{x}}+\frac{\sqrt{x}}{3+\sqrt{x}}-\frac{x+9}{x-9}\) với x ≠ 9, x ≥ 0

a, Rút gọn biểu thức A

b, Tìm các giá trị của x để B > A

#Toán lớp 9

2

VH

Vi Huyên

9 tháng 7 2019

a) \(A=\frac{\sqrt{4+2\sqrt{3}}}{\sqrt{3}+1}+\frac{5+3\sqrt{5}}{\sqrt{5}}-\left(\sqrt{5}+3\right)\)

\(A=\frac{\sqrt{\left(\sqrt{3}+1\right)^2}}{\sqrt{3}+1}+\frac{5+3\sqrt{5}}{\sqrt{5}}-\frac{\sqrt{5}\left(\sqrt{5}+3\right)}{\sqrt{5}}\)

\(A=\frac{\sqrt{3}+1}{\sqrt{3}+1}+\frac{5+3\sqrt{5}}{\sqrt{5}}-\frac{5+3\sqrt{5}}{\sqrt{5}}\)

\(A=1\)

b) Ta có:

\(B=\frac{1}{3-\sqrt{x}}+\frac{\sqrt{x}}{3+\sqrt{x}}-\frac{x+9}{x-9}\) ( x >= 0, x khác 9 )

\(B=\frac{3+\sqrt{x}}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}+\frac{\sqrt{x}\left(3-\sqrt{x}\right)}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}+\frac{x+9}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}\)

\(B=\frac{3+\sqrt{x}+3\sqrt{x}-x+x+9}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}\)

\(B=\frac{3+\sqrt{x}+3\sqrt{x}+9}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}\)

\(B=\frac{\left(3+\sqrt{x}\right)+3\left(\sqrt{x}+3\right)}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}\)

\(B=\frac{4\left(3+\sqrt{x}\right)}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}\)

\(B=\frac{4}{3-\sqrt{x}}\)

Để B > A

\(\Rightarrow\frac{4}{3-\sqrt{x}}>1\)

\(\Rightarrow4>3-\sqrt{x}\)

\(\Rightarrow4-3+\sqrt{x}>0\)

\(\Rightarrow1+\sqrt{x}>0\)

\(\Rightarrow\sqrt{x}>-1\)

\(\Rightarrow x>1\)

Đúng(0)

WE

What ever

9 tháng 7 2019

a) A=\(\frac{\sqrt{4+2\sqrt{3}}}{\sqrt{3}+1}+\frac{5+3\sqrt{5}}{\sqrt{5}}-\left(\sqrt{5}+3\right)\)

\(=\frac{\sqrt{3+2\sqrt{3}+1}}{\sqrt{3}+1}+\frac{\sqrt{5}\cdot\left(\sqrt{5}+3\right)}{\sqrt{5}}\)

\(=\frac{\sqrt{\left(\sqrt{3}+1\right)^2}}{\sqrt{3}+1}+\left(\sqrt{5}+3\right)-\left(\sqrt{5}+3\right)\)

\(=\frac{\sqrt{3}+1}{\sqrt{3}+1}+0=1\)

b) B=\(\frac{1}{3-\sqrt{x}}+\frac{\sqrt{x}}{3+\sqrt{x}}-\frac{x+9}{x-9}\)

\(=\frac{3+\sqrt{x}+\sqrt{x}\left(3-\sqrt{x}\right)}{\left(3-\sqrt{x}\right)\cdot\left(3+\sqrt{x}\right)}+\frac{x+9}{9-x}\)

\(=\frac{3+\sqrt{x}+3\sqrt{x}-x}{\left(3-\sqrt{x}\right)\cdot\left(3+\sqrt{x}\right)}+\frac{x+9}{\left(3-\sqrt{x}\right)\cdot\left(3+\sqrt{x}\right)}\)

\(=\frac{4\text{}\sqrt{x}+12}{\left(3-\sqrt{x}\right)\cdot\left(3+\sqrt{x}\right)}\)

\(=\frac{4\left(\sqrt{x}+3\right)}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}\)

\(=\frac{4}{3-\sqrt{x}}\)

\(B>A \Leftrightarrow\frac{4}{3-\sqrt{x}}>1\)

các giá trị của x là \(\left\{x\in R\backslash0\le x\le9\right\}\)

Đúng(0)

T

trandinhbao

25 tháng 12 2018

A=(\(\dfrac{\sqrt{x}}{\sqrt{x}+3}-\dfrac{x+9}{x-9}\)):(\(\dfrac{3\sqrt{x+1}}{x-3\sqrt{x}}-\dfrac{1}{\sqrt{x}}\)) (với x>0,x khác 9)

a,rút gọn biểu thức A

b,tìm x sao cho A>-1

#Toán lớp 9

1

NL

Nguyễn Lê Phước Thịnh

12 tháng 12 2022

a: \(=\dfrac{x-3\sqrt{x}-x-9}{x-9}:\dfrac{3\sqrt{x}+1-\sqrt{x}+3}{\sqrt{x}\left(\sqrt{x}-3\right)}\)

\(=\dfrac{-3\left(\sqrt{x}+3\right)}{x-9}\cdot\dfrac{\sqrt{x}\left(\sqrt{x}-3\right)}{2\sqrt{x}+4}=\dfrac{-3\sqrt{x}}{2\sqrt{x}+4}\)

b: Để A>-1 thì A+1>0

=>\(-3\sqrt{x}+2\sqrt{x}+4>0\)

=>-căn x>-4

=>0<x<16

Đúng(0)

TN

tung nguyen

16 tháng 2 2020 - olm

cho biểu thức P=(\(\frac{x-3\sqrt{x}}{x-6\sqrt{x}+9}\)-(\(\frac{2\sqrt{x}-1}{x-3\sqrt{x}}\)).\(\frac{x-9}{\sqrt{x}+3}\)

Tìm điều kiện xác định và rút gọn biểu thức P

#Toán lớp 9

0

NB

Nguyễn Bảo Long

30 tháng 10 2016 - olm

cho biểu thức p = (\(1-\frac{x-3\sqrt{x}}{x-9}\)) : (\(\frac{\sqrt{x}-3}{2-\sqrt{x}}+\frac{\sqrt{x}-2}{3+\sqrt{x}}-\frac{9-x}{x+\sqrt{x}-6}\))

a) rút gọn biểu thức P

b) tìm giá trị của x để P = 1

#Toán lớp 9

0

Chúng tôi đề xuất

Tài nguyên

Ứng dụng mobile

Bảng xếp hạng

Các khóa học có thể bạn quan tâm

Yêu cầu VIP