Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

cho 2014=2013+1 thay vào ta có:\(B=x^{2013}-\left(2013+1\right)x^{2012}+\left(2013+1\right)x^{2011}-...-\left(2013+1\right)x^2+\left(2013+1\right)x-1\)
\(=x^{2013}-\left(x+1\right)x^{2012}+\left(x+1\right)x^{2011}-...-\left(x+1\right)x^2+\left(x+1\right)x-1\)
\(=x^{2013}-x^{2013}-x^{2012}+x^{2012}+x^{2011}-...-x^3-x^2+x^2+x-1\)
\(=x-1=2013-1=2012\)

a) Ta có:
\(M\left(x\right)=A\left(x\right)-2.B\left(x\right)+C\left(x\right)\)
\(=\left(2x^5-4x^3+x^2-2x+2\right)-2.\left(x^5-2x^4+x^2-5x+3\right)+\left(x^4+3x^3+3x^2-8x+4\frac{3}{16}\right)\)
\(=2x^5-4x^3+x^2-2x+2-2x^5+4x^4-2x^2+10x-6+x^4+4x^3+3x^2-8x+\frac{67}{16}\)
\(=\left(2x^5-2x^5\right)+\left(4x^4+x^4\right)+\left(-4x^3+4x^3\right)+\left(x^2-2x^2+3x^2\right)+\left(-2x+10x-8x\right)+\left(2-6+\frac{67}{16}\right)\)
\(=0+5x^4+0+2x^2+0+\frac{3}{16}\)
\(=5x^4+2x^2+\frac{3}{16}\)
b) Thay \(x=-\sqrt{0,25}=-0,5\); ta có:
\(M\left(-0,5\right)=5.\left(-0,5\right)^4+2.\left(-0,5\right)^2+\frac{3}{16}\)
\(=5.0,0625+2.0,25+\frac{3}{16}\)
\(=\frac{5}{16}+\frac{8}{16}+\frac{3}{16}=\frac{16}{16}=1\)
c) Ta có:
\(x^4\ge0\) với mọi x
\(x^2\ge0\) với mọi x
\(\Rightarrow5x^4+2x^2+\frac{3}{16}>0\) với mọi x
Do đó không có x để M(x)=0

a) \(4x-7>0\Leftrightarrow4x>7\)\(\Leftrightarrow x>\frac{7}{4}\)
b) \(-5x+8>0\Leftrightarrow5x<8\Leftrightarrow x<\frac{8}{5}\)
c)\(9x-10\le0\Leftrightarrow9x\le10\)\(\Leftrightarrow x\le\frac{10}{9}\)
d) \(\left(x+1\right)^2+4\le x^2+3x+10\)\(\Leftrightarrow x^2-2x+1+4\le x^2+3x+10\)
\(\Leftrightarrow5x\ge-5\Leftrightarrow x\ge-1\)
a,
4x - 7 > 0
↔ 4x > 7
↔ x > \(\dfrac{7}{4}\)
Vậy tập nghiệm của bất phương trình là S = { x / x>\(\dfrac{7}{4}\) }
b,
-5x + 8 > 0
↔ 8 > 5x
↔ \(\dfrac{8}{5}\) > x
Vậy tập nghiệm của bất phương trình là S = { x / \(\dfrac{8}{5}\) > x }
c,
9x - 10 ≤ 0
↔ 9x ≤ 10
↔ x ≤ \(\dfrac{10}{9}\)
Vậy tập nghiệm của bất phương trình là S = { x / x ≤ \(\dfrac{10}{9}\) }
d,
( x - 1 )\(^2\) + 4 ≤ x\(^2\) + 3x + 10
↔ x\(^2\) - 2x +1 +4 ≤ x\(^2\) + 3x + 10
↔ 1 + 4 - 10 ≤ x \(^2\) - x\(^2\) + 3x + 2x
↔ -5 ≤ 5x
↔ -1 ≤ x
Vậy tập nghiệm của bất phương trình là S = { x / -1 ≤ x}

a) ĐK: \(x\ge0,x\ne1,x\ne\frac{1}{4}\)
\(A=1+\left(\frac{2x+\sqrt{x}-1}{1-x}-\frac{2x\sqrt{x}-\sqrt{x}+x}{1-x\sqrt{x}}\right)\frac{x-\sqrt{x}}{2\sqrt{x}-1}\)
\(A=1+\left[\frac{\left(2\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}+1\right)\left(1-\sqrt{x}\right)}-\frac{\sqrt{x}\left(2\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\left(1-\sqrt{x}\right)\left(x+\sqrt{x}+1\right)}\right]\frac{\sqrt{x}\left(\sqrt{x}-1\right)}{2\sqrt{x}-1}\)
\(A=1+\left[\frac{2\sqrt{x}-1}{1-\sqrt{x}}-\frac{\sqrt{x}\left(2\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\left(1-\sqrt{x}\right)\left(x+\sqrt{x}+1\right)}\right]\frac{\sqrt{x}\left(\sqrt{x}-1\right)}{2\sqrt{x}-1}\)
\(A=1-\sqrt{x}+\frac{x\left(\sqrt{x}+1\right)}{x+\sqrt{x}+1}\)
\(A=\frac{x+1}{x+\sqrt{x}+1}\)
Để \(A=\frac{6-\sqrt{6}}{5}\Rightarrow\frac{x+1}{x+\sqrt{x}+1}=\frac{6-\sqrt{6}}{5}\)
\(\Rightarrow5x+5=\left(6-\sqrt{6}\right)x+\left(6-\sqrt{6}\right)\sqrt{x}+6-\sqrt{6}\)
\(\Rightarrow\left(1-\sqrt{6}\right)x+\left(6-\sqrt{6}\right)\sqrt{x}+1-\sqrt{6}=0\)
\(\Rightarrow x-\sqrt{6}.\sqrt{x}+1=0\)
\(\Rightarrow\orbr{\begin{cases}\sqrt{x}=\frac{\sqrt{2}+\sqrt{6}}{2}\\\sqrt{x}=\frac{-\sqrt{2}+\sqrt{6}}{2}\end{cases}}\Rightarrow\orbr{\begin{cases}x=2+\sqrt{3}\\x=2-\sqrt{3}\end{cases}}\left(tmđk\right)\)
b) Xét \(A-\frac{2}{3}=\frac{x+1}{x+\sqrt{x}+1}-\frac{2}{3}=\frac{3x+3-2x-2\sqrt{x}-2}{3\left(x+\sqrt{x}+1\right)}\)
\(=\frac{x-2\sqrt{x}+1}{3\left(x+\sqrt{x}+1\right)}=\frac{\left(\sqrt{x}-1\right)^2}{3\left(x+\sqrt{x}+1\right)}\)
Do \(x\ge0,x\ne1,x\ne\frac{1}{4}\Rightarrow\left(\sqrt{x}-1\right)^2>0\)
Lại có \(x+\sqrt{x}+1=\left(\sqrt{x}+\frac{1}{2}\right)+\frac{3}{4}>0\)
Nên \(A-\frac{2}{3}>0\Rightarrow A>\frac{2}{3}\).

Đáp án A
Phương pháp: Chia cả 2 vế cho 3x, đặt  , tìm điều kiện của t.
, tìm điều kiện của t.
Đưa về bất phương trình dạng ![]()
Cách giải :
![]()

Ta có 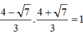
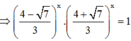
Đặt  , khi đó phương trình trở thành
, khi đó phương trình trở thành
![]()
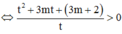
![]()
![]()
![]()
![]()
Ta có: 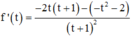
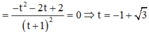
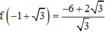
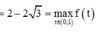
Vậy 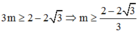

Chọn B.
Phương pháp:
Đưa phương trình về dạng tích, giải phương trình tìm nghiệm và tìm điều kiện để bài toán thỏa.
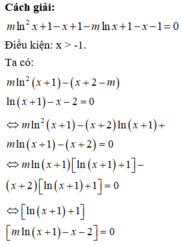
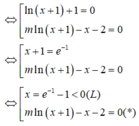

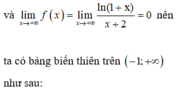
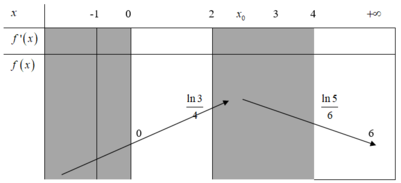


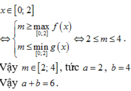

Đáp án A.
Bất phương trình log 2 x 2 - 2 x + m + 4 log 4 x 2 - 2 x + m ≤ 5
Vậy a = 2, b = 4 hay a + b = 6.