Biến đổi thành tích các biểu thức sau:a. 1 – sinxb. 1 + sinx...

K

Khách

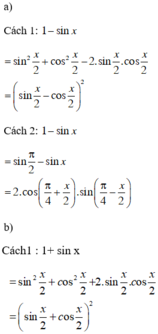
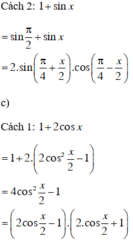
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Mua vip

PT

Pham Trong Bach

1 tháng 7 2017

Biến đổi thành tích các biểu thức sau:

a. 1 – sinx

b. 1 + sinx

c. 1 + 2cosx

d. 1 – 2sinx

#Toán lớp 10

1

CM

Cao Minh Tâm

1 tháng 7 2017

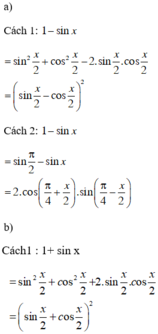
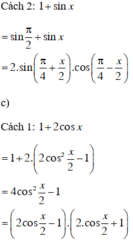
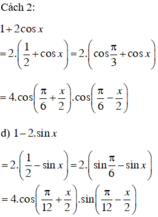
Giải bài 7 trang 155 SGK Đại Số 10 | Giải toán lớp 10

Giải bài 7 trang 155 SGK Đại Số 10 | Giải toán lớp 10

Giải bài 7 trang 155 SGK Đại Số 10 | Giải toán lớp 10

Các câu hỏi dưới đây có thể giống với câu hỏi trên

JE

Julian Edward

9 tháng 7 2020

Tìm nghiệm của các phương trình sau:

a) \(sinx\left(sinx-2\right)=0\)

b) \(\left(2sinx-3\right)\left(2sinx-\sqrt{2}\right)=0\)

c) \(\frac{2sinx-1}{2sinx+1}=3\)

d) \(\frac{2}{3-sinx}=1\)

#Toán lớp 10

1

NV

Nguyễn Việt Lâm

Giáo viên

9 tháng 7 2020

a/ \(\Leftrightarrow\left[{}\begin{matrix}sinx=0\\sinx-2=0\left(vn\right)\end{matrix}\right.\) (vô nghiệm do \(sinx\le1\) ; \(\forall x\))

\(\Leftrightarrow x=k\pi\)

b/ \(\Leftrightarrow\left[{}\begin{matrix}2sinx-3=0\\2sinx-\sqrt{2}=0\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}sinx=\frac{3}{2}\left(vn\right)\\sinx=\frac{\sqrt{2}}{2}=sin\frac{\pi}{4}\end{matrix}\right.\) (lý do vô nghiệm như câu a)

\(\Rightarrow\left[{}\begin{matrix}sinx=\frac{\pi}{4}+k2\pi\\sinx=\frac{3\pi}{4}+k2\pi\end{matrix}\right.\)

c/ ĐKXĐ: \(sinx\ne-\frac{1}{2}\)

\(\Leftrightarrow2sinx-1=6sinx+3\)

\(\Leftrightarrow4sinx=-4\Rightarrow sinx=-1\)

\(\Rightarrow x=-\frac{\pi}{2}+k2\pi\)

d/ \(\Leftrightarrow2=3-sinx\)

\(\Leftrightarrow sinx=1\Rightarrow x=\frac{\pi}{2}+k2\pi\)

(các câu \(k\in Z\) )

BT

Bành Thụy Hóii

29 tháng 10 2019

cho tanx = -1. tính giá trị biểu thức P = \(\frac{sinx+2cosx}{cosx+2sinx}\)

Cho tanx \(\sqrt{2}\). Tính B = \(\frac{sin\alpha-cos\alpha}{sin^3\alpha+3cos^3\alpha+2sin\alpha}\)

Cho \(sinx+cosx=\frac{1}{5}\) Tính P = | sinx - cosx |

#Toán lớp 10

0

NQ

Nguyễn Quỳnh

29 tháng 5 2020

1/Chứng minh rằng :

a/ cot\(^2\)x \(-cos^2x=cot^2x.cos^2x\)

b/ \(\frac{cosx+sinx}{cosx-sinx}-\frac{cosx-sinx}{cosx+sinx}=2tan2x\)

c/ \(\frac{sin4x+cos2x}{1+sin2x-cos4x}=cot2x\)

2/ Rút gọn biểu thức

A=\(sin^3+sin^2xcosx+sinxcos^2x+cos^3x\)

B=\(tanx\left(\frac{1+cos^2x}{sinx}-sinx\right)\)

#Toán lớp 10

1

NV

Nguyễn Việt Lâm

Giáo viên

29 tháng 5 2020

\(cot^2x-cos^2x=\frac{cos^2x}{sin^2x}-cos^2x=cos^2x\left(\frac{1}{sin^2x}-1\right)=\frac{cos^2x\left(1-sin^2x\right)}{sin^2x}\)

\(=cos^2x.\left(\frac{cos^2x}{sin^2x}\right)=cot^2x.cos^2x\)

\(\frac{cosx+sinx}{cosx-sinx}-\frac{cosx-sinx}{cosx+sinx}=\frac{\left(cosx+sinx\right)^2-\left(cosx-sinx\right)^2}{\left(cosx-sinx\right)\left(cosx+sinx\right)}\)

\(=\frac{cos^2x+sin^2x+2sinx.cosx-\left(cos^2x+sin^2x-2sinx.cosx\right)}{cos^2x-sin^2x}=\frac{4sinx.cosx}{cos2x}=\frac{2sin2x}{cos2x}=2tan2x\)

\(\frac{sin4x+cos2x}{1-cos4x+sin2x}=\frac{2sin2x.cos2x+cos2x}{1-\left(1-2sin^22x\right)+sin2x}=\frac{cos2x\left(2sin2x+1\right)}{sin2x\left(2sin2x+1\right)}=\frac{cos2x}{sin2x}=cot2x\)

\(A=sin^2x\left(sinx+cosx\right)+cos^2x\left(sinx+cosx\right)\)

\(=\left(sin^2x+cos^2x\right)\left(sinx+cosx\right)=sinx+cosx\)

\(B=\frac{sinx}{cosx}\left(\frac{1+cos^2x-sin^2x}{sinx}\right)=\frac{sinx}{cosx}\left(\frac{2cos^2x}{sinx}\right)=2cosx\)

TL

Thùy Lâm

1 tháng 5 2020

Gọi M = 1 + sin2x + cos2x thì:

A. M = 2cosx.(sinx - cosx)

B. M = cosx.(sinx + cosx)

C. M = \(\sqrt{2}\)cosx.cos(x - \(\frac{\pi}{4}\))

D. M = \(2\sqrt{2}\)cosx.cos(x - \(\frac{\pi}{4}\))

#Toán lớp 10

1

NV

Nguyễn Việt Lâm

Giáo viên

1 tháng 5 2020

\(M=sin^2x+cos^2x+2sinx.cosx+cos^2x-sin^2x\)

\(=\left(sinx+cosx\right)^2+\left(cosx-sinx\right)\left(cosx+sinx\right)\)

\(=\left(sinx+cosx\right)\left(sinx+cosx+cosx-sinx\right)\)

\(=2cosx\left(sinx+cosx\right)\)

\(=2\sqrt{2}cosx.cos\left(x-\frac{\pi}{4}\right)\)

TL

Thùy Lâm

1 tháng 5 2020

Cảm ơn bạn nhá!!!

DH

Đặng Hoàng Anh

8 tháng 8 2021 - olm

Biến đổi biểu thức sau thành tích:

a) \(1-sin(x)\)

b) \(1+sin(x)\)

c) \(1-2cos(x)\)

d) \(1+2cos(x)\)

#Toán lớp 10

1

NV

Nguyễn Văn Lâm ( ✎﹏IDΣΛ亗 )

13 tháng 8 2021

\(a)\)

\(1-sin\left(x\right)\)

\(=sin^2\frac{x}{2}+cos^2\frac{x}{2}-2.sin\frac{x}{2}.cos\frac{x}{2}\)

\(=\left(sin\frac{x}{2}-cos\frac{x}{2}\right)^2\)

\(b)\)

\(1+sin\left(x\right)\)

\(=sin^2\frac{x}{2}+cos^2\frac{x}{2}+2.sin\frac{x}{2}.cos\frac{x}{2}\)

\(=\left(sin\frac{x}{2}+cos\frac{x}{2}\right)^2\)

\(d)\)

\(1+2cos\left(x\right)\)

\(=2\left(\frac{1}{2}+cosx\right)\)

\(=2\left(cos60^o+cosx\right)\)

\(=4\left(cos\frac{60^o+x}{2}cos\frac{60^o-x}{2}\right)\)

\(=4cos\left(30^o+\frac{x}{2}\right)cos\left(30^o-\frac{x}{2}\right)\)

NH

Nguyễn Hà Chi

5 tháng 11 2019

rút gọn các biểu thức lượng giác sau:

\(\frac{sin^2x}{cosx\left(1+tanx\right)}-\frac{cos^2x}{sinx\left(1+cotx\right)}=sinx-cosx\)

\(\left(tanx+\frac{cosx}{1+sinx}\right)\left(cotx+\frac{sinx}{1+cosx}\right)=\frac{1}{sinx.cosx}\)

#Toán lớp 10

2

NH

Nguyễn Hà Chi

5 tháng 11 2019

đề bài đầy đủ: rút gọn các biểu thức lượng giác sau trên điều kiện xác định của chúng:

NV

Nguyễn Việt Lâm

Giáo viên

6 tháng 11 2019

\(\frac{sin^2x}{cosx+cosx.\frac{sinx}{cosx}}-\frac{cos^2x}{sinx+sinx.\frac{cosx}{sinx}}=\frac{sin^2x}{sinx+cosx}-\frac{cos^2x}{sinx+cosx}=\frac{sin^2x-cos^2x}{sinx+cosx}\)

\(=\frac{\left(sinx+cosx\right)\left(sinx-cosx\right)}{sinx+cosx}=sinx-cosx\)

\(\left(\frac{sinx}{cosx}+\frac{cosx}{1+sinx}\right)\left(\frac{cosx}{sinx}+\frac{sinx}{1+cosx}\right)=\left(\frac{sinx+sin^2x+cos^2x}{cosx\left(1+sinx\right)}\right)\left(\frac{cosx+cos^2x+sin^2x}{sinx\left(1+cosx\right)}\right)\)

\(=\left(\frac{sinx+1}{cosx\left(1+sinx\right)}\right)\left(\frac{cosx+1}{sinx\left(1+cosx\right)}\right)=\frac{1}{sinx.cosx}\)

NQ

Nguyễn Quỳnh

4 tháng 5 2020

Bài 1 : Chứng minh rằng a) \(\frac{1-sinx}{cosx}=\frac{cosx}{1+sinx}\) b) \(\frac{tanx}{sinx}-\frac{sinx}{cotx}=cosx\) Bài 2 : Chứng minh các biểu thức sau độc lập với biến x A= \(\frac{cot^2x-cos^2x}{cot^2x}+\frac{sinxcosx}{cotx}\) B= \(cos^4x+sin^2xcos^2x+sin^{2^{ }}x\) Bài 3 : Tính giá trị các biểu thức lượng giác A=\(\frac{5cosx+6tanx}{5cosx-6tanx}\) biết tanx=2 B= \(\frac{4sinxcosx-3cos^2x}{^{ }1+3sin^2x}\) biết cotx = -6 Bài 4 : Tính...

#Toán lớp 10

7

NQ

Nguyễn Quỳnh

4 tháng 5 2020

cos đó bạn

AH

Akai Haruma

Giáo viên

4 tháng 5 2020

Lời giải:

a)

\(\cos 2a=\frac{2}{5}\Rightarrow \sin ^22a=1-(\cos 2a)^2=1-(\frac{2}{5})^2=\frac{21}{25}\)

Vì $a\in (0; \frac{\pi}{4})\Rightarrow 2a\in (0; \frac{\pi}{2})$

$\Rightarrow \sin 2a>0\Rightarrow \sin 2a=\frac{\sqrt{21}}{5}$

$\tan 2a=\frac{\sin 2a}{\cos 2a}=\frac{\sqrt{21}}{5.\frac{2}{5}}=\frac{\sqrt{21}}{2}$

$\cot 2a=\frac{1}{\tan 2a}=\frac{2}{\sqrt{21}}$

-------------------------

$\sin 2a=\frac{24}{25}\Rightarrow \cos ^22a=1-(\sin 2a)^2=\frac{49}{625}$

$a\in [\frac{-3}{4}\pi; \frac{-\pi}{2}]\Rightarrow 2a\in [\frac{-3}{2}\pi ; -\pi]\Rightarrow \cos 2a< 0$

$\Rightarrow \cos 2a=\frac{-7}{25}$

$\Rightarrow \tan 2a=\frac{\sin 2a}{\cos 2a}=\frac{24}{25.\frac{-7}{25}}=\frac{-24}{7}$

$\Rightarrow \cot 2a=\frac{-7}{24}$

TT

TRẦN THỊ HÀ MY

16 tháng 5 2019

chứng minh đẳng thức lượng giác \(\frac{sin2x-cosx}{2sinx-1}\)+ sinx = \(\sqrt{2}\)sin(x+\(\frac{\text{π}}{4}\))

#Toán lớp 10

1

NV

Nguyễn Việt Lâm

Giáo viên

16 tháng 5 2019

\(\frac{sin2x-cosx}{2sinx-1}+sinx=\frac{2sinx.cosx-cosx}{2sinx-1}+sinx\)

\(=\frac{cosx\left(2sinx-1\right)}{2sinx-1}+sinx=cosx+sinx=\sqrt{2}sin\left(x+\frac{\pi}{4}\right)\)

N

Nam

16 tháng 6 2020

Rút gọn biểu thức A= \(\frac{sinx+sin3x}{2cosx}\)

#Toán lớp 10

1

NV

Nguyễn Việt Lâm

Giáo viên

16 tháng 6 2020

\(A=\frac{sin3x+sinx}{2cosx}=\frac{2sin2x.cosx}{2cosx}=sin2x\)