Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: \(=3\sqrt{5}-\left(\sqrt{5}-2\right)=2\sqrt{5}+2\)
b: \(=\left|a-b\right|-\left|b-c\right|-\left|c-d\right|\)
\(=b-a-\left(c-b\right)-\left(d-c\right)\)
=b-a-c+b-d+c
=2b-d-a

Câu 3 :
\(ĐKXĐ:x>0\)
\(P=\left(\frac{2}{\sqrt{x}}+\frac{\sqrt{x}}{\sqrt{x}+2}\right):\frac{2\sqrt{x}}{x+2\sqrt{x}}\)
\(\Leftrightarrow P=\frac{2\sqrt{x}+4+x}{x+2\sqrt{x}}\cdot\frac{x+2\sqrt{x}}{2\sqrt{x}}\)
\(\Leftrightarrow P=\frac{2\sqrt{x}+4+x}{2\sqrt{x}}\)
b) Để P = 3
\(\Leftrightarrow\frac{2\sqrt{x}+4+x}{x+2\sqrt{x}}=3\)
\(\Leftrightarrow2\sqrt{x}+4+x=6\sqrt{x}\)
\(\Leftrightarrow x-4\sqrt{x}+4=0\)
\(\Leftrightarrow\left(\sqrt{x}-2\right)^2=0\)
\(\Leftrightarrow\sqrt{x}-2=0\)
\(\Leftrightarrow\sqrt{x}=2\)
\(\Leftrightarrow x=4\)(tm)
Vậy để \(P=3\Leftrightarrow x=4\)
Câu 1 : Hình như sai đề !! Mik sửa :
\(ĐKXĐ:\hept{\begin{cases}x\ge0\\x\ne4\end{cases}}\)
\(A=\left(\frac{x}{x\sqrt{x}-4\sqrt{x}}-\frac{6}{3\sqrt{x}-6}+\frac{1}{\sqrt{x}+2}\right):\left(\sqrt{x}-2+\frac{10-x}{\sqrt{x}+2}\right)\)
\(\Leftrightarrow A=\left(\frac{\sqrt{x}}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}-\frac{2}{\sqrt{x}-2}+\frac{1}{\sqrt{x}+2}\right):\left(\frac{x-4+10-x}{\sqrt{x}+2}\right)\)
\(\Leftrightarrow A=\frac{\sqrt{x}-2\sqrt{x}-4+\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}:\frac{6}{\sqrt{x}+2}\)
\(\Leftrightarrow A=\frac{-6\left(\sqrt{x}+2\right)}{6\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\)
\(\Leftrightarrow A=-\frac{1}{\sqrt{x}-2}\)
b) Để A < 2
\(\Leftrightarrow-\frac{1}{\sqrt{x}-2}< 2\)
\(\Leftrightarrow-1< 2\sqrt{x}-4\)
\(\Leftrightarrow2\sqrt{x}>3\)
\(\Leftrightarrow\sqrt{x}>1,5\)
\(\Leftrightarrow x>2,25\)
Vậy để \(A< 2\Leftrightarrow x>2,25\)

Bài 1:
a: \(=\sqrt{\dfrac{7-4\sqrt{3}}{2-\sqrt{3}}}\cdot\sqrt{2+\sqrt{3}}\)
\(=\sqrt{2-\sqrt{3}}\cdot\sqrt{2+\sqrt{3}}=1\)
Bài 2:
\(VT=\left(4+\sqrt{15}\right)\cdot\left(\sqrt{5}-\sqrt{3}\right)\cdot\sqrt{8-2\sqrt{15}}\)
\(=\left(4+\sqrt{15}\right)\left(8-2\sqrt{15}\right)\)
\(=32-8\sqrt{15}+8\sqrt{15}-30=2\)

a. \(\sqrt{4\left(a-3\right)^2}=2.|a-3|=2\left(a-3\right)\) (vì a \(\ge3\) nên a-3\(\ge\) 0. Do đó: \(|a-3|=a-3\))
b. \(\sqrt{9\left(b-2\right)^2}=3.|b-2|=3\left(2-b\right)\) (vì b < 2 nên b-2 < 0. Do đó : \(|b-2|=2-b\))
c. \(\sqrt{a^2\left(a+1\right)^2}=a\left(a+1\right)\) ( vì a > 0)
d. \(\sqrt{b^2\left(b-1\right)^2}=b\left(b-1\right)\) (vì b < 0)

a) \(\left(2-\sqrt{2}\right)\left(-5\sqrt{2}\right)-\left(3\sqrt{2}-5\right)^2\)
\(=-10\sqrt{2}+5.2-\left(18-30\sqrt{2}+25\right)\)
\(=-10\sqrt{2}+10-18+30\sqrt{2}-25\)
\(=20\sqrt{2}-33\)
b) câu b đề sai
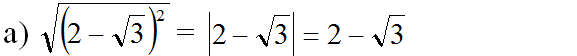
a) \(\sqrt{\left(2-\sqrt{3}\right)^2}\) = / 2 - \(\sqrt{3}\) / = 2 - \(\sqrt{3}\) ( vì 2 > \(\sqrt{3}\) )
b) \(\sqrt{\left(3-\sqrt{11}\right)^2}\) = / 3 - \(\sqrt{11}\) / = \(\sqrt{11}\) - 3 ( vì \(\sqrt{11}\) > 3 )
c) \(2\sqrt{a^2}\) = 2/a / = 2a (vì a> 0 )
d) \(3\sqrt{\left(a-2\right)^2}\) = 3 / a - 2 / = 3 ( 2 - a ) = 6 - 3a (vì a < 2 )