Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) <=>
Miền nghiệm của hệ bất phương trình là miền không bị gạch sọc ở hình bên (không kể các điểm).
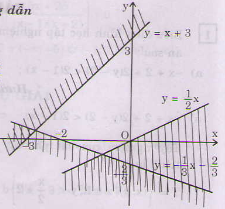
b) <=>
Miền nghiệm của hệ bất phương trình là miền tam giác ABC bao gồm cả các điểm trên cạnh AC và cạnh BC (không kể các điểm của cạnh AB).
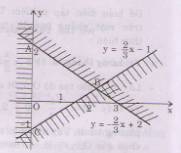

a) \(\left\{{}\begin{matrix}x+3y+2z=8\\2x+2y+z=6\\3x+y+z=6\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}x=1\\y=1\\z=2\end{matrix}\right.\)
b) \(\left\{{}\begin{matrix}x-3y+2z=-7\\-2x+4y+3z=8\\3x+y-z=5\end{matrix}\right.\)\(\Rightarrow\left\{{}\begin{matrix}x=\dfrac{11}{14}\\y=\dfrac{5}{2}\\z=-\dfrac{1}{7}\end{matrix}\right.\)
a) Đặt \(\left\{{}\begin{matrix}x+3y+2z=8\left(1\right)\\2x+2y+z=6\left(2\right)\\3x+y+z=6\left(3\right)\end{matrix}\right.\)
Cộng \(\left(2\right)+\left(3\right)\) ta có:\(\left\{{}\begin{matrix}x+3y+2z=8\left(1\right)\\2x+2y+z=6\left(2\right)\\5x+3y+2z=12\left(4\right)\end{matrix}\right.\)
Trừ \(\left(4\right)-\left(1\right)\) ta được: \(4x=4\Leftrightarrow x=1\).
Thay vào hệ phương trình ta được:
\(\left\{{}\begin{matrix}1+3y+2z=8\\2.1+2y+z=6\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}y=1\\z=2\end{matrix}\right.\).
Vậy hệ phương trình có nghiệm: \(\left\{{}\begin{matrix}x=1\\y=1\\z=2\end{matrix}\right.\).

a. \(\left\{{}\begin{matrix}3x-5y=6\\4x+7y=-8\end{matrix}\right.\)
\(x=\dfrac{2}{41}\) ; \(y=\dfrac{-48}{41}\)
b. \(\left\{{}\begin{matrix}\text{−2x+3y=5}\\5x+2y=4\end{matrix}\right.\)
\(x=\dfrac{2}{19};y=\dfrac{33}{19}\)
c.\(\left\{{}\begin{matrix}\text{2x−3y+4z=−5}\\-4x+5y-z=6\\3x+4y-3z=7\end{matrix}\right.\)
\(x=\dfrac{22}{101};y=\dfrac{131}{101};z=\dfrac{-39}{101}\)
d. \(\left\{{}\begin{matrix}\text{− x + 2 y − 3 z = 2}\\2x+y+2z=-3\\-2x-3y+z=5\end{matrix}\right.\)
\(x=-4;y=\dfrac{11}{7};z=\dfrac{12}{7}\)
a)x=0,05 ; y=-1,17
b.x=0,11 ; y=1,74
c.x=0,22 ;y=1,29 z=-0.39
d.x=-4 y=1,57 z=1,71

a) \(\left\{{}\begin{matrix}x+2y-3z=2\\2x+7y+z=5\\-3x+3y-2z=-7\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x+2y-3z=2\\3y+7z=1\\-32z=-4\end{matrix}\right.\)
Đáp số : \(\left(x,y,z\right)=\left(\dfrac{55}{24},\dfrac{1}{24},\dfrac{1}{8}\right)\)
b) \(\left\{{}\begin{matrix}-x-3y+4z=3\\3x+4y-2z=5\\2x+y+2z=4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-x-3y+4z=3\\-5y+10z=14\\-5y+10z=10\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-x-3y+4z=3\\-5y+10z=14\\0y+0z=-4\end{matrix}\right.\)
Phương trình cuối vô nghiệm, suy ra hệ phương trình đã cho vô nghiệm

b.
Với \(x=0\) không phải nghiệm
Với \(x\ne0\) hệ tương đương:
\(\left\{{}\begin{matrix}\dfrac{y}{x^2}+\dfrac{y^2}{x}=-6\\\dfrac{1}{x^3}+y^3=19\end{matrix}\right.\)
Đặt \(\left(\dfrac{1}{x};y\right)=\left(u;v\right)\) ta được: \(\left\{{}\begin{matrix}uv^2+u^2v=-6\\u^3+v^3=19\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}3uv^2+3u^2v=-18\\u^3+v^3+19\end{matrix}\right.\)
Cộng vế với vế:
\(\left(u+v\right)^3=1\Rightarrow u+v=1\)
Thay vào \(u^2v+uv^2=-6\Rightarrow uv=-6\)
Theo Viet đảo, u và v là nghiệm của:
\(t^2-t-6=0\) \(\Rightarrow\left[{}\begin{matrix}t=-2\\t=3\end{matrix}\right.\) \(\Rightarrow\left(u;v\right)=\left(-2;3\right);\left(3;-2\right)\)
\(\Rightarrow\left(\dfrac{1}{x};y\right)=\left(-2;3\right);\left(3;-2\right)\)
\(\Rightarrow\left(x;y\right)=\left(-\dfrac{1}{2};3\right);\left(\dfrac{1}{3};-2\right)\)
a.
ĐKXĐ: \(x\ne3\)
- Với \(x\ge0\) pt trở thành:
\(\dfrac{x^2-x-12}{x-3}=2x\Rightarrow x^2-x-12=2x^2-6x\)
\(\Leftrightarrow x^2-5x+12=0\) (vô nghiệm)
- Với \(x< 0\) pt trở thành:
\(\dfrac{x^2+x-12}{x-3}=2x\Rightarrow\dfrac{\left(x-3\right)\left(x+4\right)}{x-3}=2x\)
\(\Rightarrow x+4=2x\Rightarrow x=4>0\) (ktm)
Vậy pt đã cho vô nghiệm

b) Đặt \(\left\{{}\begin{matrix}x+y+z=7\left(1\right)\\3x-2y+2z=5\left(2\right)\\4x-y+3z=10\left(3\right)\end{matrix}\right.\)
Cộng \(\left(1\right)+\left(2\right)\) ta có: \(4x-y+3z=12\). (4)
Từ (3) và (4): \(\left\{{}\begin{matrix}4x-y+3z=12\\4x-y+3z=10\end{matrix}\right.\) (vô nghiệm).
Vậy hệ phương trình vô nghiệm.
\(\left\{{}\begin{matrix}2X+Y\ge1\left(1\right)\\X-3Y\le1\left(2\right)\end{matrix}\right.\)
*Giải 2X+Y-1=0
cho đi qua 2 điểm và thử điểm O(0;0) vào (1) và loại đi phần k thỏa mãn
*Tương tự giải X-3Y-1=0
*Lấy giao (1) và (2)