
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\Leftrightarrow\dfrac{2m+3}{5}=\dfrac{5m+2}{3}\\ \Leftrightarrow6m+9=25m+10\\ \Leftrightarrow19m=-1\Leftrightarrow m=-\dfrac{1}{19}\)


b: Xét ΔDEF vuông tại D có DK là đường cao
nên \(DF^2=EF\cdot KF\)

a: \(Q=\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}+2\right)-2\sqrt{x}\left(\sqrt{x}-2\right)-5\sqrt{x}-2}{x-4}:\dfrac{\sqrt{x}\left(3-\sqrt{x}\right)}{\left(\sqrt{x}+2\right)^2}\)
\(=\dfrac{x+3\sqrt{x}+2-2x+4\sqrt{x}-5\sqrt{x}-2}{x-4}\cdot\dfrac{\left(\sqrt{x}+2\right)^2}{\sqrt{x}\left(3-\sqrt{x}\right)}\)
\(=\dfrac{-x+2\sqrt{x}}{\sqrt{x}-2}\cdot\dfrac{\sqrt{x}+2}{\sqrt{x}\left(3-\sqrt{x}\right)}\)
\(=\dfrac{-\sqrt{x}\left(\sqrt{x}-2\right)}{\sqrt{x}\left(\sqrt{x}-2\right)\cdot\left(-1\right)}\cdot\dfrac{\sqrt{x}+2}{\sqrt{x}-3}=\dfrac{\sqrt{x}+2}{\sqrt{x}-3}\)
b: Khi x=4-2căn 3 thì \(Q=\dfrac{\sqrt{3}-1+2}{\sqrt{3}-1-3}=\dfrac{\sqrt{3}+1}{\sqrt{3}-4}=\dfrac{-7-5\sqrt{3}}{13}\)
c: Q>1/6
=>Q-1/6>0
=>\(\dfrac{\sqrt{x}+2}{\sqrt{x}-3}-\dfrac{1}{6}>0\)
=>\(\dfrac{6\sqrt{x}+12-\sqrt{x}+3}{6\left(\sqrt{x}-3\right)}>0\)
=>\(\dfrac{5\sqrt{x}+9}{6\left(\sqrt{x}-3\right)}>0\)
=>căn x-3>0
=>x>9

theo hệ thức lượng trong tam giác vuông ta có :
\(AC^2=HC.BC\)
\(AB^2=HB.BC\) chia các vế vs nhau ta được : \(\frac{AC^2}{AB^2}=\frac{HC}{HB}\)=> \(\frac{HC}{HB}=\left(\sqrt{2}\right)^2=2\)
Ta có : HC = HB + 2 =>\(\frac{HB+2}{HB}=2\)=> HB = 2
=> HC = 2 + 2 = 4 => BC = HB + HC = 2 + 4 = 6
\(AB^2=2.6=12\)=> AB = \(\sqrt{12}=2\sqrt{3}\)
\(\frac{AC}{AB}=\sqrt{2}\)=> \(\frac{AC}{2\sqrt{3}}=\sqrt{2}\)=> AC = \(2\sqrt{6}\)

a) Thay x=4(TMĐK) vào B ta có:
\(B=\dfrac{4-\sqrt{4}}{2\sqrt{4}+1}=\dfrac{2}{5}\)
Vậy x=4 thì B=\(\dfrac{2}{5}\)
b)\(M=A.B\)
M =\(\left(\dfrac{1}{\sqrt{x}-1}+\dfrac{\sqrt{x}}{x-1}\right).\dfrac{x-\sqrt{x}}{2\sqrt{x}+1}\)
M= \(\left(\dfrac{1}{\sqrt{x-1}}+\dfrac{\sqrt{x}}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\right).\dfrac{x-\sqrt{x}}{2\sqrt{x}+1}\)
M= \(\dfrac{2\sqrt{x}+1}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}.\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)}{2\sqrt{x}+1}\)
M= \(\dfrac{\sqrt{x}}{\sqrt{x}+1}\)
c)\(M=\dfrac{1}{3}\)
\(\Leftrightarrow\dfrac{\sqrt{x}}{\sqrt{x}+1}=\dfrac{1}{3}\)
\(\Leftrightarrow3\sqrt{x}=\sqrt{x}+1\)
\(\Leftrightarrow2\sqrt{x}=1\)
\(\Leftrightarrow\sqrt{x}=\dfrac{1}{2}\)
\(\Leftrightarrow x=\dfrac{1}{4}\)
Vậy với x=\(\dfrac{1}{4}\) thì M=\(\dfrac{1}{3}\)

`a)x=64`
`=>N=sqrtx/(sqrtx-3)=8/(8-3)=8/5`
`b)M=(2sqrtx)/(sqrtx-3)-(x+9sqrtx)/(x-9)`
`=(2x+6sqrtx-x-9sqrtx)/(x-9)`
`=(x-3sqrtx)/(x-9)`
`=sqrtx/(sqrtx+3)`
`P=M.N=x/(x-9)`
`c)` So sánh gì với 1?
a) Thay x=64(TMĐK) vào N ta có:
\(N=\dfrac{\sqrt{64}}{\sqrt{64}-5}=\dfrac{8}{3}\)
Vậy x=64 thì N=\(\dfrac{8}{3}\)
b) \(P=M.N\)
\(P=\dfrac{2\sqrt{x}}{\sqrt{x}-3}-\dfrac{x-9\sqrt{x}}{x-9}.\left(\dfrac{\sqrt{x}}{\sqrt{x}-5}\right)\)
\(P=\dfrac{2\sqrt{x}}{\sqrt{x}-3}-\dfrac{x-9\sqrt{x}}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}.\left(\dfrac{\sqrt{x}}{\sqrt{x}-5}\right)\)
\(P=\dfrac{2\sqrt{x}\left(\sqrt{x}+3\right)-x+9\sqrt{x}}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}.\dfrac{\sqrt{x}}{\sqrt{x}+5}\)
\(P=\dfrac{x+15\sqrt{x}}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}.\dfrac{\sqrt{x}}{\sqrt{x}+5}\)
\(P=\dfrac{x}{\left(\sqrt{x}-3\right)\left(\sqrt{x} +3\right)}\)
\(P=\dfrac{x}{x-9}\)



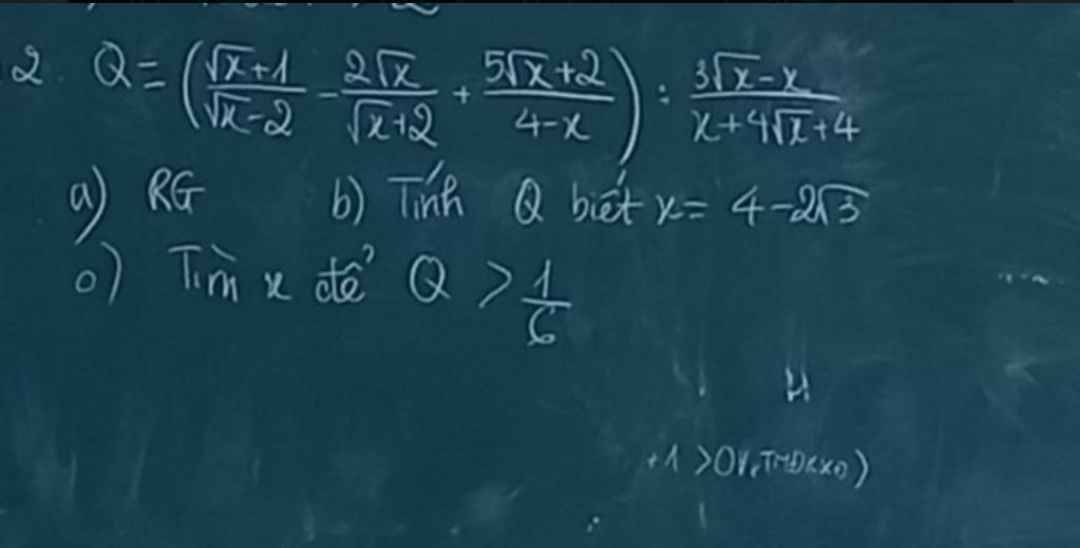
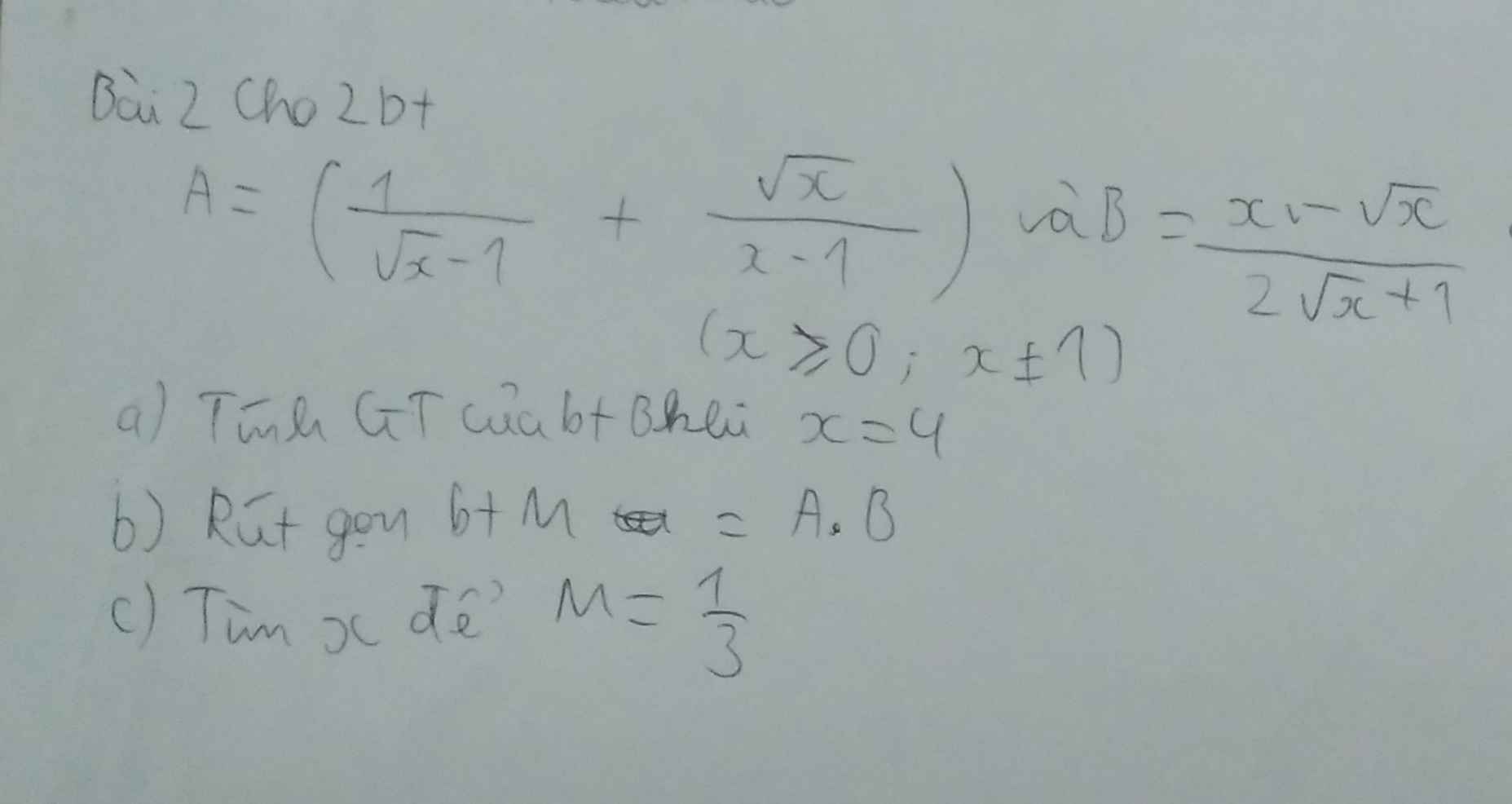
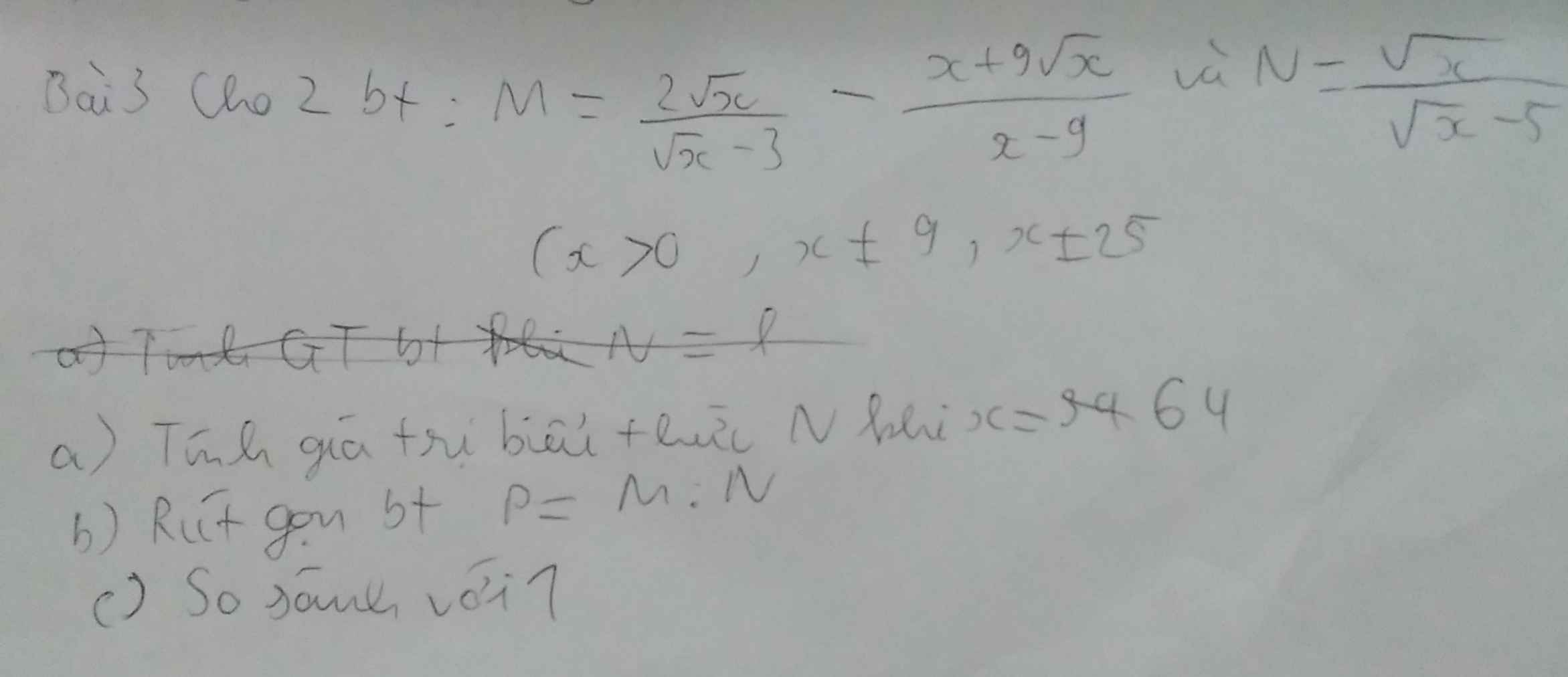
\(\hept{\begin{cases}\left(\sqrt{2}-1\right)x-y=\sqrt{2}\\x+\left(\sqrt{2}+1\right)y=1\end{cases}}\Leftrightarrow\hept{\begin{cases}\sqrt{2}x+\sqrt{2}y=\sqrt{2}+1\\x=1-\left(\sqrt{2}+1\right)y\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}1-\left(\sqrt{2}+1\right)y+y=\frac{2+\sqrt{2}}{2}\\x=1-\left(\sqrt{2}+1\right)y\end{cases}}\Leftrightarrow\hept{\begin{cases}y=-\frac{1}{2}\\x=\frac{3+\sqrt{2}}{2}\end{cases}}\)