


Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.




a) Dễ thấy cosx = 0 không thỏa mãn phương trình đã cho nên chiaw phương trình cho cos2x ta được phương trình tương đương 2tan2x + tanx - 3 = 0.
Đặt t = tanx thì phương trình này trở thành
2t2 + t - 3 = 0 ⇔ t ∈ {1 ; ![]() }.
}.
Vậy ![]()
b) Thay 2 = 2(sin2x + cos2x), phương trình đã cho trở thành
3sin2x - 4sinxcosx + 5cos2x = 2sin2x + 2cos2x
⇔ sin2x - 4sinxcosx + 3cos2x = 0
⇔ tan2x - 4tanx + 3 = 0
⇔ ![]()
⇔ x = ![]() + kπ ; x = arctan3 + kπ, k ∈ Z.
+ kπ ; x = arctan3 + kπ, k ∈ Z.
c) Thay sin2x = 2sinxcosx ; ![]() =
= ![]() (sin2x + cos2x) vào phương trình đã cho và rút gọn ta được phương trình tương đương
(sin2x + cos2x) vào phương trình đã cho và rút gọn ta được phương trình tương đương
![]() sin2x + 2sinxcosx -
sin2x + 2sinxcosx - ![]() cos2x = 0 ⇔ tan2x + 4tanx - 5 = 0 ⇔
cos2x = 0 ⇔ tan2x + 4tanx - 5 = 0 ⇔ ![]()
⇔ x = ![]() + kπ ; x = arctan(-5) + kπ, k ∈ Z.
+ kπ ; x = arctan(-5) + kπ, k ∈ Z.
d) 2cos2x - 3√3sin2x - 4sin2x = -4
⇔ 2cos2x - 3√3sin2x + 4 - 4sin2x = 0
⇔ 6cos2x - 6√3sinxcosx = 0 ⇔ cosx(cosx - √3sinx) = 0
⇔ 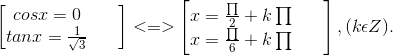

6.
\(\Leftrightarrow\left(sin^2x+cos^2x\right)^3-3sin^2x.cos^2x\left(sin^2x+cos^2x\right)+\frac{1}{2}sinx.cosx=0\)
\(\Leftrightarrow1-3sin^2x.cos^2x+\frac{1}{2}sinx.cosx=0\)
\(\Leftrightarrow1-\frac{3}{4}sin^22x+\frac{1}{4}sin2x=0\)
\(\Leftrightarrow-3sin^22x+sin2x+4=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sin2x=-1\\sin2x=\frac{4}{3}>1\left(l\right)\end{matrix}\right.\)
\(\Rightarrow2x=-\frac{\pi}{2}+k2\pi\)
\(\Rightarrow x=-\frac{\pi}{4}+k\pi\)
5.
\(\Leftrightarrow\left(sin^2x+cos^2x\right)^3-3sin^2x.cos^2x\left(sin^2x+cos^2x\right)=\frac{5}{6}\left[\left(sin^2x+cos^2x\right)^2-2sin^2x.cos^2x\right]\)
\(\Leftrightarrow1-3sin^2x.cos^2x=\frac{5}{6}\left(1-2sin^2x.cos^2x\right)\)
\(\Leftrightarrow1-\frac{3}{4}sin^22x=\frac{5}{6}\left(1-\frac{1}{2}sin^22x\right)\)
\(\Leftrightarrow\frac{1}{3}sin^22x=\frac{1}{6}\)
\(\Leftrightarrow sin^22x=\frac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}sin2x=\frac{\sqrt{2}}{2}\\sin2x=-\frac{\sqrt{2}}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{8}+k\pi\\x=\frac{3\pi}{8}+k\pi\\x=-\frac{\pi}{8}+k\pi\\x=\frac{5\pi}{8}+k\pi\end{matrix}\right.\)

a) Đặt t = cos![]() , t ∈ [-1 ; 1] thì phương trình trở thành
, t ∈ [-1 ; 1] thì phương trình trở thành
(1 - t2) - 2t + 2 = 0 ⇔ t2 + 2t -3 = 0 ⇔ ![]()
Phương trình đã cho tương đương với
cos![]() = 1 ⇔
= 1 ⇔ ![]() = k2π ⇔ x = 4kπ, k ∈ Z.
= k2π ⇔ x = 4kπ, k ∈ Z.
b) Đặt t = sinx, t ∈ [-1 ; 1] thì phương trình trở thành
8(1 - t2) + 2t - 7 = 0 ⇔ 8t2 - 2t - 1 = 0 ⇔ t ∈ {![]() }.
}.
Các nghiệm của phương trình đã cho là nghiệm của hai phương trình sau :
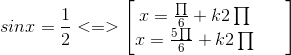
và ![]()
Đáp số : x = ![]() + k2π; x =
+ k2π; x = ![]() + k2π;
+ k2π;
x = arcsin(![]() ) + k2π; x = π - arcsin(
) + k2π; x = π - arcsin(![]() ) + k2π, k ∈ Z.
) + k2π, k ∈ Z.
c) Đặt t = tanx thì phương trình trở thành 2t2 + 3t + 1 = 0 ⇔ t ∈ {-1 ; ![]() }.
}.
Vậy ![]()
d) Đặt t = tanx thì phương trình trở thành
t - ![]() + 1 = 0 ⇔ t2 + t - 2 = 0 ⇔ t ∈ {1 ; -2}.
+ 1 = 0 ⇔ t2 + t - 2 = 0 ⇔ t ∈ {1 ; -2}.
Vậy ![]()

7.
ĐKXĐ: \(x\ne\frac{k\pi}{2}\)
\(\Leftrightarrow8cosx=\frac{\sqrt{3}cosx+sinx}{sinx.cosx}\)
\(\Leftrightarrow8cosx.sinx.cosx=\sqrt{3}cosx+sinx\)
\(\Leftrightarrow4sin2x.cosx=\sqrt{3}cosx+sinx\)
\(\Leftrightarrow2sin3x+2sinx=\sqrt{3}cosx+sinx\)
\(\Leftrightarrow2sin3x=\sqrt{3}cosx-sinx\)
\(\Leftrightarrow sin3x=\frac{\sqrt{3}}{2}cosx-\frac{1}{2}sinx\)
\(\Leftrightarrow sin\left(-3x\right)=sin\left(x-\frac{\pi}{3}\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}-3x=x-\frac{\pi}{3}+k2\pi\\-3x=\frac{4\pi}{3}-x+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{12}+\frac{k\pi}{2}\\x=-\frac{2\pi}{3}+k\pi\end{matrix}\right.\)
5.
\(sin\left(2x+\frac{\pi}{2}+2\pi\right)-2cos\left(x+\frac{\pi}{2}-4\pi\right)=1+2sinx\)
\(\Leftrightarrow sin\left(2x+\frac{\pi}{2}\right)-2cos\left(x+\frac{\pi}{2}\right)=1+2sinx\)
\(\Leftrightarrow cos2x+2sinx=1+2sinx\)
\(\Leftrightarrow cos2x=1\)
\(\Rightarrow x=k\pi\)
6.
\(sin^22x-cos^28x=sin\left(10x+\frac{\pi}{2}+8\pi\right)\)
\(\Leftrightarrow\frac{1-cos4x}{2}-\frac{1+cos16x}{2}=sin\left(10x+\frac{\pi}{2}\right)\)
\(\Leftrightarrow-\left(cos4x+cos16x\right)=2cos10x\)
\(\Leftrightarrow-2cos10x.cos6x=2cos10x\)
\(\Leftrightarrow\left[{}\begin{matrix}cos10x=0\\cos6x=-1\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}10x=\frac{\pi}{2}+k\pi\\6x=\pi+k2\pi\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{20}+\frac{k\pi}{10}\\x=\frac{\pi}{6}+\frac{k\pi}{3}\end{matrix}\right.\)