Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

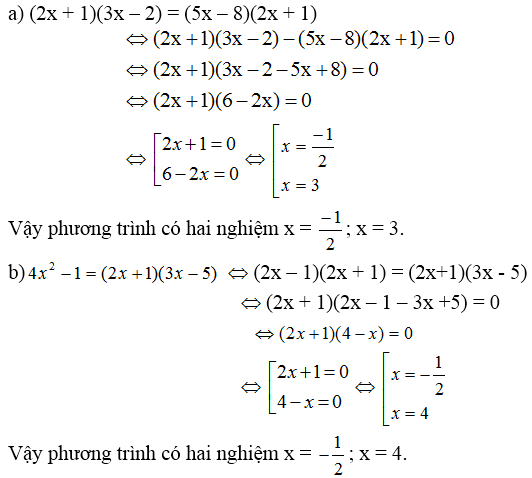
a)(2x+1)(3x-2)=(5x-8)(2x+1)
⇔(2x+1)(3x-2)-(5x-8)(2x+1)=0
⇔(2x+1)(3x-2-5x+8)=0
⇔(2x+1)(-2x+6)=0
⇔2x+1=0 hoặc -2x+6=0
1.2x+1=0⇔2x=-1⇔x=-1/2
2.-2x+6=0⇔-2x=-6⇔x=3
phương trình có 2 nghiệm x=-1/2 và x=3

\(9x^2-1=\left(3x+1\right)\left(2x-3\right)\)
\(\Leftrightarrow\left(3x+1\right)\left(3x-1\right)=\left(3x+1\right)\left(2x-3\right)\)
\(\Leftrightarrow\left(3x+1\right)\left(3x-1\right)-\left(3x+1\right)\left(2x-3\right)=0\)
\(\Leftrightarrow\left(3x+1\right)\left(3x-1-2x+3\right)=0\)
\(\Leftrightarrow\left(3x+1\right)\left(x+2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}3x+1=0\\x+2=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=\frac{-1}{3}\\x=-2\end{cases}}\)
\(2\left(9x^2+6x+1\right)=\left(3x+1\right)\left(x-2\right)\)
\(\Leftrightarrow2\left(3x+1\right)^2=\left(3x+1\right)\left(x-2\right)\)
\(\Leftrightarrow2\left(3x+1\right)^2-\left(3x+1\right)\left(x-2\right)=0\)
\(\Leftrightarrow\left(3x+1\right)\left(6x+2-x+2\right)=0\)
\(\Leftrightarrow\left(3x+1\right)\left(5x+4\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}3x+1=0\\5x+4=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=\frac{-1}{3}\\x=\frac{-4}{5}\end{cases}}\)

a, \(\frac{1-x}{x+1}+3=\frac{2x+3}{x+1}\)
\(=>\frac{1-x+x+1}{x+1}+2=\frac{1}{x+1}+2\)
\(=>\frac{2}{x+1}=\frac{1}{x+1}\)
\(=>2x+2=x+1\)
\(=>2x-x=1-2=-1\)
\(=>x=-1\)
vậy nghiệm của phương trình trên là {-1}
À quên ĐKXĐ của câu a là \(x\ne-1\)
Nên \(x\in\varnothing\)nhé :v

Câu a:
\(2x\left(8x-1\right)^2\left(4x-1\right)=9\)
\(\Leftrightarrow\left(64x^2-16x+1\right)\left(64x^2-16x\right)=72\)
Đặt 64x2 - 16x = t \(\left(t\ge-1\right)\)
\(\Rightarrow t\left(t+1\right)=72\)
\(\Leftrightarrow\left(t+9\right)\left(t-8\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}t=-9\left(loai\right)\\t=8\left(nhan\right)\end{matrix}\right.\)
\(\Rightarrow64x^2-16x=8\)
\(\Leftrightarrow8\left(2x-1\right)\left(4x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=-\dfrac{1}{4}\end{matrix}\right.\)
Câu b:
\(\Leftrightarrow\left(x+1\right)^2\left(2x+1\right)\left(2x+3\right)=18\)
\(\Leftrightarrow\left(4x^2+8x+4\right)\left(4x^2+8x+3\right)=72\)
Đặt 4x2 + 8x + 4 = m \(\left(m\ge0\right)\)
\(\Rightarrow m\left(m-1\right)=72\)
\(\Leftrightarrow\left(m-9\right)\left(m+8\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m=9\left(nhan\right)\\m=-8\left(loai\right)\end{matrix}\right.\)
\(\Rightarrow4\left(x+1\right)^2=9\)
\(\Leftrightarrow x+1=\pm\dfrac{3}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{5}{2}\\x=\dfrac{1}{2}\end{matrix}\right.\)

a) Thay x=2 vào phương trình ta có:
(2.2+1)(9.2+2k)+5(2+2)=40
5(18+2k)+20=40
90+10k=20
10k=-70
k=-7
b) Thay x=1 vào phương trình ta có:
2(2.1+1)+18=3(1+2)(2.1+k)
2+2+18=(3+6)(2+k)
22=20+18k
2=18k
k=1/9

\(a.\Leftrightarrow x^2+x-6+2x^2+4x+2=x^2-6x+9-2x^2+4x\)
\(\Leftrightarrow4x^2+7x-13=0\)(pt vô nghiệm)
\(b.\Leftrightarrow x^3+3x^2+3x+1-x^2+2x+8=x^3-8+2x^2\)
\(\Leftrightarrow5x=-17\Rightarrow x=\frac{-17}{5}\)
Đặt \(t=x^2+2x+2\left(t\ge1\right)\)
\(c.\Leftrightarrow\frac{t-1}{t}+\frac{t}{t+1}=\frac{7}{6}\)\(\Leftrightarrow\frac{t^2-1+t^2}{t^2+t}=\frac{7}{6}\)\(\Leftrightarrow12t^2-6=7t^2+7t\)
\(\Leftrightarrow5t^2-7t-6=0\Rightarrow\orbr{\begin{cases}t=2\left(tm\right)\\t=\frac{-3}{5}\left(l\right)\end{cases}}\)
\(\Rightarrow x^2+2x+2=2\Rightarrow x=-2\)
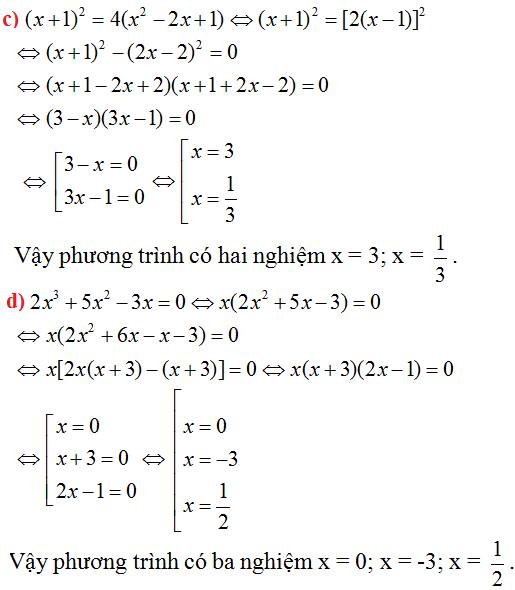
\(\text{Đặt:}x+1=a\Rightarrow\left(2a-1\right)\left(2a+1\right)a^2=\left(4a^2-1\right)a^2=18\Leftrightarrow4a^2\left(4a^2-1\right)=72\)
\(\Rightarrow4a^2=9\left(\text{bạn tự giải phương trình dạng:}k^2+k=72\right)\Rightarrow a^2=\frac{9}{4}\Leftrightarrow a=\pm\frac{3}{2}\)
Đệ đặt khác :)
Đặt \(2x+2=k\Rightarrow x+1=\frac{k}{2}\)
\(pt\Leftrightarrow\left(t-1\right)\cdot\frac{t^2}{4}\cdot\left(t+1\right)=18\)
\(\Leftrightarrow\left(t^2-1\right)\cdot t^2=72\)
\(\Leftrightarrow t^4-t^2-72=0\)
\(\Leftrightarrow\left(t^2-9\right)\left(t^2+8\right)=0\)
Đến đây quá EZ