
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


sin3x + sin5x = 0
⇔ 2sin4x. cosx = 0
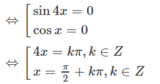
Vậy nghiệm của phương trình là:
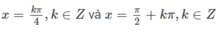

\(4\sin3x+\sin5x-2\sin x\cos2x=0\)
\(\Leftrightarrow\)\(4\sin3x+\sin5x-\sin3x+\sin x=0\)
\(\Leftrightarrow3\sin3x+\sin5x+\sin x=0\)
\(\Leftrightarrow3\sin3x+2\sin3x\cos2x=0\)
\(\Leftrightarrow\sin3x\left(3+2\cos2x\right)=0\)
Đáp số : \(x=k\dfrac{\pi}{3},k\in\mathbb{Z}\)

\(cosx+cos3x+cos2x+cos4x=0\)
\(\Leftrightarrow2cos2x.cosx+2cos3x.cosx=0\)
\(\Leftrightarrow cosx.\left(cos2x+cos3x\right)=0\)
\(\Leftrightarrow cosx.cos\frac{5x}{2}.cos\frac{x}{2}=0\)
\(\Rightarrow\left[{}\begin{matrix}cosx=0\\cos\frac{5x}{2}=0\\cos\frac{x}{2}=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{2}+k\pi\\\frac{5x}{2}=\frac{\pi}{2}+k\pi\\\frac{x}{2}=\frac{\pi}{2}+k\pi\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{2}+k\pi\\x=\frac{\pi}{5}+\frac{k2\pi}{5}\\x=\pi+k2\pi\end{matrix}\right.\)
\(sinx+sin7x+sin3x+sin5x=0\)
\(\Leftrightarrow2sin4x.cos3x+2sin4x.cosx=0\)
\(\Leftrightarrow sin4x\left(cos3x+cosx\right)=0\)
\(\Leftrightarrow sin4x.cos2x.cosx=0\)
\(\Leftrightarrow sin4x=0\)
\(\Rightarrow4x=k\pi\Rightarrow x=\frac{k\pi}{4}\)
Lý do chỉ cần 1 pt sin4x=0 do sin4x bao hàm cả cosx và cos2x ở trong đó

\(\Leftrightarrow sin5x=-sin3x\)
\(\Leftrightarrow sin5x=sin\left(-3x\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}5x=-3x+k2\pi\\5x=\pi+3x+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}8x=k2\pi\\2x=\pi+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{k\pi}{4}\\x=\dfrac{\pi}{2}+k\pi\end{matrix}\right.\) (\(k\in Z\))



