Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Mấy này bạn quy đồng lên cùng mẫu xong khử mẫu rồi giải. Dễ mà.

a) ĐKXĐ: x # -5
\(\dfrac{2x-5}{x+5}=3\) ⇔ \(\dfrac{2x-5}{x+5}=\dfrac{3\left(x+5\right)}{x+5}\)
⇔ 2x - 5 = 3x + 15
⇔ 2x - 3x = 5 + 20
⇔ x = -20 thoả ĐKXĐ
Vậy tập hợp nghiệm S = {-20}
b) ĐKXĐ: x # 0
\(\dfrac{x^2-6}{x}=x+\dfrac{3}{2}\Leftrightarrow\dfrac{2\left(x^2+6\right)}{2x}=\dfrac{2x^2+3x}{2x}\)
Suy ra: 2x2 – 12 = 2x2 + 3x ⇔ 3x = -12 ⇔ x = -4 thoả x # 0
Vậy tập hợp nghiệm S = {-4}.
c) ĐKXĐ: x # 3
\(\dfrac{\left(x^2+2x\right)-\left(3x+6\right)}{x-3}=0\) ⇔ x(x + 2) - 3(x + 2) = 0
⇔ (x - 3)(x + 2) = 0 mà x # 3
⇔ x + 2 = 0
⇔ x = -2
Vậy tập hợp nghiệm S = {-2}
d) ĐKXĐ: x # \(-\dfrac{2}{3}\)
\(\dfrac{5}{3x+2}=2x-1\Leftrightarrow\dfrac{5}{3x+2}=\dfrac{\left(2x-1\right)\left(3x+2\right)}{3x+2}\)
⇔ 5 = (2x - 1)(3x + 2)
⇔ 6x2 – 3x + 4x – 2 – 5 = 0
⇔ 6x2 + x - 7 = 0
⇔ 6x2 - 6x + 7x - 7 = 0
⇔ 6x(x - 1) + 7(x - 1) = 0
⇔ (6x + 7)(x - 1) = 0
⇔ x = \(-\dfrac{7}{6}\) hoặc x = 1 thoả x # \(-\dfrac{2}{3}\)
Vậy tập nghiệm S = {1;\(-\dfrac{7}{6}\)}.
a)ĐKXĐ:x≠-5
Khử mẫu:2x-5=3(x+5) (1)
giải phương trình (1),ta được:
(1)⇔2x-5=3x+15
⇔2x-3x=15+5
⇔-x=20⇔x=-20(TM)
vậy phương trình đã cho có nghiệm x=-20

Câu 2:
ĐKXĐ: \(\left[{}\begin{matrix}1-9x^2\ne0\\1+3x\ne0\\1-3x\ne0\end{matrix}\right.\Rightarrow \left[{}\begin{matrix}x\ne\dfrac{-1}{3}\\x\ne\dfrac{1}{3}\end{matrix}\right.\)
\(\dfrac{12}{1-9x^2}=\dfrac{1-3x}{1+3x}-\dfrac{1+3x}{1-3x}\left(1\right)\)
\(\left(1\right):\dfrac{12}{\left(1-3x\right)\left(1+3x\right)}-\dfrac{\left(1-3x\right)\left(1-3x\right)}{\left(1-3x\right)\left(1+3x\right)}+\dfrac{\left(1+3x\right)\left(1+3x\right)}{\left(1-3x\right)\left(1+3x\right)}=0\)
\(\Leftrightarrow 12-\left(1-3x-3x+9x^2\right)+\left(1+3x+3x+9x^2\right)=0\)
\(\Leftrightarrow 12-1+3x+3x-9x^2+1+3x+3x+9x^2=0\)
\(\Leftrightarrow12x+12=0\\ \Leftrightarrow12x=-12\\ \Leftrightarrow x=-1\left(TM\right)\)
Vậy \(S=\left\{-1\right\}\)
giải các phương trình sau:
a) 6x-3= 4x+5
b) \(\dfrac{2x+3}{x+1}\)- \(\dfrac{6}{x}\)= 2
c) \(|3x-1|\)=3x

a)\(6x-3=4x+5\)
\(\Rightarrow6x-3-4x-5=0\)
\(\Rightarrow2x-8=0\)
\(\Rightarrow x=4\)
Vậy x=4
b)\(\frac{2x+3}{x+1}-\frac{6}{x}=2\left(ĐKXĐ:x\ne-1;0\right)\)
\(\Rightarrow\frac{2x^2+3x}{x\left(x+1\right)}-\frac{6x+6}{x\left(x+1\right)}=2\)
\(\Rightarrow\frac{2x^2+3x-6x-6}{x\left(x+1\right)}=2\)
\(\Rightarrow2x^2-3x-6=2\left(x^2+x\right)\)
\(\Rightarrow2x^2-3x-6-2x^2-2x=0\)
\(\Rightarrow-5x-6=0\)
\(\Rightarrow x=-\frac{6}{5}\)
Vậy \(x=-\frac{6}{5}\)
c)\(\left|3x-1\right|=3x\left(1\right)\)
TH1:\(x\ge\frac{1}{3}\).PT(1) có dạng:3x-1=3x
0x=1
PT vô nghiệm
TH2:\(x< \frac{1}{3}\).PT(1) có dạng:1-3x=3x
\(\Rightarrow6x=1\)
\(\Rightarrow x=\frac{1}{6}\left(TM\right)\)
Vậy PT có nghiệm là \(\frac{1}{6}\)
a, \(6x-3=4x+5 \)
\(\Leftrightarrow6x-4x=5+3\)
\(\Leftrightarrow2x=8\)
\(\Leftrightarrow x=4\)
vậy no của pt là : x = 4
b, \(\frac{2x+3}{x+1}-\frac{6}{x}=2\)
ĐKXĐ : \(\hept{\begin{cases}x\ne-1\\x\ne0\end{cases}}\)
\(\Leftrightarrow\frac{2x^2+3x-6x-6}{x\left(x+1\right)}=2\)
\(\Leftrightarrow\frac{2x^2-3x-6}{x\left(x+1\right)}=2\)
\(\Leftrightarrow2x^2-3x-6=2x^2+2x\)
\(\Leftrightarrow-5x=6\)
\(\Leftrightarrow x=\frac{-6}{5}\)
vậy no của pt là x=-6/5
c, \(\left|3x-1\right|=3x\)
Với \(3x-1\ge0\)
\(\Rightarrow3x-1=3x\Leftrightarrow-1=0\)( vô lí )

1) \(2\left(3x-1\right)-3x=10\)
<=> \(6x-2-3x=10\)
<=>\(3x-2=10\)
<=> \(3x=12\)
<=> \(x=4\)
Vậy tập nghiệm của pt S={4}
2) \(\dfrac{x+1}{x}+1=\dfrac{3x-1}{x+1}+\dfrac{1}{x\left(x+1\right)}\)
ĐKXĐ: x khác 0; x khác 1,-1
<=> \(\dfrac{\left(x+1\right)^2}{x\left(x+1\right)}+\dfrac{x\left(x+1\right)}{x\left(x+1\right)}\)= \(\dfrac{3x^2-x}{x\left(x+1\right)}+\dfrac{1}{x\left(x+1\right)}\)
=> \(\left(x+1\right)^2+x\left(x+1\right)\)= \(3x^2-x+1\)
<=> \(x^2+2x+1+x^2+x=3x^2-x+1\)
<=> \(x^2+x^2+2x+x-3x^2+x\)= \(1-1\)
<=> \(-x^2+4x=0\)
<=>\(4x=x^2\)
<=> \(4=x\) ( TMĐKXĐ)
Vậy tập nghiệm của pt S={4}
c) \(\dfrac{2x+1}{3}-\dfrac{3x-2}{2}>\dfrac{1}{6}\)
<=> \(\dfrac{4x+2}{6}-\dfrac{9x-6}{6}>\dfrac{1}{6}\)
<=> \(\dfrac{4x+2-9x+6}{6}-\dfrac{1}{6}>0\)
<=> \(\dfrac{-5x+7}{6}>0\)
Mà 6>0 . Nên \(-5x+7>0\)
Ta có \(-5x+7>0\)
<=> \(-5x>-7\)
<=> \(x< \dfrac{7}{5}\)
Vậy tập nghiệm của bất phương trình S={x thuộc R| \(x< \dfrac{7}{5}\)}
1)2.(3x-1)-3x=10
6x-2-3x =10
6x-3x =10+2
3x =12
x =4
Vậy S=4
2) \(\dfrac{x+1}{x}+1=\dfrac{3x-1}{x+1}+\dfrac{1}{x\left(x+1\right)}\)
Đkxđ: \(x\ne0\) và \(x\ne-1\)
MTC;x(x+1)
\(\dfrac{x+1}{x}+1=\dfrac{3x-1}{x+1}+\dfrac{1}{x\left(x+1\right)}\)
\(\Leftrightarrow\)\(\dfrac{\left(x+1\right)\left(x+1\right)+x\left(x+1\right)}{x\left(x+1\right)}=\dfrac{x\left(3x-1\right)+1}{x\left(x+1\right)}\)
\(\Leftrightarrow\)(x+1) (x+1)+x(x+1) = x (3x-1)+1
\(\Leftrightarrow\)x2+x+x+1+x2+x =3x2-x+1
\(\Leftrightarrow\)x2+x+x+1+x2+x-3x2+x-1=0
\(\Leftrightarrow\)-x24x=0
\(\Leftrightarrow\)4x-x2=0
\(\Leftrightarrow\)x(4-x)=0
\(\Leftrightarrow\)x=0 hoặc 4-x=0
\(\Leftrightarrow\)x=0 hoặc x =4
3)\(\dfrac{2x+1}{3}-\dfrac{3x-2}{2}>\dfrac{1}{6}\)
\(\Leftrightarrow\)\(\dfrac{2x+1}{3}6-\dfrac{3x-2}{2}6>\dfrac{1}{6}\)6
\(\Leftrightarrow\)2(2x+1)-3(3x-2)>1
\(\Leftrightarrow\)4x+2-9x+6>1
\(\Leftrightarrow\)4x-9x>1-2-6
\(\Leftrightarrow\)-5x>-7
\(\Leftrightarrow\)-5x.\(\dfrac{1}{-5}>-7.\dfrac{1}{-5}\)
\(\Leftrightarrow x>\dfrac{7}{5}\)

a: \(\Leftrightarrow5x-2+\left(2x-1\right)\left(1-x\right)=2-2x-2x^2-2x+6\)
\(\Leftrightarrow5x-2+2x-2x^2-1+x=-2x^2-4x+8\)
=>8x-3=-4x+8
=>-4x=11
hay x=-11/4
b: \(\Leftrightarrow\left(-2x+5\right)\left(3x-1\right)+3\left(x^2-1\right)=\left(x+2\right)\left(1-3x\right)\)
\(\Leftrightarrow-6x^2+2x+15x-5+3x^2-3=x-3x^2+2-6x\)
\(\Leftrightarrow17x-8=-5x+2\)
=>22x=10
hay x=5/11
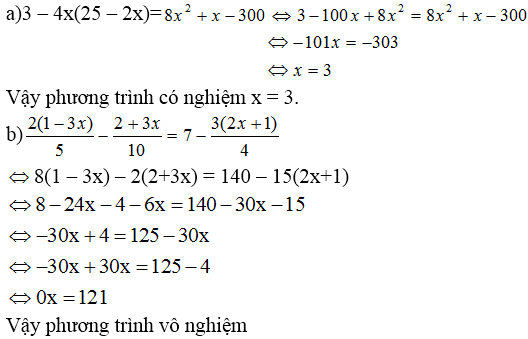



\(\Leftrightarrow3\left(3x+2\right)-3x-1=12x+10\)
=>9x+6-3x-1=12x+10
=>12x+10=3x+5
=>9x=-5
hay x=-5/9
\(9x+6-3x-1=12x+10\Leftrightarrow6x+5=12x+10\)
\(\Leftrightarrow6x=-5\Leftrightarrow x=-\dfrac{5}{6}\)