Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a, \(6x^2-5x+3=2x-3x\left(3-2x\right)\)
⇔ \(6x^2-5x+3=2x-9x+6x^2\)
⇔ \(6x^2-5x+3-6x^2+9x-2x=0\)
⇔ \(2x+3=0\)
⇔ \(2x=-3\)
⇔ \(x=-\dfrac{3}{2}\)
b, \(\dfrac{2\left(x-4\right)}{4}-\dfrac{3+2x}{10}=x+\dfrac{1-x}{5}\)
⇔ \(\dfrac{20\left(x-4\right)}{4.10}-\dfrac{4\left(3+2x\right)}{4.10}=\dfrac{5x}{5}+\dfrac{1-x}{5}\)
⇔ \(\dfrac{20x-80}{40}-\dfrac{12+8x}{40}=\dfrac{5x+1-x}{5}\)
⇔ \(\dfrac{20x-80-12-8x}{40}=\dfrac{4x+1}{5}\)
⇔ \(\dfrac{12x-92}{40}-\dfrac{4x+1}{5}=0\)
⇔ \(\dfrac{12x-92}{40}-\dfrac{8\left(4x+1\right)}{40}=0\)
⇔ \(12x-92-8\left(4x+1\right)=0\)
⇔ 12x - 92 - 32x - 8 = 0
⇔ -100 - 20x = 0
⇔ 20x = -100
⇔ x = -100 : 20
⇔ x = -5

Bạn Kim Tuyến làm sai rùi , mk sửa lại :
a) 4x2 - 4x + 1 > 9
⇔ 4x2 - 4x - 8 > 0
⇔4x2 + 4x - 8x - 8 > 0
⇔ 4x( x + 1) -8( x + 1) > 0
⇔ ( x + 1)( 4x - 8) > 0
⇔ ( x + 1)( x - 2) > 0
Lập bảng xét dấu , ta có :
x x+1 x-2 -1 2 0 0 - + + - - + Tích số + - + 0 0 Vậy, nghiệm của BPT : x < -1 hoặc : x > 2
b) ( x - 5)( 7 - 2x ) < 0
Lập bảng xét dấu :
x x-5 7-2x tích số 7/2 5 0 0 0 0 - - + + - - - + - Vậy , nghiệm của BPT : x < 7/2 hoặc x > 5

a) 1x−1−3x2x3−1=2xx2+x+11x−1−3x2x3−1=2xx2+x+1
Ta có: x3−1=(x−1)(x2+x+1)x3−1=(x−1)(x2+x+1)
=(x−1)[(x+12)2+34]=(x−1)[(x+12)2+34] cho nên x3 – 1 ≠ 0 khi x – 1 ≠ 0⇔ x ≠ 1
Vậy ĐKXĐ: x ≠ 1
Khử mẫu ta được:
x2+x+1−3x2=2x(x−1)⇔−2x2+x+1=2x2−2xx2+x+1−3x2=2x(x−1)⇔−2x2+x+1=2x2−2x
⇔4x2−3x−1=0⇔4x2−3x−1=0
⇔4x(x−1

a) 4x -8 ≥ 3(3x-1)-2x +1
⇒4x -8 ≥7x -2
⇒4x -7x ≥ -2 +8
⇒-3x ≥ 6
⇒x≤-2
Vậy bpt có nghiệm là:{x|x≤-2}
b) (x-3)(x+2)+(x+4)2≤ 2x (x+5)+4
⇔ x2+2x - 3x - 6 +x2 + 8x +16≤ 2x2 + 10x +4
⇔ x2 +2x - 3x + x2 + 8x - 2x2- 10x ≤ 4+6-16
⇔ -3x ≤ -6
⇔ x≥ 2
Vậy bpt có tập nghiệm là: {x|x≥2}



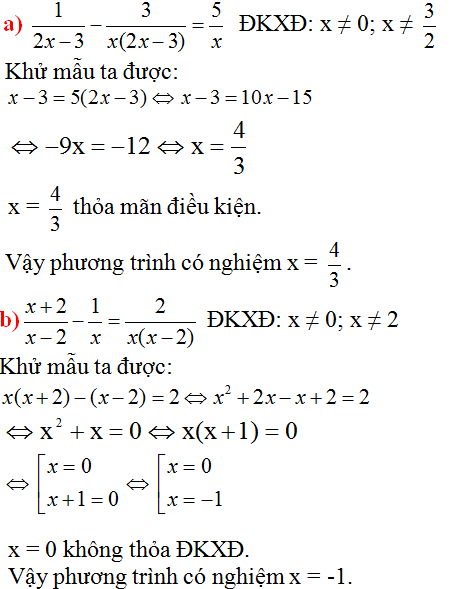


a) \(\left|x-1\right|+\left|x-2\right|>x+3\)
ta có các trường hợp
trường hợp 1:\(\left|x-1\right|< 0\Leftrightarrow\left|x-2\right|< 0\Leftrightarrow\left\{{}\begin{matrix}\left|x-1\right|=-x+1\\\left|x-2\right|=-x+2\end{matrix}\right.\Leftrightarrow x< 1\)
trường hợp 2: \(\left|x-1\right|\ge0và\left|x-2\right|< 0\Leftrightarrow\left\{{}\begin{matrix}\left|x-1\right|=x-1\\\left|x-2\right|=-x+2\end{matrix}\right.\Leftrightarrow1\le x< 2\)
trường hợp 3:\(\left|x-2\right|\ge0\Leftrightarrow\left|x-1\right|>0\Leftrightarrow\left\{{}\begin{matrix}\left|x-2\right|=x-2\\\left|x-1\right|=x-1\end{matrix}\right.\Leftrightarrow x\ge2\)\(\) \(\)
xét trường hợp 1:ta có BPT:
\(-x+1-x+2>x+3\Leftrightarrow-x-x-x>-1-2+3\\ \Leftrightarrow-3x>0\Leftrightarrow x< 0\)
vì điều kiện là x<1 nên mọi giá trị của x<0 đều thỏa mãn
trường hợp 2:
\(x-1-x+2>x+3\Leftrightarrow x-x-x>1-2+3\\ \Leftrightarrow-x>2\Leftrightarrow x< -2\)
vì điều kiện là \(1\le x< 2\) nên không có giá trị nào của x TM
trường hợp 3:
\(x-1+x-2>x+3\Leftrightarrow x+x-x>1+2+3\\ \Leftrightarrow x>6\)
vì điều kiện là x>=2 nên với mọi giá trị x>6 đều TM
Vậy nghiệm BPT là: x<0 hoặc x>6
c)
\(\left(x+5\right)\left(7-2x\right)>0\\ \Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x+5>0\\7-2x>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>-5\\-2x>-7\Leftrightarrow x< \dfrac{7}{2}\end{matrix}\right.\Leftrightarrow-5< x< \dfrac{7}{2}\\\left\{{}\begin{matrix}x+5< 0\\7-2x< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x< -5\\-2x< -7\Leftrightarrow x>\dfrac{7}{2}\end{matrix}\right.\end{matrix}\right.\)
Vì trường hợp 2 không có giá trị nào của x TM nên ta loại
Vậy tập nghiệm của BPT là {x/5<x<7/2}