Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Lời giải:
a)
\(3x^2-5x+1=2x-3\)
\(\Leftrightarrow 3x^2-5x+1-2x+3=0\)
\(\Leftrightarrow 3x^2-7x+4=0\) (\(a=3; b=-7; c=4)\)
b)
\(\frac{3}{5}x^2-4x-3=3x+\frac{1}{3}\)
\(\Leftrightarrow \frac{3}{5}x^2-4x-3-3x-\frac{1}{3}=0\)
\(\Leftrightarrow \frac{3}{5}x^2-7x-\frac{10}{3}=0(a=\frac{3}{5};b=-7; c=\frac{-10}{3})\)
c)
\(\Leftrightarrow -\sqrt{3}x^2+x-5-\sqrt{3}x-\sqrt{2}=0\)
\(\Leftrightarrow -\sqrt{3}x^2+(1-\sqrt{3})x-(5+\sqrt{2})=0\)
(\(a=-\sqrt{3}; b=1-\sqrt{3}; c=-(5+\sqrt{2}))\)
d)
\(\Leftrightarrow x^2-5(m+1)x+m^2-2=0\)
(\(a=1;b=-5(m+1); c=m^2-2)\)

a: \(\Leftrightarrow4x^2-3x+7=0\)
a=4; b=-3; c=7
b: \(\Leftrightarrow\sqrt{5}x^2-x^2+5x-3-3x+4=0\)
\(\Leftrightarrow x^2\cdot\left(\sqrt{5}-1\right)+2x+1=0\)
\(a=\sqrt{5}-1;b=2;c=1\)
c: \(\Leftrightarrow mx^2-x^2-3x+mx+5=0\)
\(\Leftrightarrow x^2\left(m-1\right)+x\left(m-3\right)+5=0\)
a=m-1; b=m-3; c=5
d: \(\Leftrightarrow m^2x^2-x^2+x+m-mx-m-2=0\)
\(\Leftrightarrow x^2\left(m^2-1\right)+x\left(1-m\right)-2=0\)
\(a=m^2-1;b=1-m;c=-2\)

1) Đk: x khác -3
x khác 1
Biểu thức \(\Leftrightarrow\dfrac{x^2-x}{x^2+2x-3}+\dfrac{2x+6}{x^2+2x-3}=\dfrac{12}{x^2+2x-3}\)
\(\Leftrightarrow x^2-x+2x+6=12\Leftrightarrow x^2+x-6=0\Leftrightarrow\left(x-2\right)\left(x+3\right)=0\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-3\end{matrix}\right.\)
kl: x thuộc {-3;2}

Bài giải:
a) 3x2 – 2x = x2 + 3 ⇔ 2x2 – 2x - 3 = 0.
b’ = -1, ∆’ = (-1)2 – 2 . (-3) = 7
x1 = ≈ 1, 82; x2 =
≈ -0,82
b) (2x - √2)2 – 1 = (x + 1)(x – 1) ⇔ 3x2 - 4√2 . x + 2 = 0 . b’ = -2√2
∆’ = (-2√2)2 – 3 . 2 = 2
x1 = = √2 ≈ 1,41; x2 =
=
≈ 0,47.
c) 3x2 + 3 = 2(x + 1) ⇔ 3x2 – 2x + 1 = 0.
b’ = -1; ∆’ = (-1)2 – 3 . 1 = -2 < 0
Phương trình vô nghiệm.
d) 0,5x(x + 1) = (x – 1)2 ⇔ 0,5x2 – 2,5x + 1 = 0
⇔ x2 – 5x + 2 = 0, b’ = -2,5; ∆’ = (-2,5)2 – 1 . 2 = 4,25
x1 = 2,5 + √4,25 ≈ 4,56, x2 = 2,5 - √4,25 ≈ 0,44
(Rõ ràng trong trường hợp này dung công thức nghiệm thu gọn cũng không đơn giản hơn)
a) 3x2 – 2x = x2 + 3 ⇔ 2x2 – 2x - 3 = 0.
b’ = -1, ∆’ = (-1)2 – 2 . (-3) = 7
x1 = ≈ 1, 82; x2 =
≈ -0,82
b) (2x - √2)2 – 1 = (x + 1)(x – 1) ⇔ 3x2 - 4√2 . x + 2 = 0 . b’ = -2√2
∆’ = (-2√2)2 – 3 . 2 = 2
x1 = = √2 ≈ 1,41; x2 =
=
≈ 0,47.
c) 3x2 + 3 = 2(x + 1) ⇔ 3x2 – 2x + 1 = 0.
b’ = -1; ∆’ = (-1)2 – 3 . 1 = -2 < 0
Phương trình vô nghiệm.
d) 0,5x(x + 1) = (x – 1)2 ⇔ 0,5x2 – 2,5x + 1 = 0
⇔ x2 – 5x + 2 = 0, b’ = -2,5; ∆’ = (-2,5)2 – 1 . 2 = 4,25
x1 = 2,5 + √4,25 ≈ 4,56, x2 = 2,5 - √4,25 ≈ 0,44

c: =>3x^2+3y^2=39 và 3x^2-2y^2=-6
=>5y^2=45 và x^2=13-y^2
=>y^2=9 và x^2=4
=>\(\left\{{}\begin{matrix}x\in\left\{2;-2\right\}\\y\in\left\{3;-3\right\}\end{matrix}\right.\)
d: \(\Leftrightarrow\left\{{}\begin{matrix}5\sqrt{x}=5\\\sqrt{x}-\sqrt{y}=-\dfrac{11}{2}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\\sqrt{y}=1+\dfrac{11}{2}=\dfrac{13}{2}\end{matrix}\right.\)
=>x=1 và y=169/4
b: \(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{3x+3-3}{x+1}-\dfrac{2}{y+4}=4\\\dfrac{2x+2-2}{x+1}-\dfrac{5}{y+4}=9\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-\dfrac{3}{x+1}-\dfrac{2}{y+4}=4-3=1\\-\dfrac{2}{x+1}-\dfrac{5}{y+4}=9-2=7\end{matrix}\right.\)
=>x+1=11/9 và y+4=-11/19
=>x=2/9 và y=-87/19

\(\sqrt{x+4\sqrt{x-4}}+\sqrt{x-4\sqrt{x-4}}=m\)
\(\Leftrightarrow\sqrt{\left(\sqrt{x-4}+2\right)^2}+\sqrt{\left(2-\sqrt{x-4}\right)^2}=m\)
\(\Leftrightarrow\left|\sqrt{x-4}+2\right|+\left|2-\sqrt{x-4}\right|=m\)
mà \(\left|\sqrt{x-4}+2\right|+\left|2-\sqrt{x-4}\right|\)
\(\ge\left|\sqrt{x-4}+2+2-\sqrt{x-4}\right|=4\)
\(\Rightarrow m\ge4\) thì pt trên có no
a) 5x2 + 2x = 4 – x ⇔ 5x2 + 3x – 4 = 0; a = 5, b = 3, c = -4
b) x2 + 2x – 7 = 3x +
x2 + 2x – 7 = 3x +  ⇔
⇔  x2 – x -
x2 – x - 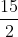 = 0, a =
= 0, a =  , b = -1, c = -
, b = -1, c = -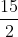
c) 2x2 + x - √3 = √3 . x + 1 ⇔ 2x2 + (1 - √3)x – 1 - √3 = 0
Với a = 2, b = 1 - √3, c = -1 - √3
d) 2x2 + m2 = 2(m – 1)x ⇔ 2x2 - 2(m – 1)x + m2 = 0; a = 2, b = - 2(m – 1), c = m2