
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bạn sử dụng cong thức soạn thảo như người khác thì có sao đâu?
1. Không biết yêu cầu đề bài là gì???
2. Biểu thức đề bài ko rõ ràng (ko biết căn thức tới đâu, đâu là tử số đâu là mẫu số).
Bạn cần ghi rõ yêu cầu đề bài, và sử dụng công cụ gõ công thức (kí hiệu khoanh đỏ trên khung soạn thảo) để mọi người đỡ mệt.

Hàm là vậy phải không nhỉ? \(y=\dfrac{sin^2x-3sinx}{\left(tanx-1\right)\left(cotx+1\right)}\)
ĐKXĐ: \(\left\{{}\begin{matrix}sinx\ne0\\cosx\ne0\\tanx-1\ne0\\cotx+1\ne0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}sin2x\ne0\\tanx\ne1\\cotx\ne-1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ne\dfrac{k\pi}{2}\\x\ne\dfrac{\pi}{4}+k\pi\\x\ne-\dfrac{\pi}{4}+k\pi\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x\ne\dfrac{k\pi}{2}\\x\ne\dfrac{\pi}{4}+\dfrac{k\pi}{2}\end{matrix}\right.\)
\(\Leftrightarrow x\ne\dfrac{k\pi}{4}\)

\(\frac{2.sinx}{cosx}+\frac{cosx}{sinx}-4sinx.cosx-\frac{1}{2sinx.cosx}=0\) (Điều kiện \(x\ne\frac{k\pi}{2}\))
\(\Leftrightarrow\frac{4sin^2x+2cos^2x-8sin^2x.cos^2x-1}{2sinx.cosx}=0\)
\(\Leftrightarrow2sin^2x-8sin^2x.cos^2x+2\left(sin^2x+cos^2x\right)-1=0\)
\(\Leftrightarrow2sin^2x-8sin^2x.\left(1-sin^2x\right)+2-1=0\)
\(\Leftrightarrow8sin^4x-6sin^2x+1=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sin^2x=\frac{1}{4}\\sin^2x=\frac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\frac{1-cos2x}{2}=\frac{1}{4}\\\frac{1-cos2x}{2}=\frac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=\frac{1}{2}=cos\frac{\pi}{3}\\cos2x=0=cos\frac{\pi}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=\frac{\pi}{2}+k\pi\\2x=\frac{\pi}{3}+k2\pi\\2x=-\frac{\pi}{3}+k2\pi\end{matrix}\right.\left(k\in Z\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{4}+\frac{k\pi}{4}\\x=\frac{\pi}{6}+k\pi\\x=-\frac{\pi}{6}+k\pi\end{matrix}\right.\left(k\in Z\right)\)
giao với điều kiện \(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{4}+\frac{k\pi}{2}\\x=\frac{\pi}{6}+k\pi\\x=-\frac{\pi}{6}+k\pi\end{matrix}\right.\left(k\in Z\right)\)

\(tan\left(\dfrac{x}{2}\right)=\sqrt{3}\)
\(\Leftrightarrow\dfrac{x}{2}=\dfrac{\pi}{3}+k\pi\)
\(\Leftrightarrow x=\dfrac{2\pi}{3}+k2\pi\) (\(k\in Z\))

\(\dfrac{tanx+1}{tanx-1}=\dfrac{1+cotx}{1-cotx}\)
=>(tanx+1)(1-cotx)=(1+cotx)(tan x-1)
=>tan x-1+1-cot x=tan x-1+1-cot x
=>tan x-cot x=tan x-cot x(luôn đúng)
=>ĐPCM

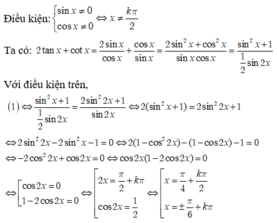
Đề??
ĐK: \(x\ne\dfrac{k\pi}{2}\)
\(cotx=tanx+2tanx\)
\(\Leftrightarrow cotx=3tanx\)
\(\Leftrightarrow\dfrac{1}{tanx}=3tanx\)
\(\Leftrightarrow tan^2x=\dfrac{1}{3}\)
\(\Leftrightarrow\left[{}\begin{matrix}tanx=\dfrac{1}{3}\\tanx=-\dfrac{1}{3}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=arctan\dfrac{1}{3}+k\pi\\x=arctan\left(-\dfrac{1}{3}\right)+k\pi\end{matrix}\right.\)
\(\left[{}\begin{matrix}tanx=\dfrac{\sqrt{3}}{3}\\tanx=-\dfrac{\sqrt{3}}{3}\end{matrix}\right.\) ?