Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Lời giải:
a) Ta có f'(x) = 3x2 + 1, g(x) = 6x + 1. Do đó
f'(x) > g'(x) <=> 3x2 + 1 > 6x + 1 <=> 3x2 - 6x >0
<=> 3x(x - 2) > 0 <=> x > 2 hoặc x > 0 <=> x ∈ (-∞;0) ∪ (2;+∞).
b) Ta có f'(x) = 6x2 - 2x, g'(x) = 3x2 + x. Do đó
f'(x) > g'(x) <=> 6x2 - 2x > 3x2 + x <=> 3x2 - 3x > 0
<=> 3x(x - 1) > 0 <=> x > 1 hoặc x < 0 <=> x ∈ (-∞;0) ∪ (1;+∞).

\(C'=0\) với mọi hằng số C
nguyen thi khanh nguyen
\(f'\left(x\right)=6x^2-2x\)
\(g'\left(x\right)=3x^2+x\)
\(f'\left(x\right)>g'\left(x\right)\Leftrightarrow6x^2-2x>3x^2+x\)
\(\Leftrightarrow3x^2-3x>0\Rightarrow\left[{}\begin{matrix}x>1\\x< 0\end{matrix}\right.\)

a: \(-1< =cosx< =1\)
\(\Leftrightarrow-2< =2cosx< =2\)
\(\Leftrightarrow-5< =2cosx-3< =-1\)
\(f\left(x\right)_{min}=-5\) khi cos x=-1
hay \(x=\Pi+k2\Pi\)
\(f\left(x\right)_{max}=-1\) khi cos x=1
hay \(x=k2\Pi\)
b: \(-1< =sinx< =1\)
\(\Leftrightarrow-2< =2sinx< =2\)
\(\Leftrightarrow5< =2sinx+7< =9\)
\(\Leftrightarrow\sqrt{5}< =\sqrt{2sinx+7}< =3\)
\(\Leftrightarrow3\sqrt{5}< =3\sqrt{2sinx+7}< =9\)
\(f\left(x\right)_{min}=3\sqrt{5}\) khi sin x=-1
hay \(x=-\dfrac{\Pi}{2}+k2\Pi\)
\(f\left(x\right)_{max}=9\) khi sin x=1
hay \(x=\dfrac{\Pi}{2}+k2\Pi\)

Tại mọi điểm \(x\ne1\) hàm đã cho là hàm đa thức nên liên tục
Xét tại \(x=1\)
\(\lim\limits_{x\rightarrow1^-}f\left(x\right)=\lim\limits_{x\rightarrow1^-}\left(3x+2\right)=5\)
\(\lim\limits_{x\rightarrow1^+}f\left(x\right)=\lim\limits_{x\rightarrow1^+}\left(x^2-1\right)=0\)
Do \(\lim\limits_{x\rightarrow1^+}f\left(x\right)\ne\lim\limits_{x\rightarrow1^-}f\left(x\right)\) nên hàm số đã cho ko liên tục tại \(x=1\)
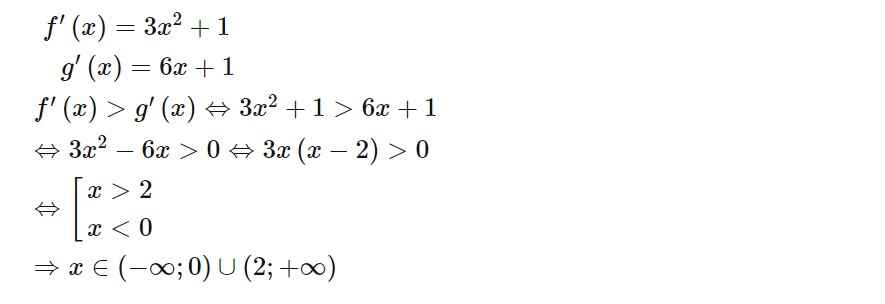
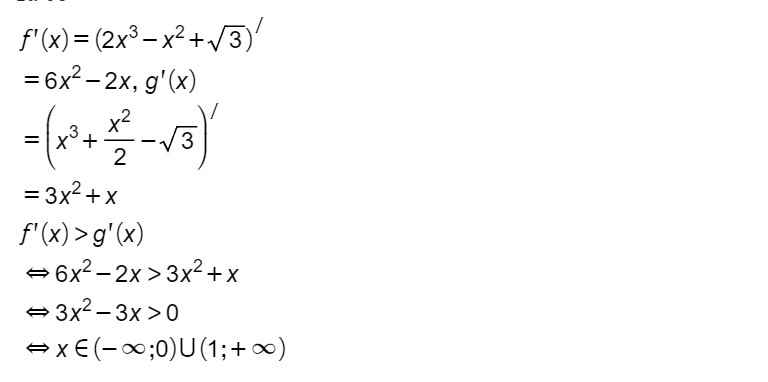
\(f'\left(x\right)=\dfrac{1-x}{\sqrt{2x-x^2}}\)
\(f'\left(x\right)\ge1\Leftrightarrow\dfrac{1-x}{\sqrt{2x-x^2}}\ge1\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x-x^2>0\\1-x>0\\\left(1-x\right)^2\ge2x-x^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}0< x< 2\\x< 1\\2x^2-4x+1\ge0\end{matrix}\right.\) \(\Rightarrow0< x\le\dfrac{2-\sqrt{2}}{2}\)
f'(x)=\(\dfrac{2-2x}{2\sqrt{2x-x^2}}\) = \(\dfrac{1-x}{\sqrt{2x-x^2}}\)
để f'(x) \(\ge\) 1 \(\Leftrightarrow\) \(\dfrac{1-x}{\sqrt{2x-x^2}}\) \(\ge\) 1 \(\Leftrightarrow\) 1-x \(\ge\) \(\sqrt{2x-x^2}\)
\(\Leftrightarrow\) \(\left\{{}\begin{matrix}2x-x^2>0\\1-2x+x^2\ge2x-x^2\end{matrix}\right.\) \(\Rightarrow\) \(\left\{{}\begin{matrix}0< x< 2\\\left\{{}\begin{matrix}x< \dfrac{2-\sqrt{2}}{2}\\x>\dfrac{2+\sqrt{2}}{2}\end{matrix}\right.\end{matrix}\right.\)\(\Rightarrow\) 0<x\(\le\) \(\dfrac{2-\sqrt{2}}{2}\)