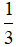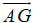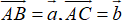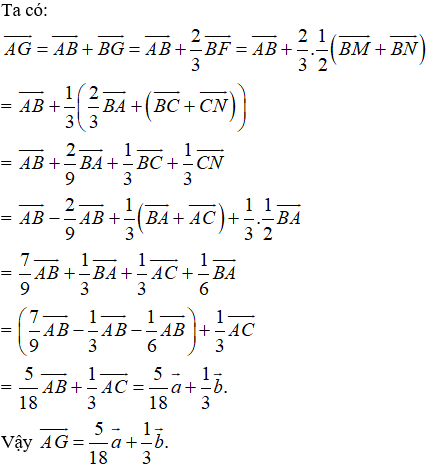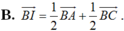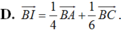Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a, Ta có:AM+AN=OM-OA+ON-OA=OM+ON+AC=OC+AC=3/2OC
GA+3GB+GC+OD=2GB+OD=OB+OD=0
C,

Cách 1:
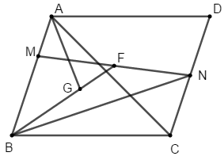
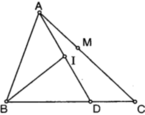
Gọi O là giao điểm của AC và BD.

Ta có:
\(\begin{array}{l}\overrightarrow {AG} = \overrightarrow {AB} + \overrightarrow {BG} = \overrightarrow a + \overrightarrow {BG} ;\\\overrightarrow {CG} = \overrightarrow {CB} + \overrightarrow {BG} = \overrightarrow {DA} + \overrightarrow {BG} = - \overrightarrow b + \overrightarrow {BG} ;\end{array}\)(*)
Lại có: \(\overrightarrow {BD} =\overrightarrow {BA} + \overrightarrow {AD} = - \overrightarrow a + \overrightarrow b \).
\(\overrightarrow {BG} ,\overrightarrow {BD} \) cùng phương và \(\left| {\overrightarrow {BG} } \right| = \frac{2}{3}BO = \frac{1}{3}\left| {\overrightarrow {BD} } \right|\)
\( \Rightarrow \overrightarrow {BG} = \frac{1}{3}\overrightarrow {BD} = \frac{1}{3}\left( { - \overrightarrow a + \overrightarrow b } \right)\)
Do đó (*) \( \Leftrightarrow \left\{ \begin{array}{l}\overrightarrow {AG} = \overrightarrow a + \overrightarrow {BG} = \overrightarrow a + \frac{1}{3}\left( { - \overrightarrow a + \overrightarrow b } \right) = \frac{2}{3}\overrightarrow a + \frac{1}{3}\overrightarrow b ;\\\overrightarrow {CG} = -\overrightarrow b + \overrightarrow {BG} = -\overrightarrow b + \frac{1}{3}\left( { - \overrightarrow a + \overrightarrow b } \right) = - \frac{1}{3}\overrightarrow a - \frac{2}{3}\overrightarrow b ;\end{array} \right.\)
Vậy \(\overrightarrow {AG} = \frac{2}{3}\overrightarrow a + \frac{1}{3}\overrightarrow b ;\;\overrightarrow {CG} = - \frac{1}{3}\overrightarrow a - \frac{2}{3}\overrightarrow b .\)
Cách 2:
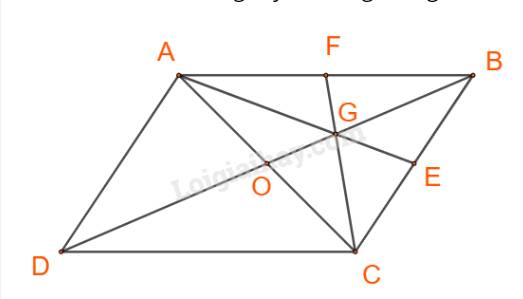
Gọi AE, CF là các trung tuyến trong tam giác ABC.
Ta có:
\(\overrightarrow {AG} = \frac{2}{3}\overrightarrow {AE} = \frac{2}{3}.\frac{1}{2}\left( {\overrightarrow {AB} + \overrightarrow {AC} } \right) = \frac{2}{3}.\frac{1}{2}\left[ {\overrightarrow {AB} + \left( {\overrightarrow {AB} + \overrightarrow {AD} } \right)} \right] \\= \frac{1}{3}\left( {2\overrightarrow a + \overrightarrow b } \right) = \frac{2}{3}\overrightarrow a + \frac{1}{3}\overrightarrow b \)
\(\overrightarrow {CG} = \frac{2}{3}\overrightarrow {CF} = \frac{2}{3}.\frac{1}{2}\left( {\overrightarrow {CA} + \overrightarrow {CB} } \right) = \frac{2}{3}.\frac{1}{2}\left[ {\left( {\overrightarrow {CB} + \overrightarrow {CD} } \right) + \overrightarrow {CB} } \right] = \frac{1}{3}\left( {2\overrightarrow {CB} + \overrightarrow {CD} } \right) = \frac{1}{3}\left( { - 2\overrightarrow {AD} - \overrightarrow {AB} } \right) = - \frac{1}{3}\overrightarrow a - \frac{2}{3}\overrightarrow b \)
Vậy \(\overrightarrow {AG} = \frac{2}{3}\overrightarrow a + \frac{1}{3}\overrightarrow b ;\;\overrightarrow {CG} = - \frac{1}{3}\overrightarrow a - \frac{2}{3}\overrightarrow b .\)

1.
\(\left\{{}\begin{matrix}\overrightarrow{BA}+\overrightarrow{BC}=2\overrightarrow{BN}\\\overrightarrow{CA}+\overrightarrow{CB}=2\overrightarrow{CP}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-\overrightarrow{AB}+\overrightarrow{BC}=2\overrightarrow{BN}\\\overrightarrow{CB}+\overrightarrow{BA}+\overrightarrow{CB}=2\overrightarrow{CP}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\overrightarrow{AB}-\overrightarrow{BC}=-2\overrightarrow{BN}\\\overrightarrow{AB}+2\overrightarrow{BC}=-2\overrightarrow{CP}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2\overrightarrow{AB}-2\overrightarrow{BC}=-4\overrightarrow{BN}\\\overrightarrow{AB}+2\overrightarrow{BC}=-2\overrightarrow{CP}\end{matrix}\right.\)
\(\Rightarrow3\overrightarrow{AB}=-4\overrightarrow{BN}-2\overrightarrow{CP}\Rightarrow\overrightarrow{AB}=-\frac{4}{3}\overrightarrow{BN}-\frac{2}{3}\overrightarrow{CP}\)
2.
\(\overrightarrow{BI}=\overrightarrow{BA}+\overrightarrow{AD}+\overrightarrow{DI}\)
\(=-\overrightarrow{AB}+\overrightarrow{AD}+\frac{1}{2}\overrightarrow{DC}\)
\(=-\overrightarrow{AB}+\overrightarrow{AD}+\frac{1}{2}\overrightarrow{AB}\)
\(\Rightarrow\overrightarrow{BI}=-\frac{1}{2}\overrightarrow{AB}+\overrightarrow{AD}\)
\(\overrightarrow{AG}=\overrightarrow{AB}+\overrightarrow{BG}=\overrightarrow{AB}+\frac{1}{3}\left(\overrightarrow{BI}+\overrightarrow{BC}\right)\)
\(=\overrightarrow{AB}+\frac{1}{3}\left(-\frac{1}{2}\overrightarrow{AB}+\overrightarrow{AD}+\overrightarrow{AD}\right)\)
\(=\overrightarrow{AB}-\frac{1}{6}\overrightarrow{AB}+\frac{2}{3}\overrightarrow{AD}\)
\(\Rightarrow\overrightarrow{AG}=\frac{5}{6}\overrightarrow{AB}+\frac{2}{3}\overrightarrow{AD}\)

\(\overrightarrow{AD}+2\overrightarrow{AB}=\overrightarrow{AD}+\overrightarrow{AB}+\overrightarrow{AB}=\overrightarrow{AC}+\overrightarrow{AB}=2\overrightarrow{AI}\) (đpcm)