Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Câu 2:
\(f'\left(x\right)=\frac{-3}{\left(2x-1\right)^2}\)
a/ \(x_0=-1\Rightarrow\left\{{}\begin{matrix}f'\left(x_0\right)=-\frac{1}{3}\\f\left(x_0\right)=0\end{matrix}\right.\)
Pttt: \(y=-\frac{1}{3}\left(x+1\right)=-\frac{1}{3}x-\frac{1}{3}\)
b/ \(y_0=1\Rightarrow\frac{x_0+1}{2x_0-1}=1\Leftrightarrow x_0+1=2x_0-1\Rightarrow x_0=2\)
\(\Rightarrow f'\left(x_0\right)=-\frac{1}{3}\)
Pttt: \(y=-\frac{1}{3}\left(x-2\right)+1\)
c/ \(x_0=0\Rightarrow\left\{{}\begin{matrix}f'\left(x_0\right)=-3\\y_0=-1\end{matrix}\right.\)
Pttt: \(y=-3x-1\)
d/ \(6x+2y-1=0\Leftrightarrow y=-3x+\frac{1}{2}\)
Tiếp tuyến song song d \(\Rightarrow\) có hệ số góc bằng -3
\(\Rightarrow\frac{-3}{\left(2x_0-1\right)^2}=-3\Rightarrow\left(2x_0-1\right)^2=1\Rightarrow\left[{}\begin{matrix}x_0=0\Rightarrow y_0=-1\\x_0=1\Rightarrow y_0=2\end{matrix}\right.\)
Có 2 tiếp tuyến thỏa mãn: \(\left[{}\begin{matrix}y=-3x-1\\y=-3\left(x-1\right)+2\end{matrix}\right.\)
Làm câu 1,3 trước, câu 2 hơi dài tối rảnh làm sau:
1/ \(\lim\limits\frac{n^2+2n+1}{2n^2-1}=lim\frac{1+\frac{2}{n}+\frac{1}{n^2}}{2-\frac{1}{n^2}}=\frac{1}{2}\)
\(\lim\limits_{x\rightarrow0}\frac{2\sqrt{x+1}-x^2+2x+2}{x}=\frac{2-0+0+2}{0}=\frac{4}{0}=+\infty\)
Chắc bạn ghi nhầm đề, câu này biểu thức tử số là \(...-x^2+2x-2\) thì hợp lý hơn
3/ \(y'=2sin2x.\left(sin2x\right)'=4sin2x.cos2x=2sin4x\)
b/ \(y'=4x^3-4x\)
c/ \(y'=\frac{3\left(x+2\right)-1\left(3x-1\right)}{\left(x+2\right)^2}=\frac{7}{\left(x+2\right)^2}\)
d/ \(y'=10\left(x^2+x+1\right)^9\left(x^2+x+1\right)'=10\left(x^2+x+1\right)^9.\left(2x+1\right)\)
e/ \(y'=\frac{\left(2x^2-x+3\right)'}{2\sqrt{2x^2-x+3}}=\frac{4x-1}{2\sqrt{2x^2-x+3}}\)

Điều kiện: \(x\ne1\)
a) Xét phương trình: \(\frac{x^2-2mx+3m-2}{x-1}=0\Leftrightarrow x^2-2mx+3m-2=0\)\(\left(x-1\ne0\right)\)
Pt có hai nghiệm phân biệt \(\Leftrightarrow\Delta'>0\Leftrightarrow m^2-3m+2>0\Leftrightarrow\orbr{\begin{cases}m>2\\m< 1\end{cases}}\)
Khi đó \(\hept{\begin{cases}x_1=m-\sqrt{m^2-3m+2}\\x_2=m+\sqrt{m^2-3m+2}\end{cases}}\)
+) \(x_1,x_2\ne1\Leftrightarrow\hept{\begin{cases}m-\sqrt{m^2-3m+2}\ne1\\m+\sqrt{m^2-3m+2}\ne1\end{cases}\Leftrightarrow m\ne1}\)
+) Tiếp tuyến của đồ thị tại hai giao điểm với trục Ox vuông góc với nhau
\(\Leftrightarrow\hept{\begin{cases}y'\left(x_1\right)=-1\left(1\right)\\y'\left(x_2\right)=1\left(2\right)\end{cases}}\)
\(\left(1\right)\Leftrightarrow\frac{\left(2x_1-2m\right)\left(x_1-1\right)-\left(x_1^2-2mx_1+3m-2\right)}{\left(x_1-1\right)^2}=-1\)
\(\Leftrightarrow\frac{m-1}{\left(x_1-1\right)^2}=2\Rightarrow m-1=2\left(m-\sqrt{m^2-3m+2}-1\right)^2\)
\(\Leftrightarrow\left(m-1\right)\left[1-2\left(2m-3-2\sqrt{m^2-3m+2}\right)\right]=0\)
\(\Leftrightarrow4\sqrt{m^2-3m+2}=4m-7\Leftrightarrow\hept{\begin{cases}m\ge\frac{7}{4}\\m=\frac{17}{8}\end{cases}}\Leftrightarrow m=\frac{17}{8}\)(t/m m>2 v m<1)
Giải (2) cho ra \(m=1\)(loại). Vậy m cần tìm là \(m=\frac{17}{8}.\)

\(y=\dfrac{2x+2}{x-1}\Rightarrow y'=\dfrac{-4}{\left(x-1\right)^2}\)
a. \(y'\left(2\right)=-4\)
Phương trình tiếp tuyến: \(y=-4\left(x-2\right)+4\Leftrightarrow y=-4x+12\)
b. Pt hoành độ giao điểm:
\(\dfrac{2x+2}{x-1}=2x-1\Leftrightarrow2x^2-5x-1=0\Rightarrow\left[{}\begin{matrix}x=\dfrac{5-\sqrt{33}}{4}\\x=\dfrac{5+\sqrt{33}}{4}\end{matrix}\right.\)
\(y'\left(\dfrac{5-\sqrt{33}}{4}\right)=-\dfrac{17+\sqrt{33}}{8}\) ; \(y'\left(\dfrac{5+\sqrt{33}}{4}\right)=\dfrac{-17+\sqrt{33}}{8}\)
\(y\left(\dfrac{5-\sqrt{33}}{4}\right)=\dfrac{3-\sqrt{33}}{2}\) ; \(y\left(\dfrac{5+\sqrt{33}}{4}\right)=\dfrac{3+\sqrt{33}}{2}\)
Có 2 tiếp tuyến thỏa mãn:
\(\left[{}\begin{matrix}y=\dfrac{-17-\sqrt{33}}{8}\left(x-\dfrac{5-\sqrt{33}}{4}\right)+\dfrac{3-\sqrt{33}}{2}\\y=\dfrac{-17+\sqrt{33}}{8}\left(x-\dfrac{5+\sqrt{33}}{4}\right)+\dfrac{3+\sqrt{33}}{2}\end{matrix}\right.\)
Đề bài cho số liệu thật kì quặc
Đáp án D.
- Ta có: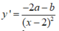
+) Giao điểm của tiếp tuyến của đồ thị (C) tại giao điểm của (C) và trục Ox là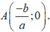
+ ) Tiếp tuyến tại A có phương trình: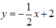
+) Tiếp tuyến tại A có hệ số góc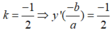
- Giải hệ phương trình (1) và (2) ta được: a = -1, b = 4.