Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Theo bài ra ta có : \(P\left(x\right)+Q\left(x\right)+R\left(x\right)=0\)
\(\Leftrightarrow x^5-x^4+x^4-x^3+R\left(x\right)=0\)
\(\Leftrightarrow x^5-x^3=R\left(x\right)\)
Từ những Đk trên suy ra : \(P\left(x\right)+Q\left(x\right)+R\left(x\right)=x^5-x^4+x^4-x^3+x^5-x^3=0\)
\(\Leftrightarrow2x^5-2x^3=0\)
Vậy P(x) + Q(x) + R(x) là đa thức.

Theo đề bài ta có : \(P\left(x\right)+Q\left(x\right)+R\left(x\right)=0\)
\(\Rightarrow\left(x^5-x^4\right)+\left(x^4-x^3\right)+R\left(x\right)=0\)
\(\Leftrightarrow x^5-x^4+x^4-x^3+R\left(x\right)=0\)
\(\Leftrightarrow x^5-x^3+R\left(x\right)=0\)
\(\Rightarrow R\left(x\right)=x^3-x^5\)
Vậy đa thức \(R\left(x\right)=x^3-x^5\)

a: Q(x)=3x^4+x^3+2x^2+x+1-2x^4+x^2-x+2
=x^4+x^2+3x^2+3
b: H(x)=2x^4-x^2+x-2-x^4+x^3-x^2+2
=x^4+x^3-2x^2+x
c: R(x)=2x^3+x^2+1+2x^4-x^2+x-2
=2x^4+2x^3+x-1

Ta có : \(P\left(x\right)+Q\left(x\right)+R\left(x\right)\)
\(\Leftrightarrow\left(x^5-x^4\right)+\left(x^4-x^3\right)+R\left(x\right)\)
\(\Leftrightarrow x^5-x^4+x^4-x^3+R\left(x\right)\)
\(\Leftrightarrow x^5-x^3+R\left(x\right)\)Đặt \(x^5-x^3+R\left(x\right)=0\)
\(\Leftrightarrow R\left(x\right)=-x^5+x^3\) => Đa thức chứ còn j nữa =))

a) Vì P(x) + Q(x) = x5 – 2x2 + 1 nên
Q(x) = x5 – 2x2 + 1 – P(x)
b) Vì P(x) – R(x) = x3 nên
R(x) = P(x) – x3
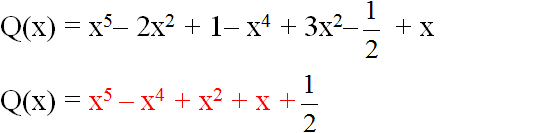
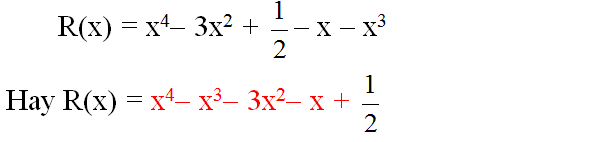
a. \(x^4-5x^3+4x-5-x^4+3x^2+2x+1\)
\(=-5x^3+3x^2+6x-4\)
b. \(R\left(x\right)=x^4-5x^3+4x-5-\left(-x^4+3x^2+2x+1\right)\)
\(=x^4-5x^3+4x-5+x^4-3x^2-2x-1\)
\(=2x^4-5x^3-3x^2+2x-6\)
a) �(�)+�(�)P(x)+Q(x)
=(�4−5�3+4�−5)+(−�4+3�2+2�+1)=(x4−5x3+4x−5)+(−x4+3x2+2x+1)
=�4−5�3+4�−5−�4+3�2+2�+1=x4−5x3+4x−5−x4+3x2+2x+1
=(�4−�4)−5�3+3�2+(4�+2�)+(1−5)=(x4−x4)−5x3+3x2+(4x+2x)+(1−5)
=−5�3+3�2+6�−4=−5x3+3x2+6x−4
b) �(�)=�(�)−�(�)R(x)=P(x)−Q(x)
=(�4−5�3+4�−5)−(−�4+3�2+2�+1)=(x4−5x3+4x−5)−(−x4+3x2+2x+1)
=�4−5�3+4�−5+�4−3�2−2�−1=x4−5x3+4x−5+x4−3x2−2x−1
=(�4+�4)−5�3−3�2+(4�−2�)+(−1−5)=(x4+x4)−5x3−3x2+(4x−2x)+(−1−5)
=2�4−5�3−3�2+2�−6=2x4−5x3−3x2+2x−6