Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Ta có :
\(A=x^4-2x^3+3x^2-5x+10\)
\(=x^4-x^3+x^2-2x^3+2x^2-2x-3x+10\)
\(=x^2\left(x^2-x+1\right)-2x\left(x^2-x+1\right)-3x+10\)
\(=\left(x^2-x+1\right)\left(x^2-2x\right)-3x+10=B.Q+R\)
\(\Rightarrow\hept{\begin{cases}Q=x^2-2x\\R=-3x+10\end{cases}}\)

\(\dfrac{A}{B}=\dfrac{2x^4-10x^3+3x^2-3x+2}{2x^2+1}=\dfrac{2x^4+x^2-10x^3-5x+2x^2+1+2x+1}{2x^2+1}\)
\(=x^2-x+1+\dfrac{2x+1}{2x^2+1}\)
R=2x+1
\(A=\left(x^2-x+1\right)\left(2x^2+1\right)+2x+1\)

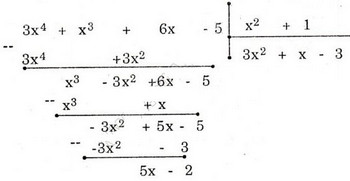
Vậy 3x4 + x3 + 6x – 5 = (x2+ 1)(3x2 + x – 3) + 5x - 2
Xem thêm tại: http://loigiaihay.com/bai-69-trang-31-sgk-toan-8-tap-1-c43a4819.html#ixzz4entQxTTD

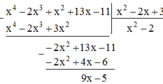
Thương Q = x 2 - 2
Dư R = 9x – 5
Ta thấy x 4 - 2 x 3 + x 2 + 13 x - 11 = ( x 2 - 2 x + 3 )( x 2 - 2 ) + (9x – 5)
Vậy A = B.Q + R

\(a.\frac{x^3-6x^2+12x-8+x^2-4x+4}{x-2}\)\(=\frac{\left(x-2\right)^3+\left(x-2\right)^2}{x-2}\)\(=2\left(x-2\right)^2\)

2x^3+3x^2-x+a x^2+x-1 2x+1 2x^3+x^2 - - 2x^2-x+a 2x^2+x -2x+a -2x-1 - a+1
Để \(A\left(x\right)⋮B\left(x\right)\Leftrightarrow a+1=0\)
\(\Leftrightarrow a=-1\)
Vậy ...

đây ạ