K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
4 tháng 5 2018
BPT\(\Leftrightarrow\left(2017x^2+2018\right)\left(2x-1\right)-\left(2017x^2+2018\right)\left(4-5x\right)\ge0\)
\(\Leftrightarrow\left(2017x^2+2018\right)\left(2x-1-4+5x\right)\ge0\)
\(\Leftrightarrow\left(2017x^2+2018\right)\left(7x-5\right)\ge0\)
DO 2017x2+2018 luôn luôn lớn hơn 0
ĐỂ B PT \(\ge\)0\(\Leftrightarrow7x-5\ge0\)
\(\Leftrightarrow x\ge\frac{5}{7}\)
vậy ...........
12 tháng 1 2018
x^4+2018x^2+2017x+2018
=(x^4-x)+(2018x^2+2018x+2018)
=x(x^3-1)+2018(x^2+x+1)
=x(x-1)(x^2+x+1)+2018(x^2+x+1)
=(x^2+x+1)(x^2-x+2018)
20 tháng 10 2017
\(A=x^3+2x^2+3x\\ =x\left(x^2+2x+1\right)\\ =x\left(x+1\right)^2\\ =1999.\left(1999+1\right)=1999.2000\\ =3998000\)
\(B=x^4-2017x^3+2017x^2-2017x+2018\\ =x^4-2016x^3-x^3+2016x^2+x^2-2016x-x+2016+2\\ =x^3\left(x-2016\right)-x^3\left(x-2016\right)+x\left(x-2016\right)-\left(x-2016\right)+2\\ =\left(x-2016\right)\left(x^3+x-1\right)+2=0+2=0\)
Bạn xem lại đề câu a nhé , theo mk thì phải là 2 thì tính ms nhanh đc, 3 thì cũng giải đc nhưng ko hợp lí lắm
NH
23 tháng 1 2020
\(x^4+2018x^2+2017x+2018\)
\(=x^4+2018x^2+2018x-x+2018\)
\(=x^4-x+2018x^2+2018x+2018\)
\(=x\left(x^3-1\right)+2018\left(x^2+x+1\right)\)
\(=x\left(x-1\right)\left(x^2+x+1\right)+2018\left(x^2+x+1\right)\)
\(=\left(x^2+x+1\right)\left[x\left(x-1\right)+2018\right]\)
\(=\left(x^2+x+1\right)\left(x^2-x+2018\right)\)
24 tháng 1 2020
x^4+2018x^2−2017x+2018
=(x^4+x)+(2018x^2−2018x+2018)
=x(x^3+1)+2018(x^2−x+1)
=x(x+1)(x^2−x+1)+2018(x^2−x+1)
=(x^2−x+1)[x(x+1)+2018]
=(x^2−x+1)(x^2+x+2018)
=(x^2−x+1)(x^2+x+2018)
LN
3 tháng 7 2018
Câu c) Sử dụng hằng đẳng thức+Đặt biến phụ
Ta có: \(x^2+2xy+y^2-x-y-12\)
\(=\left(x+y\right)^2-\left(x+y\right)-12\)
\(=\left(x+y\right)\left(x+y-1\right)-12\)
Đặt: \(x+y=t\)
\(=t\left(t-1\right)-12\)
\(=t^2-t-12\)
\(=t^2-t-9-3\)
\(=\left(t^2-3^2\right)-\left(t+3\right)\)
\(=\left(t+3\right)\left(t-3\right)-\left(t+3\right)\)
\(=\left(t+3\right)\left(t-4\right)\)Bn tự thế vào nhá. (Bài c) tương tự bài a))
Câu d) Đặt biến phụ
Ta có: \(\left(5x^2-2x\right)^2+2x-5x^2-6\)
\(=\left(5x^2-2x\right)^2-5x^2+2x-6\)
\(=\left(5x^2-2x\right)^2-\left(5x^2-2x\right)-6\)
\(=\left(5x^2-2x\right)\left(5x^2-2x-1\right)-6\)
Đặt \(t=5x^2-2x\)
\(=t\left(t-1\right)-6\)
\(=t^2-t-6\)
\(=t^2-t-9+3\)
\(=\left(t^2-3^2\right)-\left(t-3\right)\)
\(=\left(t-3\right)\left(t+3\right)-\left(t-3\right)\)
\(=\left(t-3\right)\left(t+2\right)\)Bn tự thế t vào
LN
3 tháng 7 2018
Câu a) Sử dụng phương pháp đặt biến phụ+hằng đẳng thức
Ta có: \(\left(2x^2+x-2\right)\left(2x^2+x-3\right)-12\)
Đặt: \(t=2x^2+x-2\)
\(=t\left(t-1\right)-12\)
\(=t^2-t-12=t^2-t-9-3\)
\(=\left(t^2-3^2\right)-\left(t+3\right)\)
\(\left(t+3\right)\left(t-3\right)-\left(t+3\right)=\left(t+3\right)\left(t-4\right)\)
Thay t vào: \(\left(2x^2+x+1\right)\left(2x^2+x-6\right)\)
Câu b) Sử dụng hằng đẳng thức+ đặt biến phụ
Ta có: \(x^2+9y^2-9y-3x+6xy+2\)
\(=\left(x^2+6xy+9y^2\right)-\left(9y+3x\right)+2\)
\(=\left(x+3y\right)^2-3\left(3y+x\right)+2\)
\(=\left(x+3y\right)\left(x+3y-3\right)+2\)
Đặt \(t=x+3y\)
\(=t\left(t-3\right)+2\)
\(=t^2-3t+2\)
\(=\left(t^2-4\right)-\left(3t-6\right)\)
\(=\left(t-2\right)\left(t+2\right)-3\left(t-2\right)\)
\(=\left(t-2\right)\left(t-1\right)\)Khúc sau bn tự thế vào
Còn mấy bài sau đang nghiên cứu
1
14 tháng 5 2018
A=1/2017-2/2017x+2018/2017x^2
1/2017x=y
A=2018y^2-2y+1/2017
A=2018(y^2-2.y./2018+1/2018^2)
-1/2018+1/(2017)
A=2018(y-1/2018)^2-1/(2018.2017)
GTNNA=1/(2017.2018)
khi y=1/2018
x=2017/2018
23 tháng 3 2018
Ta có : x4 + 2018x2 + 2017x + 2018
= x4 - x + 2018x2 + 2018x + 2018
= x(x3 - 1) + 2018(x2 + x + 1)
= x(x - 1)(x2 + x + 1) + 2018(x2 + x + 1)
= (x2 + x + 1)(x2 - x + 2018)
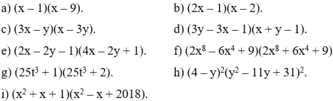
Vì 2017x2 ≥ 0
⇒ 2017x2 + 2018 > 0
Nên (2017x2 + 2018)(2x - 1) ≥ (2017x2 + 2018)(4 - 5x)
⇔ 2x - 1 ≥ 4 - 5x ( chia cho số dương nên bất phương trình không đổi chiều)
⇔ 2x + 5x ≥ 4 + 1
⇔7x ≥ 5
⇔ x ≥ \(\frac{5}{7}\)
Vậy nghiệm của phương trình là x ≥ \(\frac{5}{7}\)