
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a.
\(\Leftrightarrow2cos^2x-1-\sqrt{3}sin2x-\sqrt{3}sinx-cosx+4=0\)
\(\Leftrightarrow2cos^2x-2\sqrt{3}sinx.cosx-\sqrt{3}sinx-cosx+3=0\)
\(\Leftrightarrow4cos^2x-4\sqrt{3}sinx.cosx-2\sqrt{3}sinx-2cosx+6=0\)
\(\Leftrightarrow4cos^2x-4\sqrt{3}sinx.cosx-2\sqrt{3}sinx-2cosx+2+4\left(sin^2x+cos^2x\right)=0\)
\(\Leftrightarrow\dfrac{1}{2}\left(4cos^2x-4cosx+1\right)+\dfrac{1}{2}\left(4sin^2x-4\sqrt{3}sinx+3\right)+2\left(3cos^2x-2\sqrt{3}sinx.cosx+sin^2x\right)=0\)
\(\Leftrightarrow\dfrac{1}{2}\left(2cosx-1\right)^2+\dfrac{1}{2}\left(2sinx-\sqrt{3}\right)^2+2\left(\sqrt{3}cosx-sinx\right)^2=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}2cosx-1=0\\2sinx-\sqrt{3}=0\\\sqrt{3}cosx-sinx=0\end{matrix}\right.\)
\(\Leftrightarrow x=\dfrac{\pi}{3}+k2\pi\)
b.
\(\Leftrightarrow\left(3tan^2x-2\sqrt{3}tanx+1\right)+\left(4sin^2x-4sinx+1\right)=0\)
\(\Leftrightarrow\left(\sqrt{3}tanx-1\right)^2+\left(2sinx-1\right)^2=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}\sqrt{3}tanx-1=0\\2sinx-1=0\end{matrix}\right.\)
\(\Leftrightarrow x=\dfrac{\pi}{6}+k2\pi\)


Giả thiết suy ra MN là đường trung bình tam giác ABC \(\Rightarrow MN||BC\)
Mà \(\left\{{}\begin{matrix}MN=\left(DMN\right)\cap\left(ABC\right)\\BC=\left(BCD\right)\cap\left(ABC\right)\end{matrix}\right.\)
Và D là 1 điểm chung của (BCD) và (DMN)
\(\Rightarrow\) Giao tuyến của (BCD) và (DMN) phải là 1 đường thẳng qua D và song song MN (hoặc BC)

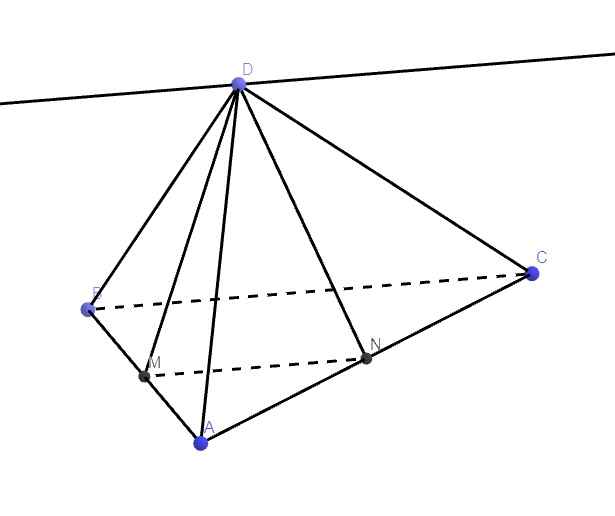
Do vai trò của 3 biến là như nhau, không mất tính tổng quát giả sử \(x>y>z\)
Ta có: \(x-z=\left(x-y\right)+\left(y-z\right)\)
Đặt \(\left\{{}\begin{matrix}x-y=a>0\\y-z=b>0\end{matrix}\right.\)
Do \(x;z\in\left[0;2\right]\Rightarrow x-z\le2\) hay \(a+b\le2\)
Ta có:
\(P=\dfrac{1}{a^2}+\dfrac{1}{b^2}+\dfrac{1}{\left(a+b\right)^2}\ge\dfrac{1}{2}\left(\dfrac{1}{a}+\dfrac{1}{b}\right)^2+\dfrac{1}{\left(a+b\right)^2}\ge\dfrac{1}{2}\left(\dfrac{4}{a+b}\right)^2+\dfrac{1}{\left(a+b\right)^2}\)
\(P\ge\dfrac{9}{\left(a+b\right)^2}\ge\dfrac{9}{2^2}=\dfrac{9}{4}\)
Dấu "=" xảy ra khi \(\left\{{}\begin{matrix}a=b\\a+b=2\\\end{matrix}\right.\) \(\Rightarrow a=b=1\) hay \(\left(x;y;z\right)=\left(0;1;2\right)\) và các hoán vị

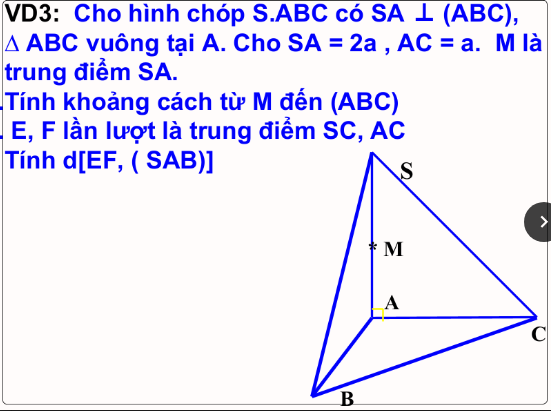
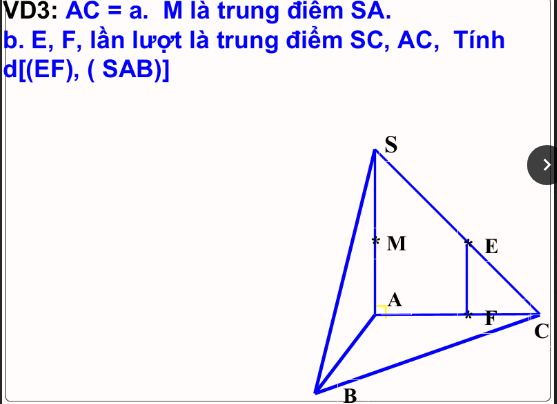
Khoảng cách từ M để ABC bằng MA
Khoảng cách từ EF đến SAB bằng EM = AF





18.
\(=lim\frac{\sqrt{1+\frac{2}{n}}}{1-\sqrt{3+\frac{1}{n^2}}}=\frac{1}{1-\sqrt{3}}\)
19.
\(=lim\frac{n\left(\sqrt{1+\frac{1}{n^2}}-\sqrt[3]{3+\frac{2}{n^3}}\right)}{n\sqrt[4]{2+\frac{2}{n^4}}}=lim\frac{\sqrt{1+\frac{1}{n^2}}-\sqrt[3]{3+\frac{2}{n^3}}}{\sqrt[4]{2+\frac{2}{n^4}}}=\frac{1-\sqrt[3]{3}}{\sqrt[4]{2}}\)
24.
\(=lim\frac{n\left(\sqrt[4]{1-\frac{2}{n^3}+\frac{1}{n^4}}+2\right)}{n\left(\sqrt[3]{3+\frac{1}{n^2}}-1\right)}=lim\frac{\sqrt[4]{1-\frac{2}{n^3}+\frac{1}{n^4}}+2}{\sqrt[3]{3+\frac{1}{n^2}}-1}=\frac{1+2}{\sqrt[3]{3}-1}=\frac{3}{\sqrt[3]{3}-1}\)