
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 2:
c: Ta có: \(3x-\sqrt{x-1}=5\)
\(\Leftrightarrow\sqrt{x-1}=3x-5\)
\(\Leftrightarrow9x^2-30x+25-x+1=0\)
\(\Leftrightarrow9x^2-31x+26=0\)
\(\text{Δ}=\left(-31\right)^2-4\cdot9\cdot26=25\)
Vì Δ>0 nên phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x_1=\dfrac{31-5}{18}=\dfrac{13}{9}\\x_2=\dfrac{31+5}{18}=2\end{matrix}\right.\)

Bài 3:
a: Ta có: \(x-2\sqrt{x+8}=0\)
\(\Leftrightarrow\sqrt{4x+32}=x\)
\(\Leftrightarrow4x+32=x^2\)
\(\Leftrightarrow x^2-4x-32=0\)
\(\Leftrightarrow\left(x-8\right)\left(x+4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=8\left(nhận\right)\\x=-4\left(loại\right)\end{matrix}\right.\)
b: Ta có: \(3\sqrt{x+5}=4x-7\)
\(\Leftrightarrow\left(4x-7\right)^2=9x+45\)
\(\Leftrightarrow16x^2-56x+49-9x-45=0\)
\(\Leftrightarrow16x^2-65x+4=0\)
\(\Leftrightarrow16x^2-64x-x+4=0\)
\(\Leftrightarrow\left(x-4\right)\left(16x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=4\\x=\dfrac{1}{16}\end{matrix}\right.\)

Bài 1:
Gọi chiều dài là x(Điều kiện: x>10,5)
Chiều rộng là 21-x
Theo đề, ta có: \(x^2+\left(21-x\right)^2=225\)
\(\Leftrightarrow x^2+x^2-42x+441-225=0\)
\(\Leftrightarrow2x^2-42x+216=0\)
\(\Leftrightarrow x^2-21x+108=0\)
\(\Leftrightarrow\left(x-12\right)\left(x-9\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=12\left(nhận\right)\\x=9\left(loại\right)\end{matrix}\right.\)
vậy: Chiều dài là 12m
Chiều rộng là 9m

Bài 1:
c: Vì a+b+c=0 nên phương trình có hai nghiệm phân biệt là
x1=1; \(x2=\dfrac{c}{a}=\dfrac{3\sqrt{2}+1}{1-\sqrt{2}}\)

a: \(\left\{{}\begin{matrix}3x+6y=4\\x+4y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=\dfrac{1}{3}\\x=\dfrac{2}{3}\end{matrix}\right.\)

a: \(\left\{{}\begin{matrix}3x+6y=4\\x+4y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3x+6y=4\\3x+12y=6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-6y=-2\\x+4y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=\dfrac{1}{3}\\x=\dfrac{2}{3}\end{matrix}\right.\)

bài 2
a) ĐKXĐ: a\(\ge\)0, a\(\ne\)1
b)P=\(\dfrac{1+\sqrt{a}-1+\sqrt{a}}{\left(1-\sqrt{a}\right)\left(1+\sqrt{a}\right)}\).\(\dfrac{1+\sqrt{a}}{\sqrt{a}}\)
P=\(\dfrac{2\sqrt{a}}{\left(1-\sqrt{a}\right)\left(1+\sqrt{a}\right)}.\dfrac{1+\sqrt{a}}{\sqrt{a}}\)
P=\(\dfrac{2}{1-\sqrt{a}}\)
c) thay a=4 vào biểu thức ta có
P=\(\dfrac{2}{1-\sqrt{4}}\)=\(\dfrac{2}{1-2}\)=-2
d) để P=9 thì
\(\dfrac{2}{1-\sqrt{a}}=9\)\(\Rightarrow\)2=9(1-\(\sqrt{a}\))
\(\Rightarrow\)2=9-\(9\sqrt{a}\)\(\Rightarrow\)\(9\sqrt{a}=7\)\(\Rightarrow\)\(\sqrt{a}=\dfrac{7}{9}\)
\(\Rightarrow a=\dfrac{49}{81}\)
bài 3
a) \(\sqrt{9x^2}=4\Rightarrow3x=4\)\(\Rightarrow\)\(x=\dfrac{4}{3}\)
b)\(\Rightarrow\)\(\left(x-\sqrt{5}\right)^2\)=0\(\Rightarrow x-\sqrt{5}=0\)
\(\Rightarrow x=\sqrt{5}\)

Bài 2:
\(b,\Delta=4\left(m+1\right)^2-4m\left(m+2\right)\\ =4m^2+8m+4-4m^2-8m=4>0,\forall m\)
Vậy PT có 2 nghiệm phân biệt với mọi m
Khi đó \(\left[{}\begin{matrix}x=\dfrac{2\left(m+1\right)-2}{2}=m\\x=\dfrac{2\left(m+1\right)+1}{2}=m+2\end{matrix}\right.\)
Bài 3:
Gọi nghiệm chung 2 PT là \(x_1\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1^2-\left(m+4\right)x_1+m+5=0\left(1\right)\\x_1^2-\left(m+2\right)x_1+m+1=0\left(2\right)\end{matrix}\right.\\ \Leftrightarrow\left(1\right)-\left(2\right)=-\left(m+4\right)x_1+m+5+\left(m+2\right)x_1-m-1=0\\ \Leftrightarrow x_1\left(-m-4+m+2\right)+4=0\\ \Leftrightarrow-2x_1=-4\Leftrightarrow x_1=-2\)
Thay \(x_1=-2\) vào \(\left(2\right)\Leftrightarrow4+2\left(m+2\right)+m+1=0\)
\(\Leftrightarrow3m+9=0\Leftrightarrow m=-3\)
Bài 2:
\(a,\forall m=0\\ PT\Leftrightarrow-2\left(m-1\right)x=-2\left(m-1\right)\Leftrightarrow x=1\\ \forall m\ne0\\ \Delta=4\left(m-1\right)^2-8m\left(m-1\right)\\ =4m^2-8m+4-8m^2+8m\\ =4-4m^2\)
PT vô nghiệm \(\Leftrightarrow4-4m^2< 0\Leftrightarrow m^2>1\Leftrightarrow\left[{}\begin{matrix}m>1\\m< -1\end{matrix}\right.\)
PT có nghiệm kép \(\Leftrightarrow4-4m^2=0\Leftrightarrow\left(1-m\right)\left(1+m\right)=0\Leftrightarrow\left[{}\begin{matrix}m=1\\m=-1\end{matrix}\right.\)
Khi đó \(x=\dfrac{2\left(m-1\right)}{2m}=\dfrac{m-1}{m}\)
PT có 2 nghiệm phân biệt \(\Leftrightarrow4-4m^2>0\Leftrightarrow-1< m< 1;m\ne0\)
Khi đó \(x=\dfrac{2\left(m-1\right)\pm\sqrt{4-4m^2}}{2m}=\dfrac{2\left(m-1\right)\pm2\sqrt{1-m^2}}{2m}=\dfrac{m-1\pm\sqrt{1-m^2}}{2}\)


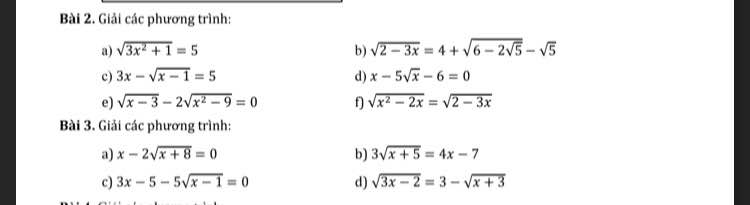
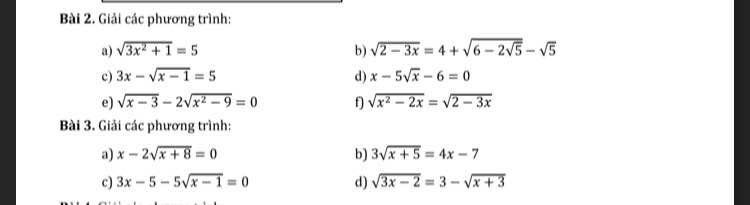






 PP
PP