
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Câu 1: Điều kiện \(D=\left(-\infty;0\right)U\left(1;+\infty\right)\)
\(y'=\frac{\sqrt{x^2-x}-x.\frac{2x-1}{2\sqrt{x^2-x}}}{x^2-x}=\frac{-x}{2\left(x^2-x\right)\sqrt{x^2-x}}\)
Ta thấy \(y'< 0\) trên \(\left(1;+\infty\right)\), suy ra hàm số nghịch biến trên \(\left(1;+\infty\right)\).
Câu 2:
\(y'=1+\frac{2x}{\sqrt{2x^2+1}}=\frac{2x+\sqrt{2x^2+1}}{\sqrt{2x^2+1}}\)
Xét bất phương trình:
\(2x+\sqrt{2x^2+1}< 0\)
\(\Leftrightarrow\sqrt{2x^2+1}< -2x\)
\(\Leftrightarrow\hept{\begin{cases}x< 0\\2x^2+1< 4x^2\end{cases}}\Leftrightarrow\hept{\begin{cases}x< 0\\x< \frac{-\sqrt{2}}{2}\left(h\right)x>\frac{\sqrt{2}}{2}\end{cases}}\Leftrightarrow x< \frac{-\sqrt{2}}{2}\)
Vậy hàm số nghịch biến trên \(\left(-\infty;\frac{-\sqrt{2}}{2}\right)\).

a) Tập xác định: D = R\{m}
Hàm số đồng biến trên từng khoảng (−∞;m),(m;+∞)(−∞;m),(m;+∞)khi và chỉ khi:
y′=−m2+4(x−m)2>0⇔−m2+4>0⇔m2<4⇔−2<m<2y′=−m2+4(x−m)2>0⇔−m2+4>0⇔m2<4⇔−2<m<2
b) Tập xác định: D = R\{m}
Hàm số nghịch biến trên từng khoảng khi và chỉ khi:
y′=−m2+5m−4(x+m)2<0⇔−m2+5m−4<0y′=−m2+5m−4(x+m)2<0⇔−m2+5m−4<0
[m<1m>4[m<1m>4
c) Tập xác định: D = R
Hàm số nghịch biến trên R khi và chỉ khi:
y′=−3x2+2mx−3≤0⇔′=m2−9≤0⇔m2≤9⇔−3≤m≤3y′=−3x2+2mx−3≤0⇔′=m2−9≤0⇔m2≤9⇔−3≤m≤3
d) Tập xác định: D = R
Hàm số đồng biến trên R khi và chỉ khi:
y′=3x2−4mx+12≥0⇔′=4m2−36≤0⇔m2≤9⇔−3≤m≤3

*Xét hàm số: y= -x3 + 2x2 – x – 7
Tập xác định: D = R
\(y'\left(x\right)=-3x^2+4x-1\); \(y'\left(x\right)=0\Leftrightarrow\left[{}\begin{matrix}x=1\\x=\dfrac{1}{3}\end{matrix}\right.\)
y’ > 0 với và y’ < 0 với \(x \in ( - \infty ,{1 \over 3}) \cup (1, + \infty )
Vậy hàm số đồng biến trong (\(\dfrac{1}{3}\),1)(\(\dfrac{1}{3}\),1) và nghịch biến trong (−∞,13)∪(1,+∞)(−∞,13)b) Xét hàm số: \(y=\dfrac{x-5}{1-x}\).
Tập xác định: D = R{1}
\(y'=\dfrac{-4}{\left(1-x\right)^2}< 0,\forall x\in D\)
Vậy hàm số nghịch biến trong từng khoảng (-∞,1) và (1, +∞)

1.
Xét \(x^2-mx+m=0\) (1)
\(\Delta=m^2-4m\)
Hàm có đúng 1 tiệm cận đứng khi:
TH1: \(\Delta=0\Leftrightarrow\left[{}\begin{matrix}m=0\\m=4\end{matrix}\right.\)
Th2: (1) có 1 nghiệm \(x=1\)
\(\Leftrightarrow1-m+m=0\left(ktm\right)\)
Vậy \(m\in\left\{0;4\right\}\)
2.
\(\Leftrightarrow m=\frac{x^3+x^2+x}{\left(x^2+1\right)^2}\)
Xét hàm \(f\left(x\right)=\frac{x^3+x^2+x}{\left(x^2+1\right)^2}\Rightarrow f'\left(x\right)=\frac{\left(1-x\right)\left(x+1\right)^2}{\left(x^2+1\right)^3}\ge0;\forall x\in\left[0;1\right]\)
Hàm đồng biến trên [0;1] \(\Rightarrow f\left(0\right)\le m\le f\left(1\right)\Leftrightarrow0\le m\le\frac{3}{4}\)
3.
\(y'=-2sin2x-4sinx=0\Leftrightarrow sinx=0\)
\(\Rightarrow x=k\pi\)
\(y\left(0\right)=6\) ; \(y\left(\pi\right)=-2\)
\(\Rightarrow M=6\)
4.
\(y'=\frac{-1}{\left(x-1\right)^2}< 0\Rightarrow\) hàm số nghịch biến trên các khoảng \(\left(-\infty;1\right)\) và \(\left(1;+\infty\right)\)
5.
\(y'=\frac{-m\left(m-1\right)+2}{\left(sinx-m\right)^2}.cosx< 0\Leftrightarrow-m^2+m+2< 0\)
\(\Leftrightarrow m\in\left(-\infty;-1\right)\cup\left(2;+\infty\right)\)


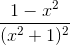 => y' = 0 ⇔ x=-1 hoặc x=1.
=> y' = 0 ⇔ x=-1 hoặc x=1.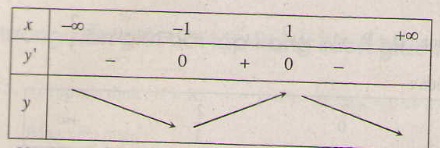




Chọn D.
y' không xác định khi x = -1 . Bảng biến thiên:
Hàm số nghịch biến trên các khoảng (-4;-1) và (-1;2)