Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Chú ý. Đối với những hệ phương trình có hệ số thập phân như thế này ta nên nhân với 10 để có hệ phương trình hệ số nguyên:
![]()
Thay vào ta thấy phương án A sai, còn phương án B đúng. Vậy đáp án là B.
Đáp án: B

Cách 1: Ta có :
x + y = 2 x - y = 3 a - 2 ⇔ x + y = 2 2 x = 3 a ⇔ y = 2 - 3 a 2 x = 3 a 2 .
Để x < y ⇔ 3 a 2 < 2 - 3 a 2 ⇔ 3 a < 2 ⇔ a < 2 3
Đáp án là D.
Cách 2: Có thể không cần tìm nghiệm của hệ bất phương trình , chỉ cần lập luận nếu x, y là nghiệm của hệ thì: x < y ⇔ x - y < 0 ⇔ 3 a - 2 < 0 ⇔ a < 2 3 .
Đáp án là D.

Đặt \(x+\dfrac{1}{x}=a;y+\dfrac{1}{y}=b\left(\left|a\right|\ge2;\left|b\right|\ge2\right)\)
\(\left\{{}\begin{matrix}x+\dfrac{1}{x}+y+\dfrac{1}{y}=5\\x^3+y^3+\dfrac{1}{x^3}+\dfrac{1}{y^3}=15m-25\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+\dfrac{1}{x}+y+\dfrac{1}{y}=5\\\left(x^3+\dfrac{1}{x^3}\right)+\left(y^3+\dfrac{1}{y^3}\right)=15m-25\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+\dfrac{1}{x}+y+\dfrac{1}{y}=5\\\left(x+\dfrac{1}{x}\right)^3-3\left(x+\dfrac{1}{x}\right)+\left(y+\dfrac{1}{y}\right)^3-3\left(y+\dfrac{1}{y}\right)=15m-25\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+\dfrac{1}{x}+y+\dfrac{1}{y}=5\\\left(x+\dfrac{1}{x}\right)^3+\left(y+\dfrac{1}{y}\right)^3-3\left(x+\dfrac{1}{x}+y+\dfrac{1}{y}\right)=15m-25\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+\dfrac{1}{x}+y+\dfrac{1}{y}=5\\\left(x+\dfrac{1}{x}\right)^3+\left(y+\dfrac{1}{y}\right)^3=15m-10\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}a+b=5\\a^3+b^3=15m-10\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}a+b=5\\\left(a+b\right)^3-3ab\left(a+b\right)=15m-10\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}a+b=5\\125-15ab=15m-10\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}a+b=5\\ab=9-m\end{matrix}\right.\)
\(\Rightarrow a,b\) là nghiệm của phương trình \(t^2-5t+9-m=0\left(1\right)\)
a, Nếu \(m=3\), phương trình \(\left(1\right)\) trở thành
\(t^2-5t+6=0\)
\(\Leftrightarrow\left[{}\begin{matrix}t=2\\t=3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}a=2\\b=3\end{matrix}\right.\\\left\{{}\begin{matrix}a=3\\b=2\end{matrix}\right.\end{matrix}\right.\)
TH1: \(\left\{{}\begin{matrix}x+\dfrac{1}{x}=2\\y+\dfrac{1}{y}=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left(x-1\right)^2=0\\y^2-3y+1=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=\dfrac{3\pm\sqrt{5}}{2}\end{matrix}\right.\)
TH2: \(\left\{{}\begin{matrix}x+\dfrac{1}{x}=3\\y+\dfrac{1}{y}=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{3\pm\sqrt{5}}{2}\\y=1\end{matrix}\right.\)
Vậy ...
b, \(\left(1\right)\Leftrightarrow t=\dfrac{5\pm\sqrt{4m-11}}{2}\left(m\ge\dfrac{11}{4}\right)\)
\(\left(1\right)\Leftrightarrow\left\{{}\begin{matrix}a=\dfrac{5\pm\sqrt{4m-11}}{2}\\b=\dfrac{5\mp\sqrt{4m-11}}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+\dfrac{1}{x}=\dfrac{5\pm\sqrt{4m-11}}{2}\\y+\dfrac{1}{y}=\dfrac{5\mp\sqrt{4m-11}}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x^2-\left(5\pm\sqrt{4m-11}\right)+2=0\left(2\right)\\2y^2-\left(5\mp\sqrt{4m-11}\right)+2=0\end{matrix}\right.\)
Yêu cầu bài toán thỏa mãn khi phương trình \(\left(2\right)\) có nghiệm dương
\(\Leftrightarrow\left\{{}\begin{matrix}\Delta=\left(5\pm\sqrt{4m-11}\right)^2-16\ge0\\\dfrac{5\pm\sqrt{4m-11}}{2}>0\\1>0\end{matrix}\right.\)
\(\Leftrightarrow...\)

Viết lại hệ \(\left\{{}\begin{matrix}2x+y=5\\-x+2y=a+5\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x+y=5\\-2x+4y=2a+10\end{matrix}\right.\)
\(\Rightarrow5y=2a+15\Leftrightarrow y=\dfrac{2a+15}{5}\)
\(\Leftrightarrow x=2y-a-5=\dfrac{5-a}{5}\)
\(xy=\dfrac{5-a}{5}.\dfrac{2a+15}{5}=\dfrac{-2a^2-5a+75}{25}=\dfrac{-\left(a+\dfrac{5}{4}\right)^2+\dfrac{625}{8}}{25}\le\dfrac{25}{8}\)
\(max=\dfrac{25}{8}\Leftrightarrow a=-\dfrac{5}{4}\)

\(\left\{{}\begin{matrix}x+y+xy=m\\x^2+y^2=m\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x+y+xy=m\\\left(x+y\right)^2-2xy=m\end{matrix}\right.\)
Đặt x+y=a, xy=b, hệ phương trình trở thành:
\(\left\{{}\begin{matrix}a+b=m\\a^2-2b=m\end{matrix}\right.\)
a)Với m=5, hệ phương trình trở thành:
\(\left\{{}\begin{matrix}a+b=5\\a^2-2b=5\end{matrix}\right.\)\(\Leftrightarrow\) \(\left\{{}\begin{matrix}b=5-a\\a^2-2\left(5-a\right)-5=0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}b=5-a\\\left[{}\begin{matrix}a=-5\\a=3\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}a=3\\b=2\end{matrix}\right.\left(nhận\right)\\\left\{{}\begin{matrix}a=-5\\b=10\end{matrix}\right.\left(loại\right)\end{matrix}\right.\)
\(\Leftrightarrow\)\(\left\{{}\begin{matrix}a=3\\b=2\end{matrix}\right.\)\(\Rightarrow\)\(\left\{{}\begin{matrix}x+y=3\\xy=2\end{matrix}\right.\Leftrightarrow\)\(\left[{}\begin{matrix}\left\{{}\begin{matrix}x=2\\y=1\end{matrix}\right.\\\left\{{}\begin{matrix}x=1\\y=2\end{matrix}\right.\end{matrix}\right.\)
Vậy S={(2;1);(1;2)}
b) Ta có: \(\left\{{}\begin{matrix}a+b=m\\a^2-2b=m\end{matrix}\right.\)\(\Leftrightarrow\)\(\left[{}\begin{matrix}\left\{{}\begin{matrix}a=-1-\sqrt{3m+1}\\b=m+1+\sqrt{3m+1}\end{matrix}\right.\\\left\{{}\begin{matrix}a=-1+\sqrt{3m+1}\\b=m+1-\sqrt{3m+1}\end{matrix}\right.\end{matrix}\right.\)(m\(\ge\)\(\dfrac{-1}{3}\)) (1)
Hệ có nghiệm khi và chỉ khi a2\(\ge\) 4b
\(\Leftrightarrow\)\(\left[{}\begin{matrix}1+2\sqrt{3m-1}+3m-1\ge4m+4+4\sqrt{3m-1}\\1-2\sqrt{3m-1}+3m-1\ge4m+4-4\sqrt{3m-1}\end{matrix}\right.\)
\(\Leftrightarrow\)m\(\ge\)0 (thỏa (1))
Vậy m\(\ge\)0 thì hệ phương trình có nghiệm
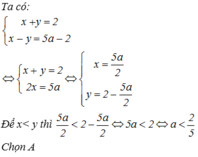
Ta có: x + y = 2 x - y = 5 a - 2 ⇔ x + y = 2 2 x = 5 a ⇔ x = 5 a 2 y = 2 - 5 a 2
Để x< y thì 5 a 2 < 2 - 5 a 2 ⇔ 5 a < 2 ⇔ a < 2 5 .