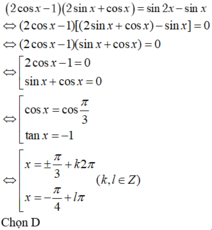K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NS
1
AH
Akai Haruma
Giáo viên
24 tháng 7 2021
Lời giải:
PT $\Leftrightarrow (2\cos x-1)(2\sin x+\cos x)=2\sin x\cos x-\sin x$
$\Leftrightarrow (2\cos x-1)(2\sin x+\cos x)=\sin x(2\cos x-1)$
$\Leftrightarrow (2\cos x-1)(\sin x+\cos x)=0$
$\Rightarrow 2\cos x=1$ hoặc $\sin x=-\cos x=\cos (\pi -x)=\sin (x-\frac{\pi}{2})$
Đến đây thì đơn giản rồi.
AH
Akai Haruma
Giáo viên
24 tháng 7 2021
Nkjuiopmli Sv5: Bạn chuyển vế sin x(2cos x-1) sang vế trái thì vế phải còn 0 đó.
NV
Nguyễn Việt Lâm
Giáo viên
27 tháng 8 2020
c/ ĐKXĐ: \(x\ne\frac{\pi}{2}+k\pi\)
\(\Leftrightarrow\frac{1}{cos^2x}=\frac{1-cos^2x+1-sin^3x}{1-sin^3x}\)
\(\Leftrightarrow\frac{1}{cos^2x}=\frac{sin^2x}{1-sin^3x}+1\)
\(\Leftrightarrow\frac{1}{cos^2x}-1=\frac{sin^2x}{1-sin^3x}\)
\(\Leftrightarrow\frac{1-cos^2x}{cos^2x}=\frac{sin^2x}{1-sin^3x}\)
\(\Leftrightarrow\frac{sin^2x}{cos^2x}=\frac{sin^2x}{1-sin^3x}\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=0\Rightarrow x=k\pi\\cos^2x=1-sin^3x\left(1\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow1-sin^2x=1-sin^3x\)
\(\Leftrightarrow sin^3x-sin^2x=0\Leftrightarrow\left[{}\begin{matrix}sinx=0\\sinx=1\left(l\right)\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
27 tháng 8 2020
b/ ĐKXĐ: \(x\ne\frac{k\pi}{2}\)
\(\Leftrightarrow\frac{sin2x.sinx+cos2x.cosx}{sinx.cosx}=\frac{sinx}{cosx}-\frac{cosx}{sinx}\)
\(\Leftrightarrow\frac{cos\left(2x-x\right)}{sinx.cosx}=\frac{sin^2x-cos^2x}{sinx.cosx}\)
\(\Leftrightarrow cosx=sin^2x-cos^2x\)
\(\Leftrightarrow cosx=1-2cos^2x\)
\(\Leftrightarrow2cos^2x+cosx-1=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=-1\left(l\right)\\cosx=\frac{1}{2}\end{matrix}\right.\)
\(\Rightarrow x=\pm\frac{\pi}{3}+k2\pi\)
