
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Câu 1:
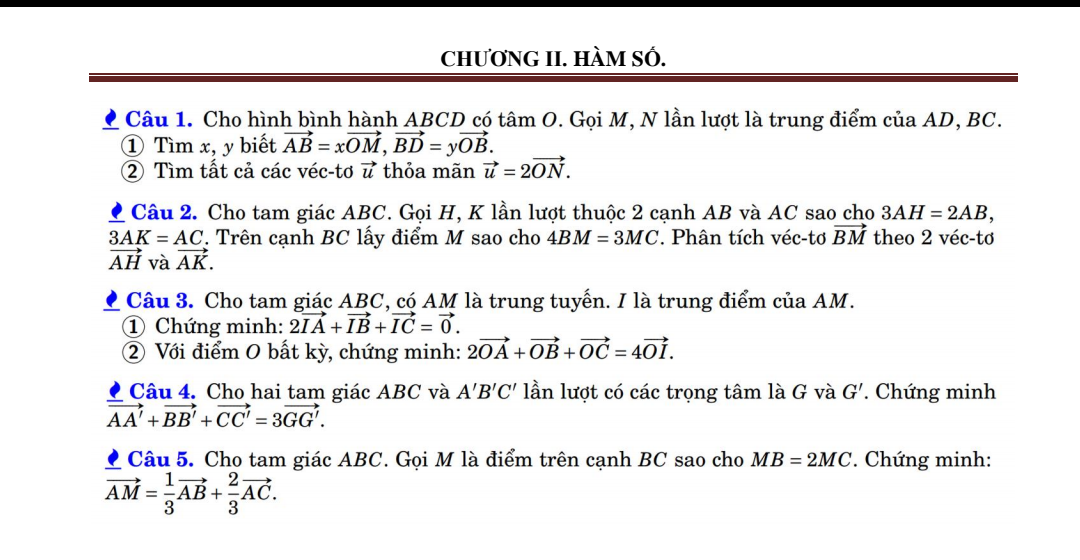
1: \(\overrightarrow{OM}=\dfrac{\overrightarrow{OA}+\overrightarrow{OD}}{2}=\dfrac{\overrightarrow{BO}+\overrightarrow{OA}}{2}=\dfrac{\overrightarrow{BA}}{2}=-\dfrac{1}{2}\overrightarrow{AB}\)
\(\Leftrightarrow x=-\dfrac{1}{2}\)
\(\overrightarrow{BD}=2\cdot\overrightarrow{BO}=-2\cdot\overrightarrow{OB}\)
nên y=-2
2: \(2\cdot\overrightarrow{ON}=\overrightarrow{OB}+\overrightarrow{OC}=\overrightarrow{DO}+\overrightarrow{OC}=\overrightarrow{DC}=\overrightarrow{AB}\)
Vậy: Các vecto u thỏa mãn là vecto DC và vecto AB

Câu 1:
1: \(\overrightarrow{OM}=\dfrac{\overrightarrow{OA}+\overrightarrow{OD}}{2}=\dfrac{\overrightarrow{BO}+\overrightarrow{OA}}{2}=\dfrac{\overrightarrow{BA}}{2}=-\dfrac{1}{2}\overrightarrow{AB}\)
\(\Leftrightarrow x=-\dfrac{1}{2}\)
\(\overrightarrow{BD}=2\cdot\overrightarrow{BO}=-2\cdot\overrightarrow{OB}\)
nên y=-2
2: \(2\cdot\overrightarrow{ON}=\overrightarrow{OB}+\overrightarrow{OC}=\overrightarrow{DO}+\overrightarrow{OC}=\overrightarrow{DC}=\overrightarrow{AB}\)
Vậy: Các vecto u thỏa mãn là vecto DC và vecto AB

Ta có: a=-1<0
=>Bề lõm của (P) quay xuống
M(2;10) là điểm có tung độ lớn nhất mà bề lõm của (P) quay xuống
nên M(2;10) là đỉnh của (P)
Do đó, ta có:
\(\left\{{}\begin{matrix}-\dfrac{b}{2\cdot\left(-1\right)}=2\\-\dfrac{b^2-4\cdot\left(-1\right)\cdot c}{4\cdot\left(-1\right)}=10\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\dfrac{b}{2}=2\\\dfrac{b^2+4c}{4}=10\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}b=4\\b^2+4c=4\cdot10=40\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}b=4\\4c=40-16=24\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}b=4\\c=6\end{matrix}\right.\)
=>\(b\cdot c=24\)


Đặt \(\overrightarrow{b}=x\cdot\overrightarrow{a}+y\cdot\overrightarrow{c}\)
mà \(\overrightarrow{b}=\left(-1;-1\right);\overrightarrow{a}=\left(4;-2\right);\overrightarrow{c}=\left(2;5\right)\)
nên \(\left\{{}\begin{matrix}4x+2y=-1\\-2x+5y=-1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}4x+2y=-1\\-4x+10y=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}12y=-3\\4x+2y=-1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=-\dfrac{1}{4}\\4x=-1-2y=-1-2\cdot\dfrac{-1}{4}=-1+\dfrac{1}{2}=-\dfrac{1}{2}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=-\dfrac{1}{8}\\y=-\dfrac{1}{4}\end{matrix}\right.\)
Vậy: \(\overrightarrow{b}=\dfrac{-1}{8}\cdot\overrightarrow{a}+\dfrac{-1}{4}\cdot\overrightarrow{c}\)


A(m-1;-1); B(2;2-2m); C(m+3;3)
\(\overrightarrow{AB}=\left(2-m+1;2-2m+1\right)\)
=>\(\overrightarrow{AB}=\left(3-m;3-2m\right)\)
\(\overrightarrow{AC}=\left(m+3-m+1;3+1\right)\)
=>\(\overrightarrow{AC}=\left(4;4\right)\)
Để A,B,C thẳng hàng thì \(\dfrac{3-m}{4}=\dfrac{3-2m}{4}\)
=>3-m=3-2m
=>m=0
\(\left\{{}\begin{matrix}\overrightarrow{AB}=\left(3-m;3-2m\right)\\\overrightarrow{AC}=\left(4;4\right)\end{matrix}\right.\)
3 điểm A;B;C thẳng hàng khi và chỉ khi \(\overrightarrow{AB}=k\overrightarrow{AC}\) với \(k\ne0\)
Hay \(\dfrac{3-m}{4}=\dfrac{3-2m}{4}\Rightarrow m=0\)




a) \(\overrightarrow{BA}.\overrightarrow{BC}=\overrightarrow{BA}.\left(\overrightarrow{BA}+\overrightarrow{AC}\right)=\overrightarrow{BA}.\overrightarrow{BA}+\overrightarrow{BA}.\overrightarrow{AC}=BA^2\).
b) \(\overrightarrow{AB}.\overrightarrow{CB}=\overrightarrow{AB}.\left(\overrightarrow{CA}+\overrightarrow{AB}\right)=\overrightarrow{AB}.\overrightarrow{CA}+\overrightarrow{AB}.\overrightarrow{AB}=AB^2\)
\(\overrightarrow{AC}.\overrightarrow{BC}=\overrightarrow{AC}.\left(\overrightarrow{BA}+\overrightarrow{AC}\right)=\overrightarrow{AC}.\overrightarrow{BA}+\overrightarrow{AC}.\overrightarrow{AC}=AC^2\)
\(BC=\sqrt{AB^2+AC^2}=\sqrt{9+16}=5\).