K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
15 tháng 6 2023
b: C=sin(x-pi/2+2pi)+sin(x+pi)+cos(pi/2-x)+cos(pi/2+x)
=sin(x-pi/2)+sin(x+pi)+sinx+cos(pi/2+x)
=-sin(pi/2-x)-sinx+sinx-sinx=-cosx-sinx
NV
Nguyễn Việt Lâm
Giáo viên
10 tháng 5 2021
b.
\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x< 2\\x>\dfrac{9}{2}\end{matrix}\right.\\-\dfrac{1}{3}< x< 7\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}-\dfrac{1}{3}< x< 2\\\dfrac{9}{2}< x< 7\end{matrix}\right.\)
Hay \(S=\left(-\dfrac{1}{3};2\right);\left(\dfrac{9}{2};7\right)\)
d.
\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x\le-\dfrac{11}{5}\\x\ge7\end{matrix}\right.\\-\dfrac{1}{2}< x< 3\end{matrix}\right.\) \(\Rightarrow x\in\varnothing\) hay BPT vô nghiệm
NV
Nguyễn Việt Lâm
Giáo viên
1 tháng 4 2021
a. \(sinx+cosx=\dfrac{1}{5}\Rightarrow\left(sinx+cosx\right)^2=\dfrac{1}{25}\Rightarrow sin^2x+cos^2x+2sinx.cosx=\dfrac{1}{25}\)
\(\Rightarrow1+2sinx.cosx=\dfrac{1}{25}\Rightarrow sinx.cosx=-\dfrac{12}{25}\)
\(P=tanx+cotx=\dfrac{sinx}{cosx}+\dfrac{cosx}{sinx}=\dfrac{sin^2x+cos^2x}{sinx.cosx}=\dfrac{1}{sinx.cosx}=\dfrac{1}{-\dfrac{12}{25}}=-\dfrac{25}{12}\)
b. \(\left(tana-cota\right)^2=\left(2\sqrt{3}\right)^2\Leftrightarrow\left(tana+cota\right)^2-4tana.cota=12\)
\(\Rightarrow\left(tana+cota\right)^2-4=12\Rightarrow\left(tana+cota\right)^2=16\)
\(\Rightarrow\left|tana+cota\right|=4\)
NV
Nguyễn Việt Lâm
Giáo viên
30 tháng 4 2021
Hàm xác định trên R khi và chỉ khi:
\(\left\{{}\begin{matrix}a=2>0\\\Delta'=m^2-2m\le0\end{matrix}\right.\)
\(\Leftrightarrow0\le m\le2\)
\(\Rightarrow m_{max}=2\) ; \(m_{min}=0\)
NV
Nguyễn Việt Lâm
Giáo viên
30 tháng 4 2021
\(f\left(x\right)=\dfrac{x+4}{\left(x-3\right)\left(x+3\right)}-\dfrac{2}{x+3}+\dfrac{4x}{x\left(x-3\right)}\)
\(f\left(x\right)=\dfrac{x\left(x+4\right)}{x\left(x-3\right)\left(x+3\right)}-\dfrac{2x\left(x-3\right)}{x\left(x+3\right)\left(x-3\right)}+\dfrac{4x\left(x+3\right)}{x\left(x-3\right)\left(x+3\right)}\)
\(f\left(x\right)=\dfrac{3x^2+22x}{x\left(x-3\right)\left(x+3\right)}\)
\(f\left(x\right)< 0\Leftrightarrow\left\{{}\begin{matrix}x< -\dfrac{22}{3}\\-3< x< 0\\0< x< 3\end{matrix}\right.\) \(\Rightarrow x_{max}=2\)
7 tháng 1 2022
c: \(\Leftrightarrow x^2-5x-x^2-7< =0\)
=>-5x<=7
hay x>=-7/5
d: \(\Leftrightarrow x^2-x-2+3-x^2>=0\)
=>-x+1>=0
=>-x>=-1
hay x<=1
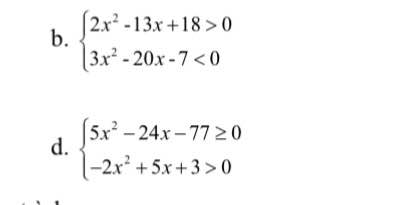
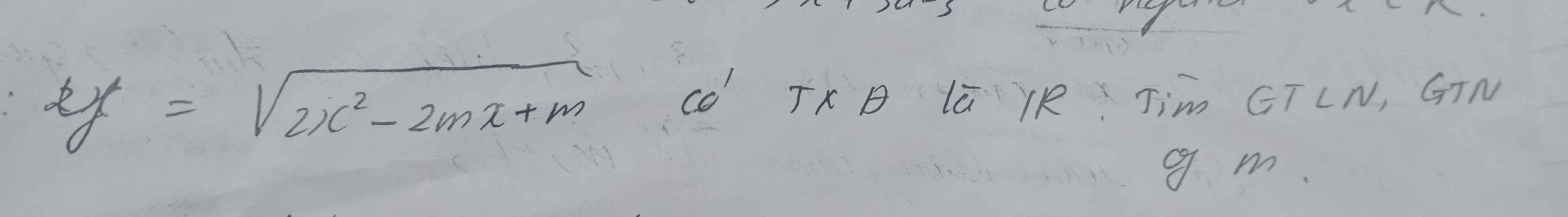
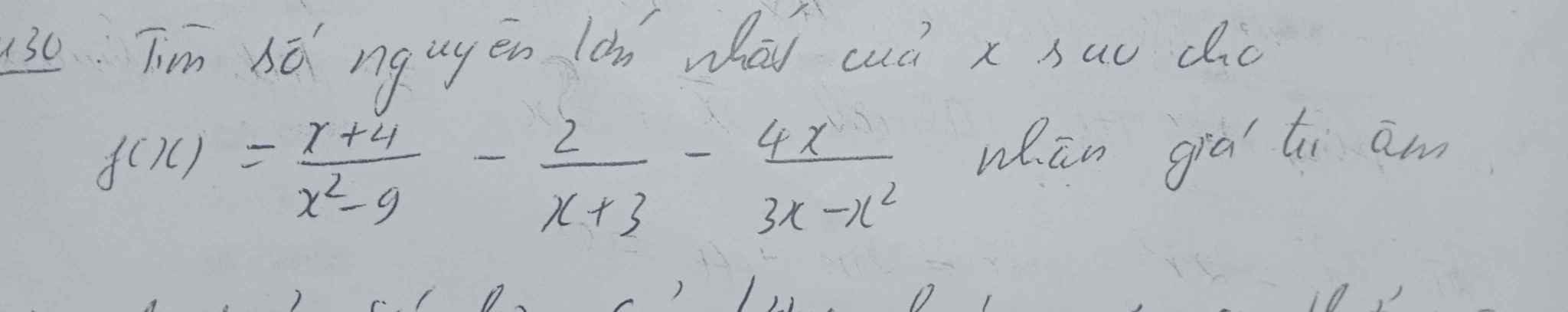
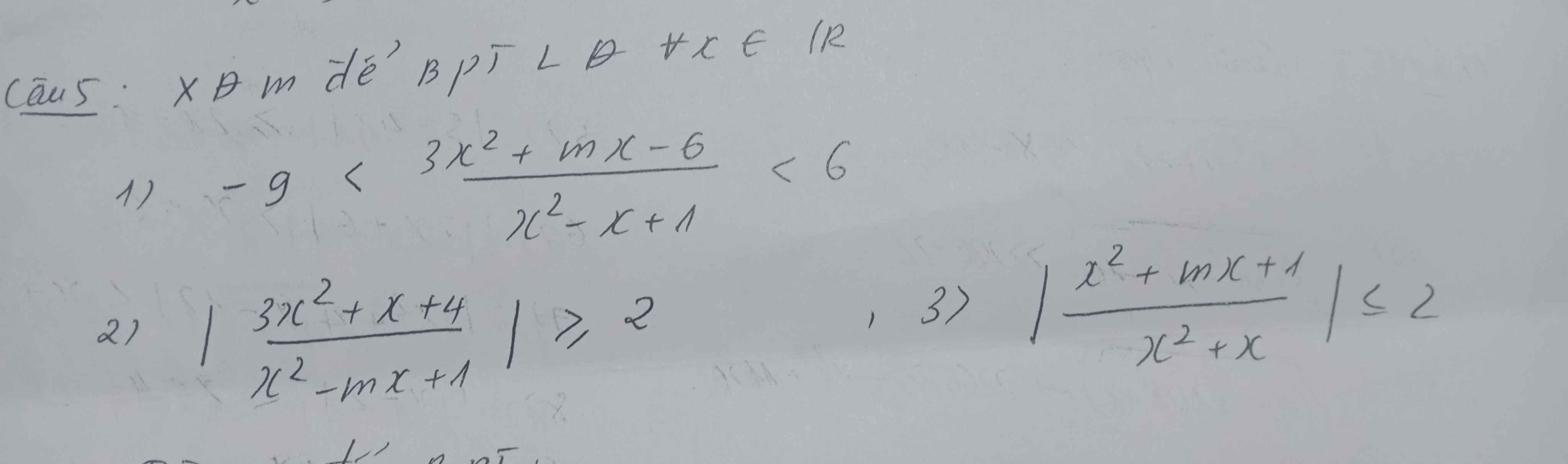
\(D=\dfrac{sin2x+2\cdot cos4x\cdot sinx}{cosx+cos4x}=\dfrac{2\cdot sinx\left(cosx+cos4x\right)}{cosx+cos4x}=2\cdot sinx\)
b: C=sin(x-pi/2+2pi)+sin(x+pi)+cos(pi/2-x)+cos(pi/2+x)
=sin(x-pi/2)+sin(x+pi)+sinx+cos(pi/2+x)
=-sin(pi/2-x)-sinx+sinx-sinx=-cosx-sinx