TP
4
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
DN
3
AH
Akai Haruma
Giáo viên
30 tháng 10 2021
Bài 1:
a. $=2x(x-3)$
b. $=x^3(x+3)+(x+3)=(x^3+1)(x+3)=(x+1)(x^2-x+1)(x+3)$
c. $=64-(x^2-2xy+y^2)=8^2-(x-y)^2$
$=(8-x+y)(8+x-y)$
AH
Akai Haruma
Giáo viên
30 tháng 10 2021
Bài 2:
$(x+5)(x+1)+(x-2)(x^2+2x+4)-x(x^2+x-2)$
$=x^2+6x+5+(x^3-2^3)-(x^3+x^2-2x)$
$=x^2+6x+5+x^3-8-x^3-x^2+2x$
$=8x-3$
Ta có đpcm.
NT
0
1 tháng 10 2021
(3x-4-x-1)(3x-4+x+1)=0
(2x-5)(4x-3)=0
2x-5 = 0 hoặc 4x-3=0
2x=5 hoặc 4x=3
x=5/2 hoặc x=3/4
MA
1
30 tháng 9 2021
a) Đặt \(a=x^2+x\)
Đa thức trở thành: \(a^2-14a+24=\left(a^2-14a+49\right)-25=\left(a-7\right)^2-25=\left(a-7-5\right)\left(a-7+5\right)=\left(a-12\right)\left(a-2\right)\)
Thay a:
\(\left(a-12\right)\left(a-2\right)=\left(x^2+x-12\right)\left(x^2+x-2\right)\)
b) Đặt \(a=x^2+x\)
Đa thức trở thành:
\(\left(x^2+x\right)^2+4x^2+4x-12=\left(x^2+x\right)^2+4\left(x^2+x\right)-12=a^2+4a-12=\left(a^2+4x+4\right)-16=\left(a+2\right)^2-16=\left(a+2-4\right)\left(a+2+4\right)=\left(a-2\right)\left(a+6\right)\)
Thay a:
\(\left(a-2\right)\left(a+6\right)=\left(x^2+x-2\right)\left(x^2+x+6\right)\)
24 tháng 12 2021
Bài 2:
a: \(\Leftrightarrow4x^2-20x+25-4x^2+12x=0\)
=>-8x=-25
hay x=25/8
15 tháng 6 2022
1.
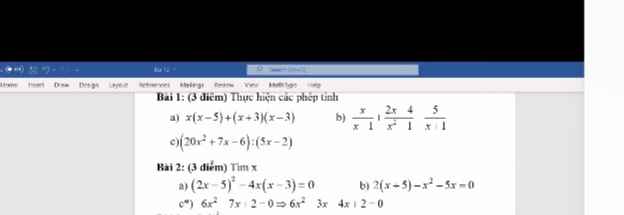
a) x (x - 5) + (x + 3)(x - 3)=
= x^2 - 5x + (x + 3)(x - 3)
= x^2 - 5x + x^2 - 9
= x^2 + x^2 - 5x - 9
= 2x^2 - 5x - 9.
b. không thể nhìn thấy hết bài được. Nó bị mất dấu!!
c. (20x^2 + 7x - 6) : (5x - 2)
= (5x - 2) (4x + 3) : (5x - 2)
= 4x + 3.
2.
a. (2x - 5)^2 - 4x (x - 3)= 0
-8x + 25= 0
-8x + 25 - 25= 0 - 25
-8x= -25
-8x : 8= -25 : 8
x = 25/8
Vậy x= 25/8
b. 2(x - 5) - x^2 - 5x= 0
-10x= 0
-10x : (-10)= 0 : (-10)
x= 0
Vậy x= 0
c. Lí do cũng giống câu b bài 1.
QN
3
5 tháng 9 2021
\(a,A=x^2-6x-2=\left(x-3\right)^2-11\ge-11\)
Dấu \("="\Leftrightarrow x=3\)
\(b,B=6x-9x^2+2=-\left(3x-1\right)^2+3\le3\)
Dấu \("="\Leftrightarrow x=\dfrac{1}{3}\)
17 tháng 9 2021
Gọi E là giao điểm của AC và BD.
∆ECD có ∠C1 = ∠D1 (do ∠ACD = ∠BDC) nên là tam giác cân.
Suy ra EC = ED (1)
Tương tự ∆EAB cân tại A suy ra: EA = EB (2)
Từ (1) và (2) ta có: EA + EC = EB + ED ⇒ AC = BD
Hình thang ABCD có hai đường chéo bằng nhau nên là hình thang cân.4
6 tháng 2 2021
a) Ta có: \(\left(2x-3\right)\left(3x+4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-3=0\\3x+4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=3\\3x=-4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{3}{2}\\x=-\dfrac{4}{3}\end{matrix}\right.\)
Vậy: \(S=\left\{\dfrac{3}{2};-\dfrac{4}{3}\right\}\)
b) Ta có: \(x^3-3x^2+3x-1=\left(x-1\right)\left(x+1\right)\)
\(\Leftrightarrow\left(x-1\right)^3-\left(x-1\right)\left(x+1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left[x^2-2x+1-x-1\right]=0\)
\(\Leftrightarrow\left(x-1\right)\left(x^2-3x\right)=0\)
\(\Leftrightarrow x\left(x-1\right)\left(x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x-1=0\\x-3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\\x=3\end{matrix}\right.\)
Vậy: S={0;1;3}
c) Ta có: \(x^2+x=2x+2\)
\(\Leftrightarrow x\left(x+1\right)-2\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+1=0\\x-2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=2\end{matrix}\right.\)
Vậy: S={-1;2}
d) Ta có: \(\left(x-1\right)^2=2\left(x^2-1\right)\)
\(\Leftrightarrow\left(x-1\right)^2-2\left(x-1\right)\left(x+1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x-1-2x-2\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(-x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\-x-3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\-x=3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-3\end{matrix}\right.\)Vậy: S={1;-3}
e) Ta có: \(2\left(x+2\right)^2-x^3-8=0\)
\(\Leftrightarrow2\left(x+2\right)^2-\left(x^3+8\right)=0\)
\(\Leftrightarrow2\left(x+2\right)\cdot\left(x+2\right)-\left(x+2\right)\left(x^2-2x+4\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(2x+4-x^2+2x-4\right)=0\)
\(\Leftrightarrow\left(x+2\right)\cdot\left(-x^2+4x\right)=0\)
\(\Leftrightarrow-x\left(x+2\right)\left(x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x+2=0\\x-4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-2\\x=4\end{matrix}\right.\)
Vậy: S={0;-2;4}
Lần sau bn nhớ bổ sung thêm đề nhé! Lần này mình sẽ xem như đề là tìm GTLN
\(12x-4x^2+9=-\left(4x^2-12x+9\right)+18=-\left(2x-3\right)^2+18\le18\)
Dấu "=" xảy ra \(\Leftrightarrow x=\dfrac{3}{2}\)
Xin lỗi bạn nha đề của mình là phân tích đa thức thành nhân tử. Sorry bạn!