Giúp mình với ạ! Cần rất rất rất gấp ấy ạ!
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
9 tháng 10 2021
Bài 1:
a) \(=\dfrac{\sqrt{5}.\sqrt{7}}{5}=\dfrac{\sqrt{35}}{5}\)
b) \(=\dfrac{\left|y\right|}{\sqrt{3}}=\dfrac{\sqrt{3}y}{3}\)
c) \(=\dfrac{\sqrt{2}}{\sqrt{t}}=\dfrac{\sqrt{2t}}{t}\)
d) \(=\sqrt{\dfrac{7p^2-3p^2}{7}}=\sqrt{\dfrac{4p^2}{7}}=\dfrac{2\left|p\right|}{\sqrt{7}}=\dfrac{-2\sqrt{7}p}{7}\)
Bài 2:
a) \(=\dfrac{\sqrt{21}-\sqrt{15}}{3}\)
b) \(=\dfrac{10\left(4+3\sqrt{2}\right)}{16-18}=-20-15\sqrt{2}\)
c) \(=\dfrac{\left(3\sqrt{10}-5\right)\left(6+\sqrt{10}\right)}{36-10}=\dfrac{18\sqrt{10}+30-30-5\sqrt{10}}{26}=\dfrac{13\sqrt{10}}{26}=\dfrac{\sqrt{10}}{2}\)
PM
1
27 tháng 8 2021
a: Áp dụng định lí Pytago vào ΔBAC vuông tại A, ta được:
\(BC^2=AB^2+AC^2\)
\(\Leftrightarrow BC^2=10^2+15^2=325\)
hay \(BC=5\sqrt{13}\left(cm\right)\)
Xét ΔBAC vuông tại A có
\(\sin\widehat{B}=\dfrac{AC}{BC}=\dfrac{15}{5\sqrt{13}}=\dfrac{3}{\sqrt{13}}\)
\(\Leftrightarrow\widehat{B}\simeq56^0\)
b: Xét ΔBAC có
BI là đường phân giác ứng với cạnh AC
nên \(\dfrac{AI}{AB}=\dfrac{CI}{BC}\)
hay \(\dfrac{AI}{10}=\dfrac{CI}{5\sqrt{13}}\)
mà AI+CI=15cm
nên Áp dụng tính chất của dãy tỉ số bằng nhau, ta được:
\(\dfrac{AI}{10}=\dfrac{CI}{5\sqrt{13}}=\dfrac{AI+CI}{10+5\sqrt{13}}=\dfrac{15}{10+5\sqrt{13}}=\dfrac{-2+\sqrt{13}}{3}\)
Do đó: \(AI=\dfrac{-20+10\sqrt{13}}{3}\left(cm\right)\)
E
1
8 tháng 8 2021
ta có sinB=\(\dfrac{AH}{AB}\)\(\Rightarrow\)AH=AB.sinB=3,6.sin62=3,18
BH=\(\sqrt{AB^2-AH^2}\)(pytago)=\(\sqrt{3,6^2-3,18^2}\)=1,69
\(_{\widehat{C}}\)=90-\(\widehat{B}\)=90-62=28\(^0\)
sinC=\(\dfrac{AB}{BC}\)\(\Rightarrow\)BC=\(\dfrac{AB}{sinC}\)=\(\dfrac{3,6}{sin28}\)=7,67
mà:CH=BC-BH=7,67-1,69=5,98
AC=\(\sqrt{BC^2-AB^2}\)(pytago)=\(\sqrt{7,67^2-3,6^2}\)=6.77
AH
0
13 tháng 1
Bài 5:
Thay x=1 và y=2 vào hệ phương trình, ta được:
\(\left\{{}\begin{matrix}-m\cdot1+2=-2m\\1+m^2\cdot2=9\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}-2m=-m+2\\2m^2=8\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m^2=4\\-m=2\end{matrix}\right.\)
=>m=-2
Bài 6:
a: ĐKXĐ: x>=1 và y>=-2
\(\left\{{}\begin{matrix}\sqrt{x-1}-3\sqrt{y+2}=2\\2\sqrt{x-1}+5\sqrt{y+2}=15\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2\sqrt{x-1}-6\sqrt{y+2}=4\\2\sqrt{x-1}+5\sqrt{y+2}=15\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}-11\sqrt{y+2}=-11\\\sqrt{x-1}-3\sqrt{y+2}=2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\sqrt{y+2}=1\\\sqrt{x-1}=2+3=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y+2=1\\x-1=25\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=26\\y=-1\end{matrix}\right.\left(nhận\right)\)
b: ĐKXĐ: x<>0 và y<>0
\(\left\{{}\begin{matrix}\dfrac{1}{x}+\dfrac{1}{y}=\dfrac{1}{12}\\\dfrac{8}{x}+\dfrac{15}{y}=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\dfrac{8}{x}+\dfrac{8}{y}=\dfrac{8}{12}=\dfrac{2}{3}\\\dfrac{8}{x}+\dfrac{15}{y}=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-\dfrac{7}{y}=\dfrac{-1}{3}\\\dfrac{1}{x}+\dfrac{1}{y}=\dfrac{1}{12}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=21\\\dfrac{1}{x}=\dfrac{1}{12}-\dfrac{1}{21}=\dfrac{7-4}{84}=\dfrac{3}{84}=\dfrac{1}{28}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=28\\y=21\end{matrix}\right.\left(nhận\right)\)
c: ĐKXĐ: x<>0 và y<>2
\(\left\{{}\begin{matrix}\dfrac{2}{x}+\dfrac{3}{y-2}=4\\\dfrac{4}{x}-\dfrac{1}{y-2}=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\dfrac{4}{x}+\dfrac{6}{y-2}=8\\\dfrac{4}{x}-\dfrac{1}{y-2}=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{7}{y-2}=7\\\dfrac{2}{x}+\dfrac{3}{y-2}=4\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y-2=1\\\dfrac{2}{x}=4-\dfrac{3}{1}=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=3\end{matrix}\right.\left(nhận\right)\)
d: ĐKXĐ: x<>-2y và x<>-y/2
\(\left\{{}\begin{matrix}\dfrac{2}{x+2y}+\dfrac{1}{2x+y}=3\\\dfrac{4}{x+2y}-\dfrac{3}{2x+y}=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\dfrac{6}{x+2y}+\dfrac{3}{2x+y}=9\\\dfrac{4}{x+2y}-\dfrac{3}{2x+y}=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{10}{x+2y}=10\\\dfrac{4}{x+2y}-\dfrac{3}{2x+y}=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x+2y=1\\\dfrac{3}{2x+y}=4-1=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x+2y=1\\2x+y=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2x+4y=2\\2x+y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3y=1\\x+2y=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=\dfrac{1}{3}\\x=1-\dfrac{2}{3}=\dfrac{1}{3}\end{matrix}\right.\left(nhận\right)\)
e: ĐKXĐ: x>4 và y<>-2
\(\left\{{}\begin{matrix}\dfrac{3}{\sqrt{x-4}}+\dfrac{4}{y+2}=7\\\dfrac{5}{\sqrt{x-4}}-\dfrac{1}{y+2}=4\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\dfrac{3}{\sqrt{x-4}}+\dfrac{4}{y+2}=7\\\dfrac{20}{\sqrt{x-4}}-\dfrac{4}{y+2}=16\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{23}{\sqrt{x-4}}=23\\\dfrac{5}{\sqrt{x-4}}-\dfrac{1}{y+2}=4\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\sqrt{x-4}=1\\\dfrac{1}{y+2}=5-4=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x-4=1\\y+2=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=5\\y=-1\end{matrix}\right.\left(nhận\right)\)
f: ĐKXĐ: x>=-1
\(\left\{{}\begin{matrix}2\left(x+y\right)+\sqrt{x+1}=4\\\left(x+y\right)-\sqrt{x+1}=-5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2\left(x+y\right)+\sqrt{x+1}+\left(x+y\right)-\sqrt{x+1}=4-5=-1\\\left(x+y\right)-\sqrt{x+1}=-5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}3\left(x+y\right)=-1\\\sqrt{x+1}=-\dfrac{1}{3}+5=\dfrac{14}{3}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x+y=-\dfrac{1}{3}\\x+1=\dfrac{196}{9}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{187}{9}\\y=-\dfrac{1}{3}-\dfrac{187}{9}=-\dfrac{190}{9}\end{matrix}\right.\left(nhận\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
13 tháng 1
Nhiều quá em, em chỉ nên đăng những câu nào cảm thấy khó khăn khi giải quyết thôi
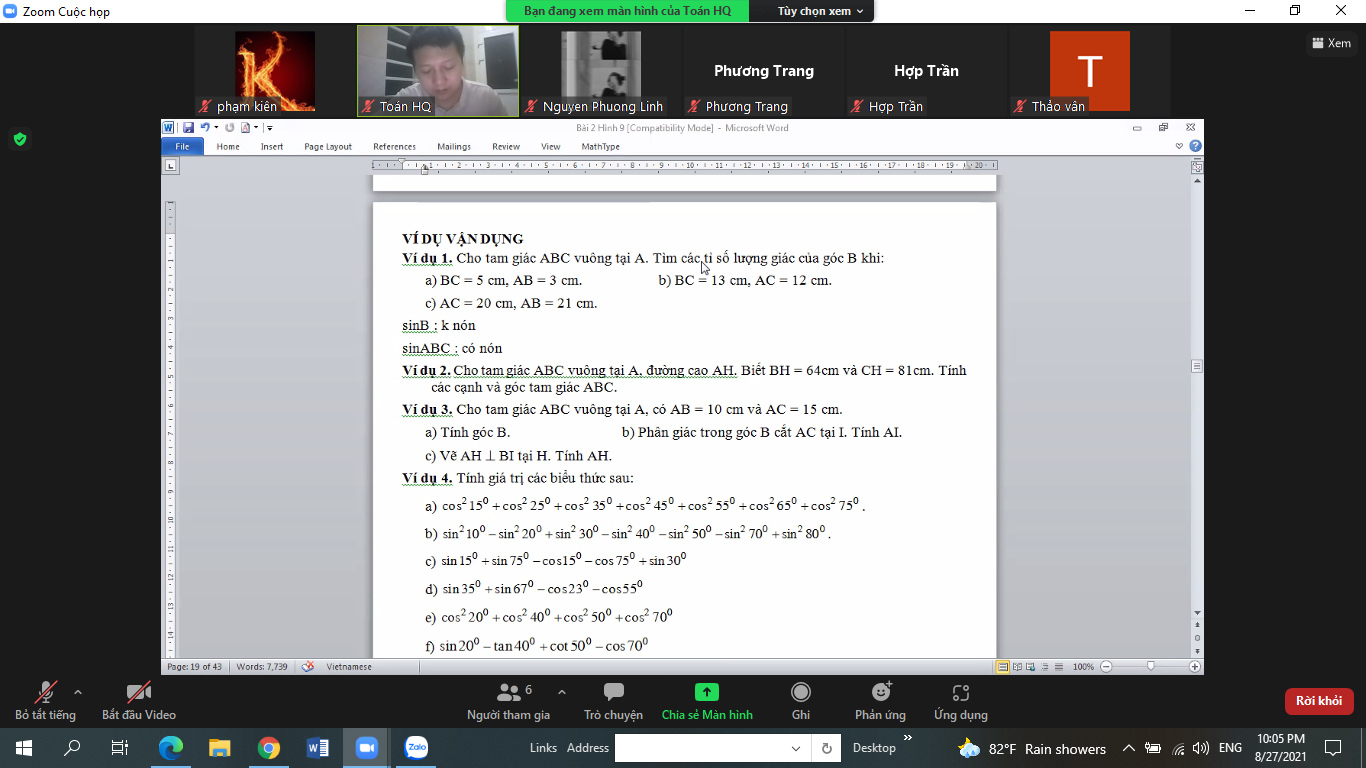
Ai giúp em với ạ em cần rất gấp bài 4 thôi ạ
HK
1
Ta có : \(\hept{\begin{cases}BD^2=BA^2+AD^2\\BC^2=\left(DC-AB\right)^2+AD^2\end{cases}\Leftrightarrow\hept{\begin{cases}225=BA^2+AD^2\\169=\left(14-AB\right)^2+AD^2\end{cases}}}\)
lấy hiệu ta có : \(56=28AB-196\Leftrightarrow AB=9\Rightarrow AD=\sqrt{225-9^2}=12\)
Diện tích hình thang là :\(\frac{1}{2}\left(AB+CD\right)\times AD=\frac{1}{2}\left(9+14\right)\times12=138cm^2\)