
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


2:
1+cot^2a=1/sin^2a
=>1/sin^2a=1681/81
=>sin^2a=81/1681
=>sin a=9/41
=>cosa=40/41
tan a=1:40/9=9/40

Bạn nên chịu khó gõ đề ra khả năng được giúp sẽ cao hơn.
Câu h của em đây nhé
h, ( 1 + \(\dfrac{3-\sqrt{3}}{\sqrt{3}-1}\)).(1 - \(\dfrac{3+\sqrt{3}}{\sqrt{3}+1}\))
= \(\dfrac{\sqrt{3}-1+3-\sqrt{3}}{\sqrt{3}-1}\).\(\dfrac{\sqrt{3}+1-3-\sqrt{3}}{\sqrt{3}+1}\)
= \(\dfrac{2}{\sqrt{3}-1}\).\(\dfrac{-2}{\sqrt{3}+1}\)
= \(\dfrac{-4}{2}\)
= -2

a, \(2\sqrt{3}-\sqrt{4+x^2}=0\Leftrightarrow\sqrt{4+x^2}=2\sqrt{3}\)
\(\Leftrightarrow x^2+4=12\Leftrightarrow x^2=8\Leftrightarrow x=\pm2\sqrt{2}\)
b, \(\sqrt{16x+16}-\sqrt{9x+9}=0\)ĐK : x >= -1
\(\Leftrightarrow4\sqrt{x+1}-3\sqrt{x+1}=0\Leftrightarrow\sqrt{x+1}=0\Leftrightarrow x+1=0\Leftrightarrow x=-1\)
c, \(\sqrt{4\left(x+2\right)^2}=8\Leftrightarrow2\left|x+2\right|=8\Leftrightarrow\left|x+2\right|=4\)
TH1 : \(x+2=4\Leftrightarrow x=2\)
TH2 : \(x+2=-4\Leftrightarrow x=-6\)
c: Ta có: \(\sqrt{4\left(x+2\right)^2}=8\)
\(\Leftrightarrow\left|x+2\right|=4\)
\(\Leftrightarrow\left[{}\begin{matrix}x+2=4\\x+2=-4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-6\end{matrix}\right.\)

phương trình nghiệm nguyên có nhiều dạng và nhiều cách làm lắm bạn ạ, bạn hỏi cụ thể bài nào để mình còn xem chứ
mua quyển "pt nghiệm nguyên và kinh nghiệm giải" ,muốn dạng nào cũng có

a: Khi m=2 thì (1) sẽ là:
2x+y=2 và 4x+3y=10
=>x=-2 và y=6
b: 2x+y=m và 4x+3y=10
=>4x+2y=2m và 4x+3y=10
=>4x+3y=10 và 4x+2y=2m
=>y=10-2m và 2x=m-10+2m=3m-10
=>y=10-2m và x=3/2m-5
x>0 và y>0
=>10-2m>0 và 3/2m-5>0
=>m>5:3/2=10/3 và m<5
=>10/3<m<5

a,\(\Delta=3^2-4\left(-2\right).6=9+48=57\)
\(x_1=\dfrac{-3+\sqrt{57}}{-4}=\dfrac{3-\sqrt{57}}{4}\)
\(x_2=\dfrac{-3-\sqrt{57}}{-4}=\dfrac{3+\sqrt{57}}{4}\)
b, \(\Delta=6^2-4.3.3=36-36=0\)
\(\Rightarrow x_1=x_2=\dfrac{-6}{2.3}=\dfrac{-6}{6}=-1\)
c, \(\Delta=1^2-4.6.5=1-120=-119< 0\)
Vậy pt vô nghiệm
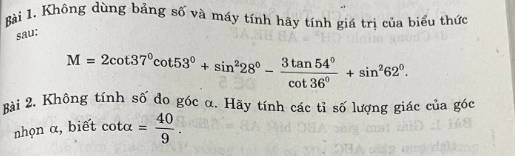
 Mọi người giúp mình với, giải chi tiết (+giải thích giúp mình nha), cảm ơn mọi người rất nhiều lun =)
Mọi người giúp mình với, giải chi tiết (+giải thích giúp mình nha), cảm ơn mọi người rất nhiều lun =)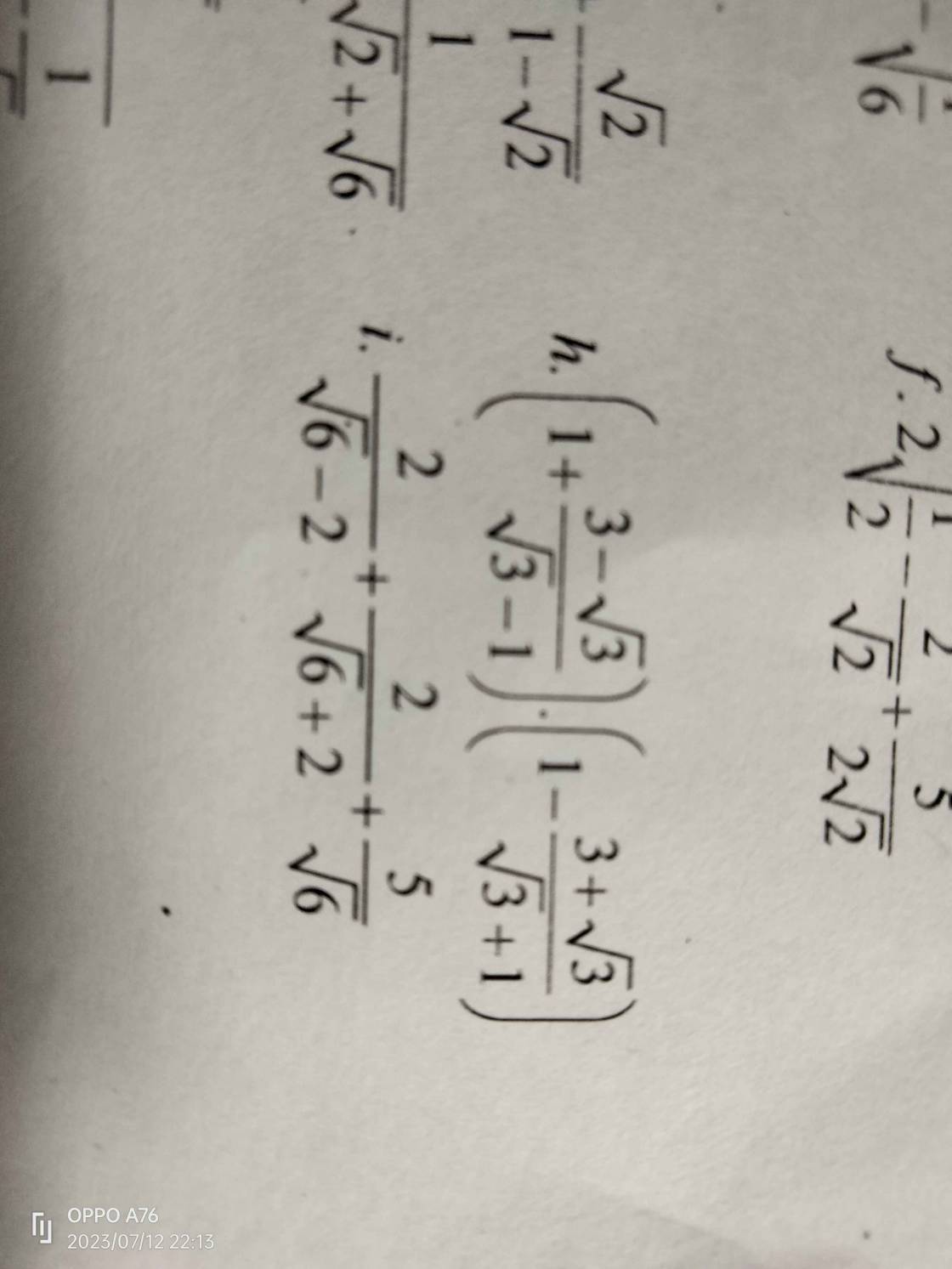
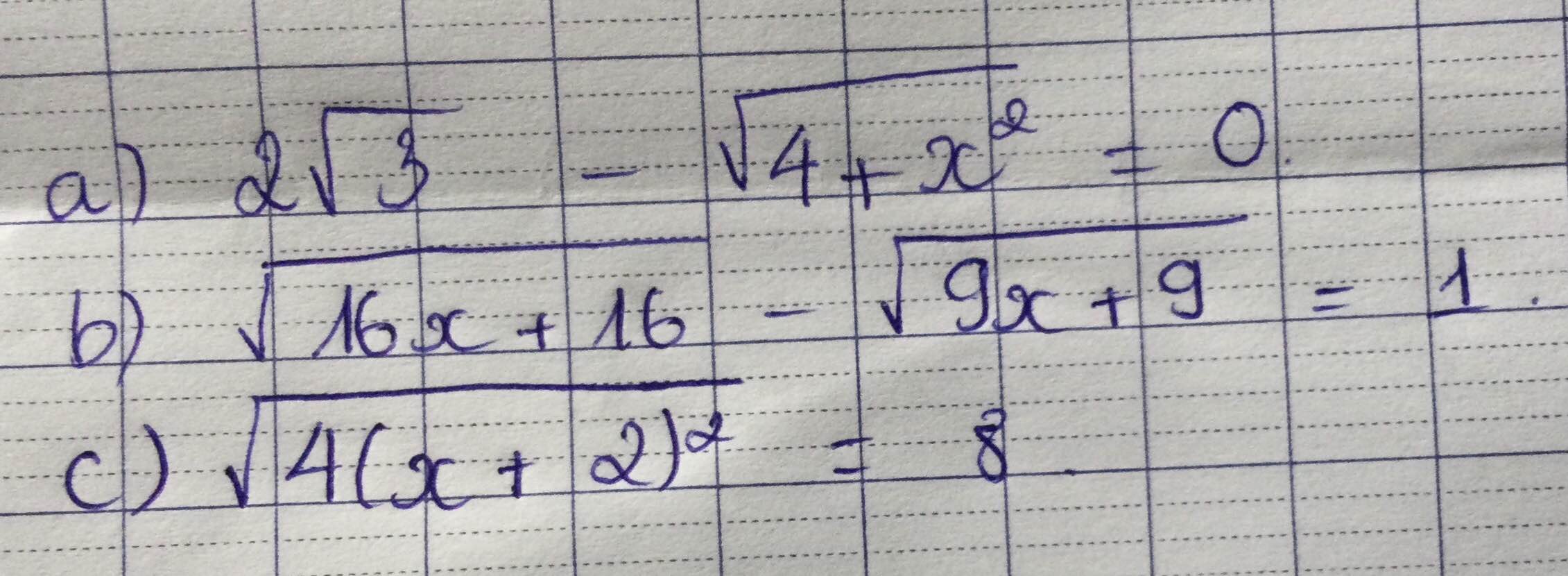 Các bạn ơi giúp mk với. Mk cần gấp lắm ạ.Các bạn giải chi tiết giúp mk nhé . Mk hứa sẽ vote 5* cho . Mk cảm ơn nhiều.
Các bạn ơi giúp mk với. Mk cần gấp lắm ạ.Các bạn giải chi tiết giúp mk nhé . Mk hứa sẽ vote 5* cho . Mk cảm ơn nhiều.
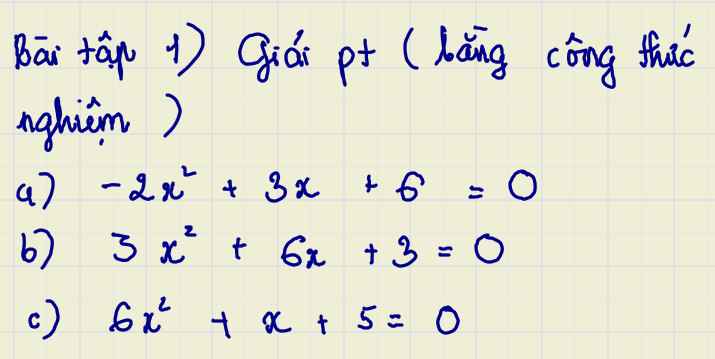
bạn đánh dâu cho mik nha
góc nào vuông vậy bạn:))