K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
9 tháng 12 2021
Câu 1:
\(a,=2x^4-6x^2\\ b,=3y^2+2\)
Câu 2:
\(\widehat{C}=360^0-55^0-80^0-120^0=105^0\\ \Rightarrow\text{Góc ngoài tại }\widehat{C}=180^0-105^0=75^0\)
Câu 3:
\(a,AM=\dfrac{BC}{2}=\dfrac{\sqrt{AB^2+AC^2}}{2}=\dfrac{15}{2}\left(cm\right)\\ b,S_{ABC}=\dfrac{1}{2}AB\cdot AC=\dfrac{1}{2}\cdot12\cdot9=54\left(cm^2\right)\)
Câu 4:
\(a,=\dfrac{x^2-4x+4}{x-2}=\dfrac{\left(x-2\right)^2}{x-2}=x-2\\ b,=\dfrac{x+1+2x+3-4+x}{6x^2y}=\dfrac{4x}{6x^2y}=\dfrac{2}{3xy}\)
NK
0
9 tháng 7 2017
Ta có : |2x - 5| + |4 + x| = 0
Mà : |2x - 5| \(\ge0\forall x\)
|4 + x| \(\ge0\forall x\)
Nên \(\orbr{\begin{cases}\left|2x-5\right|=0\\\left|4+x\right|=0\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}2x-5=0\\4+x=0\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}2x=5\\x=-4\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=\frac{5}{2}\\x=-4\end{cases}}\)
18 tháng 8 2021
Ta có: \(\left(3x-1\right)\left(2x+7\right)-\left(x+1\right)\left(6x-5\right)=\left(x+2\right)-\left(x-5\right)\)
\(\Leftrightarrow6x^2+21x-2x-7-6x^2+5x-6x+5=x+2-x+5\)
\(\Leftrightarrow18x-2=7\)
\(\Leftrightarrow18x=9\)
hay \(x=\dfrac{1}{2}\)
23 tháng 10 2021
\(b,N=\left(2x-1\right)^2-4\ge-4\\ N_{min}=-4\Leftrightarrow x=\dfrac{1}{2}\\ c,P=\left(2x-5\right)^2+6\left(2x-5\right)+9-4\\ P=\left(2x-5+3\right)^2-4=\left(2x-2\right)^2-4\ge-4\\ P_{min}=-4\Leftrightarrow x=1\\ d,Q=\left(x^2-2x+1\right)+\left(y^2+4y+4\right)+1\\ Q=\left(x-1\right)^2+\left(y+2\right)^2+1\ge1\\ Q_{min}=1\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=-2\end{matrix}\right.\)
AH
Akai Haruma
Giáo viên
23 tháng 10 2021
6a.
$M=x^2-x+1=(x^2-x+\frac{1}{4})+\frac{3}{4}$
$=(x-\frac{1}{2})^2+\frac{3}{4}\geq \frac{3}{4}$
Vậy $M_{\min}=\frac{3}{4}$ khi $x-\frac{1}{2}=0\Leftrightarrow x=\frac{1}{2}$
27 tháng 2 2022
7, ĐKXĐ:\(x\ne2\)
\(\dfrac{1}{x-2}+\dfrac{3-x}{2-x}=-3\\ \Leftrightarrow\dfrac{1}{x-2}+\dfrac{x-3}{x-2}=-3\\ \Leftrightarrow\dfrac{1+x-3}{x-2}=-3\\ \Rightarrow x-2=-3\left(x-2\right)\\ \Leftrightarrow x-2=6-3x\\ \Leftrightarrow x-2-6+3x=0\\ \Leftrightarrow4x-8=0\\ \Leftrightarrow x=2\left(ktm\right)\)
27 tháng 2 2022
7: \(\Leftrightarrow\dfrac{1}{x-2}+\dfrac{x-3}{x-2}=\dfrac{-3\left(x-2\right)}{x-2}\)
\(\Leftrightarrow x-2=-3\left(x-2\right)\)
=>x-2=0
hay x=2(loại)
8: \(\Leftrightarrow\left(x+5\right)^2-\left(x-5\right)^2=20\)
\(\Leftrightarrow x^2+10x+25-x^2+10x-25=20\)
=>20x=20
hay x=1(nhận)
NH
22 tháng 2 2023
`x^3 -2x-x+2=0`
`<=> (x^3 -x)-(2x-2)=0`
`<=> x(x^2 -1)-2(x-1)=0`
`<=> x(x-1)(x+1)-2(x-1)=0`
`<=> (x-1)(x^2 +x-2)=0`
`<=> (x-1)(x^2 +2x-x-2)=0`
`<=> (x-1)[x(x+2)-(x+2)]=0`
`<=> (x-1)(x+2)(x-1)=0`
`<=> (x-1)^2 (x+2)=0`
\(< =>\left[{}\begin{matrix}x-1=0\\x+2=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=1\\x=-2\end{matrix}\right.\)
28 tháng 9 2016
Câu 1:\(x^2.y+x.y^2-x-y=x.\left(x.y-1\right)+y.\left(x.y-1\right)=\left(x+y\right).\left(x.y-1\right)\)
Câu 3:\(a.x^2+a.y-b.x^2-b.y=x^2.\left(a-b\right)+y.\left(a-b\right)=\left(x^2+y\right).\left(a-b\right)\)
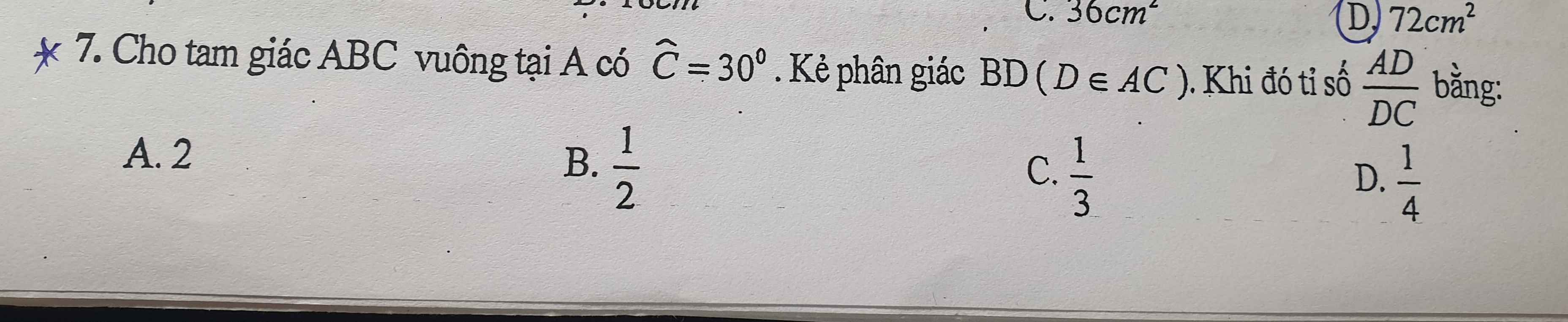
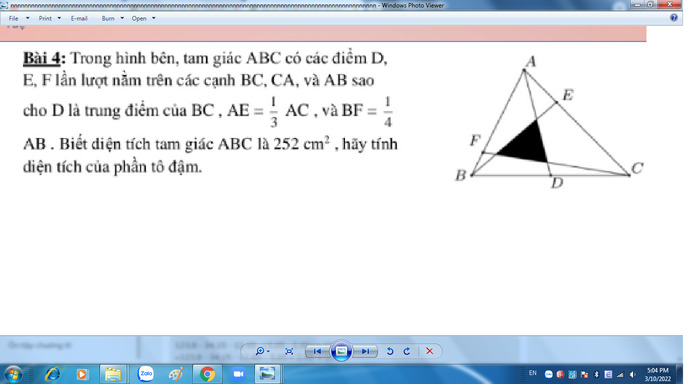
A nhá bạn
Bạn giải chi tiết cho mình với ạ