K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
3 tháng 2 2023
Câu 42:
Δ1: VTPT là (m;-1)
Δ2: VTPT là (2;-1)
Để Δ1 vuông góc Δ2 thì m*2+(-1)*(-1)=0
=>2m+1=0
=>m=-1/2
NL
1
PK
0
MH
13 tháng 5 2022
a) \(d\left(A;\Delta\right)=\dfrac{\left|4.1-3.3+2\right|}{\sqrt{4^2+\left(-3\right)^2}}=\dfrac{3}{5}\)
b) \(\overrightarrow{AB}=\left(-3;-2\right)\) là VTCP của đường thẳng d
PT tham số của d: \(\left\{{}\begin{matrix}x=1-3t\\y=3-2t\end{matrix}\right.\left(t\in R\right)\)
c) Đường tròn (C) có bán kính \(R=AB=\sqrt{\left(1+2\right)^2+\left(3-1\right)^2}=\sqrt{13}\)
PT đường tròn (C): \(\left(x-1\right)^2+\left(y-3\right)^2=13\)
JV
1
GN
GV Nguyễn Trần Thành Đạt
Giáo viên
22 tháng 11 2021
1b)
Song song => (d): x-y +a =0
Vì d đi qua C(2;-2) => 2- (-2)+a=0
<=>a=4
=> d: x-y+4=0
NV
Nguyễn Việt Lâm
Giáo viên
4 tháng 3 2022
\(sina.sinb=-\dfrac{1}{2}\left[cos\left(a+b\right)-cos\left(a-b\right)\right]\) là công thức đúng
1 tháng 8 2023
a: Gọi (d1): ax+by+c=0 là phương trình đường thẳng cần tìm
(d1) vuông góc (d)
=>(d1): 10x+4y+c=0
Thay x=2 và y=3 vào (d1), ta được:
c+20+12=0
=>c=-32
=>10x+4y-32=0
c: (d): x=1-2t và y=3+4t
=>VTCP là (-2;4)=(-1;2)
=>(d1) có VTPT (-1;2)
mà (d1) đi qua M(2;-3)
nên (d1): -1(x-2)+2(y+3)=0
=>-x+2+2y+6=0
=>-x+2y+8=0
b: (d1) vuông góc (d)
=>(d1): y=0x+b
Thay x=-1 và y=2 vào (d1), ta được:
0*(-1)+b=2
=>b=2
=>(d1): y=2
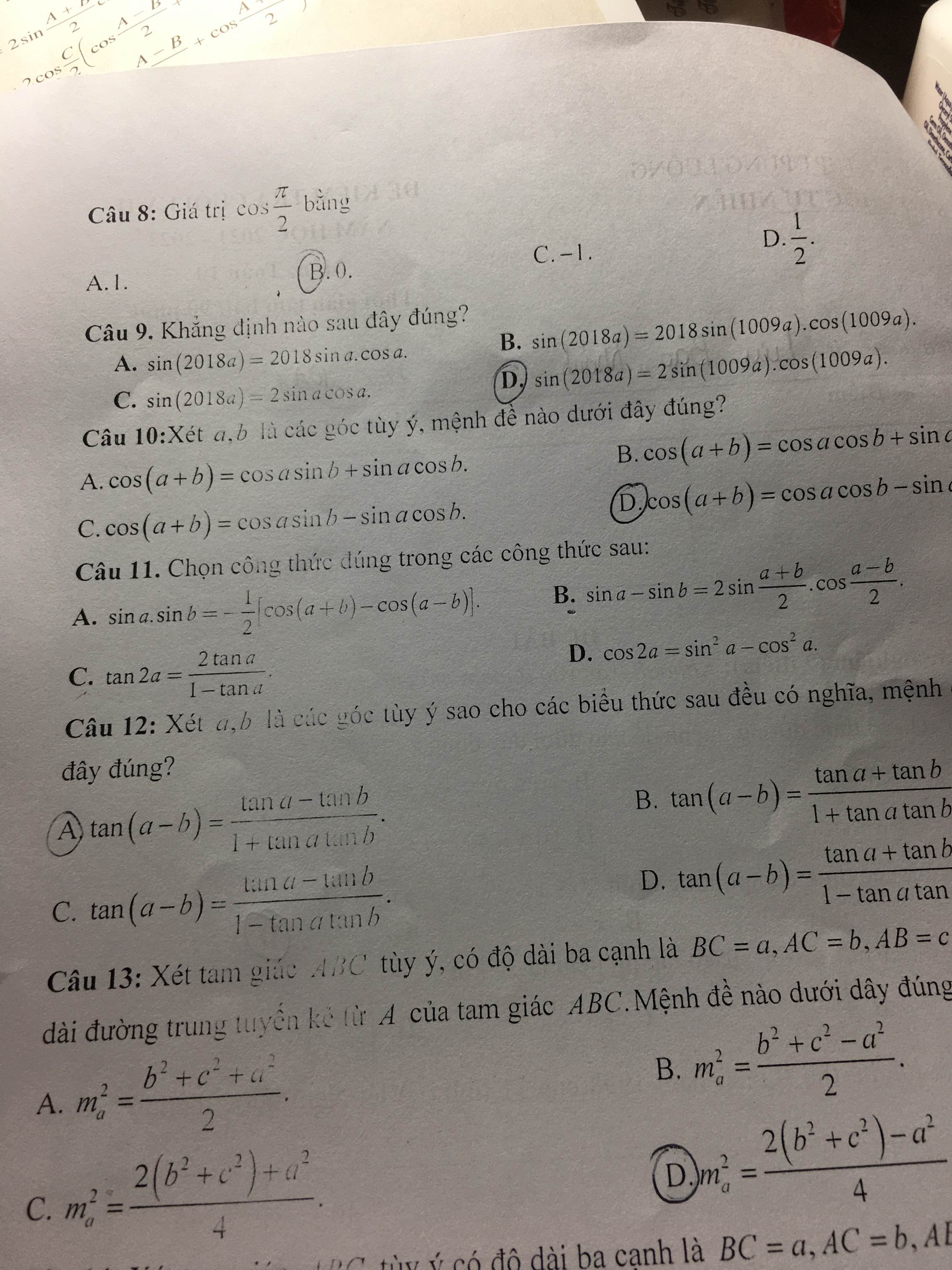
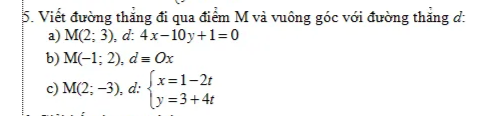
\(\overrightarrow{u}=4\overrightarrow{MA}-3\left(\overrightarrow{MA}+\overrightarrow{AB}\right)+\overrightarrow{MA}+\overrightarrow{AC}-2\left(\overrightarrow{MA}+\overrightarrow{AD}\right)\)
\(=-3\overrightarrow{AB}+\overrightarrow{AC}-2\overrightarrow{AD}=-3\left(\overrightarrow{AB}+\overrightarrow{AD}\right)+\overrightarrow{AC}+\overrightarrow{AD}\)
\(=-3\overrightarrow{AC}+\overrightarrow{AC}+\overrightarrow{AD}=-2\overrightarrow{AC}+\overrightarrow{AD}\)
\(\overrightarrow{u}^2=\left(-2\overrightarrow{AC}+\overrightarrow{AD}\right)^2=4AC^2-4\overrightarrow{AC}.\overrightarrow{AD}+AD^2\)
\(=4\left(a\sqrt{2}\right)^2-4.a\sqrt{2}.a.cos45^0+a^2=5a^2\)
\(\Rightarrow\left|\overrightarrow{u}\right|=a\sqrt{5}\)