
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1.
Do $a>1$ nên $a-1>0; 2a+1>0$. Khi đó
$A=\sqrt{(a-1)^2(2a+1)^2}=\sqrt{(a-1)^2}.\sqrt{(2a+1)^2}$
$=|a-1|.|2a+1|=(a-1)(2a+1)$
2.
$B=\sqrt{(b-1)(b+7)+16}=\sqrt{b^2+6b-7+16}=\sqrt{b^2+6b+9}$
$=\sqrt{(b+3)^2}=|b+3|=-(b+3)$ do $b+3<0$ với mọi $b< -3$

Bài 3:
Ta có: ΔMNK vuông tại M
nên \(\widehat{N}+\widehat{K}=90^0\)
hay \(\widehat{K}=30^0\)
Xét ΔMNK vuông tại M có
\(NK=\dfrac{MN}{\dfrac{1}{2}}=\dfrac{10}{\dfrac{1}{2}}=20\left(cm\right)\)
Áp dụng định lí Pytago vào ΔMNK vuông tại M, ta được:
\(NK^2=MK^2+MN^2\)
\(\Leftrightarrow MK^2=300\)
hay \(MK=10\sqrt{3}\left(cm\right)\)

Bài 2:
a: =>2x+3=3+2căn 2
=>2x=2 căn 2
=>x=căn 2
b: =>2 căn x-1+căn x-1=15
=>3 căn x-1=15
=>x-1=25
=>x=26
c: =>|x-4|=2x+7
TH1: x>=4
=>2x+7=x-4
=>x=-11(loại)
TH2: x<4
=>2x+7=4-x
=>3x=-3
=>x=-1(nhận)


Nãy ghi nhầm =="
a)Hđ gđ là nghiệm pt
`x^2=2x+2m+1`
`<=>x^2-2x-2m-1=0`
Thay `m=1` vào pt ta có:
`x^2-2x-2-1=0`
`<=>x^2-2x-3=0`
`a-b+c=0`
`=>x_1=-1,x_2=3`
`=>y_1=1,y_2=9`
`=>(-1,1),(3,9)`
Vậy tọa độ gđ (d) và (P) là `(-1,1)` và `(3,9)`
b)
Hđ gđ là nghiệm pt
`x^2=2x+2m+1`
`<=>x^2-2x-2m-1=0`
PT có 2 nghiệm pb
`<=>Delta'>0`
`<=>1+2m+1>0`
`<=>2m> -2`
`<=>m> 01`
Áp dụng hệ thức vi-ét:`x_1+x_2=2,x_1.x_2=-2m-1`
Theo `(P):y=x^2=>y_1=x_1^2,y_2=x_2^2`
`=>x_1^2+x_2^2=14`
`<=>(x_1+x_2)^2-2x_1.x_2=14`
`<=>4-2(-2m-1)=14`
`<=>4+2(2m+1)=14`
`<=>2(2m+1)=10`
`<=>2m+1=5`
`<=>2m=4`
`<=>m=2(tm)`
Vậy `m=2` thì ....

1: Ta có: \(\sqrt{3x-5}=2\)
\(\Leftrightarrow3x-5=4\)
hay x=3
2: Ta có: \(\sqrt{25\left(x-1\right)}=20\)
\(\Leftrightarrow x-1=16\)
hay x=17

2.c
\(x+\sqrt{4x^2-4x+1}=5\)
\(\Leftrightarrow x+\sqrt{\left(2x-1\right)^2}=5\)
\(\Leftrightarrow x+\left|2x-1\right|=5\)
\(\Leftrightarrow\left[{}\begin{matrix}x+2x-1=5;x\ge\dfrac{1}{2}\\x+1-2x=5;x< \dfrac{1}{2}\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}3x=6\\-x=4\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=2\left(tm\right)\\x=-4\left(tm\right)\end{matrix}\right.\)
Vậy \(S=\left\{2;-4\right\}\)










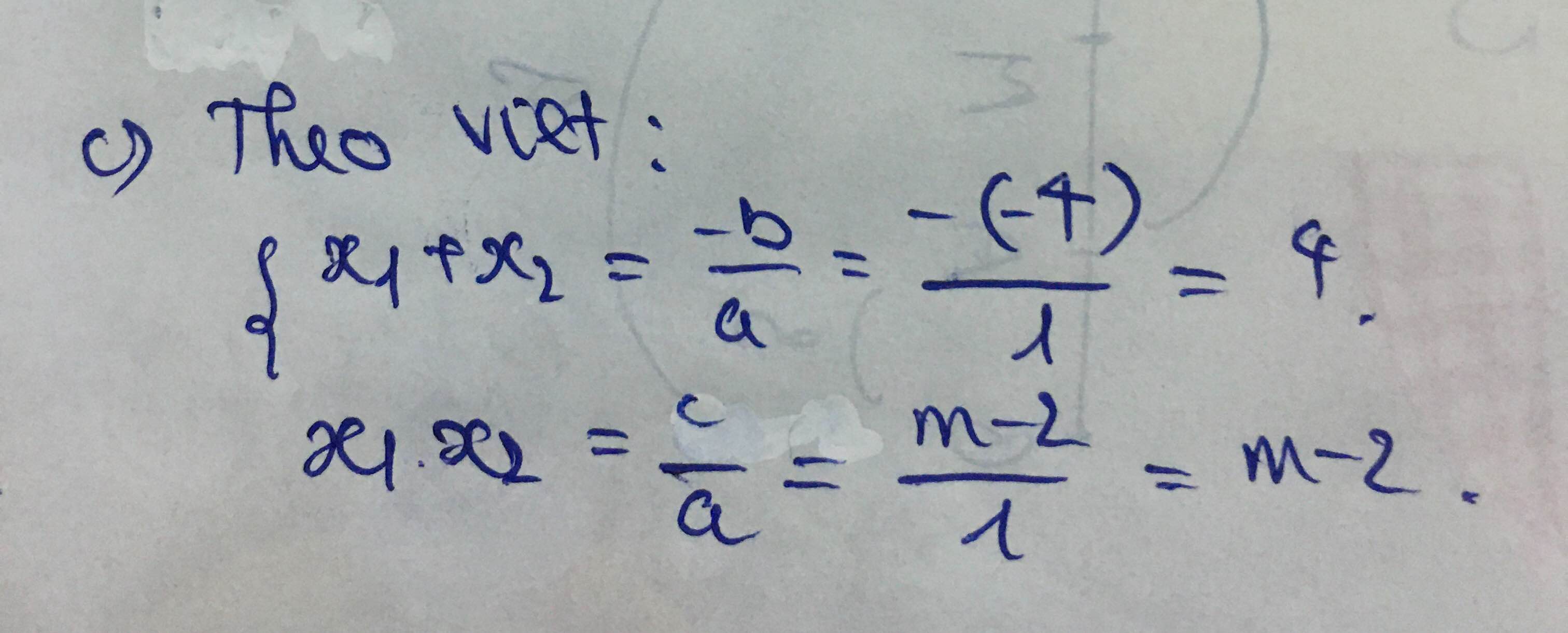
 ai giúp mình giải với mình cần câu c với câu d thôi cũng đc ạ! cảm ơn
ai giúp mình giải với mình cần câu c với câu d thôi cũng đc ạ! cảm ơn
Câu 1:
\(a,B=\dfrac{2\cdot3-1}{9-4}=\dfrac{5}{5}=1\\ b,A=\dfrac{x-2\sqrt{x}+x+2\sqrt{x}-2x+\sqrt{x}+5}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\dfrac{\sqrt{x}+5}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\\ b,\dfrac{A}{B}=\dfrac{\sqrt{x}+5}{x-4}\cdot\dfrac{x-4}{2\sqrt{x}-1}=\dfrac{\sqrt{x}+5}{2\sqrt{x}-1}< 0\\ \Leftrightarrow2\sqrt{x}-1< 0\left(\sqrt{x}+5>0\right)\\ \Leftrightarrow\sqrt{x}< \dfrac{1}{2}\Leftrightarrow0\le x< \dfrac{1}{4}\)
1 a) Với \(a\ge0\) và \(a\ne4\) ta có
\(A=\dfrac{\sqrt{x}}{\sqrt{x}+2}-\dfrac{\sqrt{x}}{2-\sqrt{x}}-\dfrac{2x-2\sqrt{x}-5}{x-4},B=\dfrac{2\sqrt{x}-1}{x-4}\)
a)
A=\(\dfrac{\sqrt{x}\left(\sqrt{x}-2\right)}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}-\dfrac{\sqrt{x}}{-\left(\sqrt{x}-2\right)}-\dfrac{2x-2\sqrt{x}-5}{x-4}\)
A=\(\dfrac{\sqrt{x}+\left(\sqrt{x}-2\right)}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}+\dfrac{\sqrt{x}\left(\sqrt{x}+2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}-\dfrac{2x-2\sqrt{x}-5}{x-4}\)
A=\(\dfrac{\sqrt{x}\left(\sqrt{x}-2\right)+\sqrt{x}\left(\sqrt{x}+2\right)}{x-4}-\dfrac{2x-2\sqrt{x}-5}{x-4}\)
A=\(\dfrac{x-2\sqrt{x}+x+2\sqrt{x}}{x-4}-\dfrac{2x-2\sqrt{x}-5}{x-4}\)=\(\dfrac{2x}{x-4}-\dfrac{2x-2\sqrt{x}-5}{x-4}\)=\(\dfrac{2x-2x+2\sqrt{x}+5}{x-4}\)
A=\(\dfrac{2\sqrt{x}+5}{x-4}\)
Gía Trị Của B Khi x=9 Là ;
B=\(\dfrac{2\sqrt{9}-1}{9-4}=\dfrac{6-1}{5}=\dfrac{5}{5}=1\)
:)) câu c ko chắc :V
để \(\dfrac{A}{B}< 0\)(=)\(\dfrac{2\sqrt{x}+5}{x-4}:\dfrac{2\sqrt{x}-1}{x-4}\left(=\right)\dfrac{2\sqrt{x}+4}{x-4}\cdot\dfrac{x-4}{2\sqrt[]{x}-1}\left(=\right)\dfrac{\left(2\sqrt{x}+4\right)\left(x-4\right)}{\left(x-4\right)\left(2\sqrt{x}-1\right)}\)(=)\(\dfrac{2\sqrt{x}+4}{2\sqrt{x}-1}\)<0(=) bí :V