K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
11 tháng 1 2023
a: Xét tứ giác DIHK có
góc DIH=góc DKH=góc KDI=90 độ
nên DIHK là hình chữ nhật
b: Xét tứ giác IHAK có
IH//AK
IH=AK
Do đó: IHAK là hình bình hành
=>B là trung điểm chung của IA và HK
Xét ΔIKA có IC/IK=IB/IA
nên BC//KA
Xét ΔIDA có IB/IA=IM/ID
nên BM//DA
=>B,C,M thẳng hàng
B
2
HQ
Hà Quang Minh
Giáo viên
20 tháng 7 2023
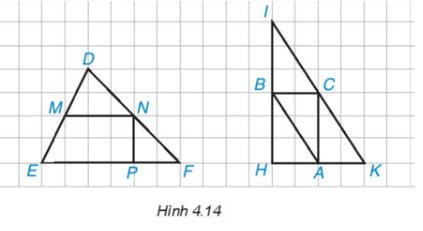
Đường trung bình trong tam giác DEF là: cạnh MN.
Đường trung bình trong tam giác HIK là: cạnh BC, CA, AB.
HQ
Hà Quang Minh
Giáo viên
13 tháng 1 2024
Quan sát Hình 4.14, ta thấy:
* Xét ∆DEF có M là trung điểm của cạnh DE; N là trung điểm của cạnh DF nên MN là đường trung bình của ∆DEF.
* Xét ∆IHK có:
• B là trung điểm của cạnh IH; C là trung điểm của cạnh IK nên BC là đường trung bình của ∆DEF.
• B là trung điểm của cạnh IH; A là trung điểm của cạnh HK nên AB là đường trung bình của ∆DEF.
• A là trung điểm của cạnh HK; C là trung điểm của cạnh IK nên AC là đường trung bình của ∆DEF.
Vậy đường trung bình của ∆DEF là MN; các đường trung bình của ∆IHK là AB, BC, AC.
P
Phong
CTVHS
2 tháng 2 2024
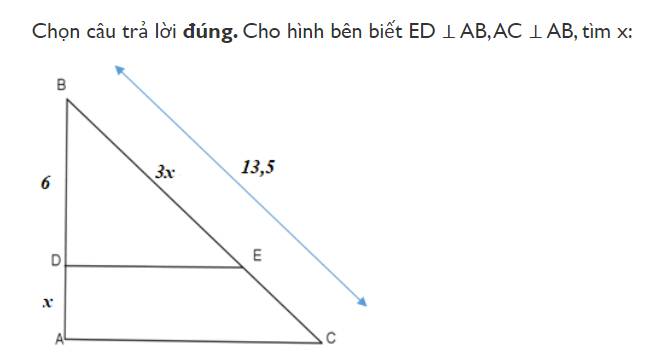
Ta có: DE//AC (cùng vuông góc với AB)
Áp dụng định lý Ta-lét ta có:
\(\dfrac{BD}{AD}=\dfrac{BE}{CE}\Rightarrow\dfrac{BD}{AD}=\dfrac{BE}{BC-BE}\Rightarrow\dfrac{6}{x}=\dfrac{3x}{13,5-3x}\)
\(\Leftrightarrow6\left(13,5-3x\right)=x\cdot3x\)
\(\Leftrightarrow81-18x=3x^2\)
\(\Leftrightarrow27-6x=x^2\)
\(\Leftrightarrow x^2+6x-27=0\)
\(\Leftrightarrow x^2-3x+9x-27=0\)
\(\Leftrightarrow x\left(x-3\right)+9\left(x-3\right)=0\)
\(\Leftrightarrow\left(x-3\right)\left(x+9\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\left(tm\right)\\x=-9\left(ktm\right)\end{matrix}\right.\)
Vậy: `x=3`
P
Phong
CTVHS
19 tháng 10 2023
a) Xét tứ giác ABCD ta có:
\(\widehat{A}+\widehat{B}+\widehat{C}+\widehat{D}=360^o\)
\(\Rightarrow\widehat{D}=360^o-102^o-102^o-102^o\)
\(\Rightarrow\widehat{D}=54^o\)
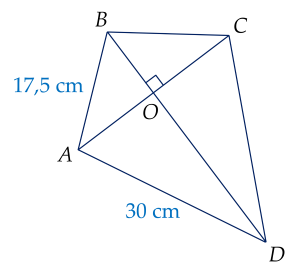
b) Xét tam giác vuông AOD ta có:
\(AD^2=OD^2+OA^2\)
\(\Rightarrow OA=\sqrt{AD^2-OD^2}\)
\(\Rightarrow OA=\sqrt{30^2-26,7^2}\approx13,7\left(cm\right)\)
Xét tam giác vuông AOB ta có:
\(AB^2=OA^2+OB^2\)
\(\Rightarrow OB=\sqrt{AB^2-OA^2}\)
\(\Rightarrow OB=\sqrt{17,5^2-13,7^2}\approx10,9\left(cm\right)\)
Độ dài đường chéo BD là:
\(BD=OB+OD=26,7+10,9\approx37,6\left(cm\right)\)
NM
Nguyễn Minh Châu
VIP
22 tháng 8
a) Số đo góc \(D\) ở đuôi chiếc diều là: \(\hat{D} = 36 0^{\circ} - \left(\right. \hat{A} + \hat{B} + \hat{C} \left.\right) = 36 0^{\circ} - \left(\right. 10 2^{\circ} + 10 2^{\circ} + 10 2^{\circ} \&\text{nbsp}; \left.\right) = 5 4^{\circ} .\)
b) Xét \(\Delta O A D\) vuông tại \(O\), theo định lí Pythagore ta có:
\(O A^{2} = A D^{2} - O D^{2} = 30^{2} - 26 , 7^{2} = 187 , 11\)
Xét \(\Delta O A B\) vuông tại \(O ,\) theo định lí Pythagore ta có:
\(O B^{2} = A B^{2} - O A^{2} = 17 , 5^{2} - 187 , 11 = 119 , 14\)
Do đó \(O B = \sqrt{119 , 14} \approx 10 , 9\) (cm).
Suy ra \(B D = O B + O D = 10 , 9 + 26 , 7 = 37 , 6\) (cm).
HQ
Hà Quang Minh
Giáo viên
11 tháng 1 2024
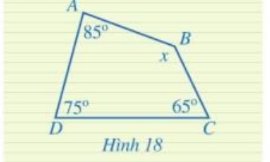
Xét tứ giác ABCD có:
\(\begin{array}{l} \widehat A + \widehat B + \widehat C + \widehat D = {360^0}\\{85^0} + x + {65^0} + {75^0} = {360^0}\\x = {360^0} - {85^0} - {65^0} - {75^0} = {135^0}\end{array}\)
NT
3
HQ
Hà Quang Minh
Giáo viên
13 tháng 1 2024
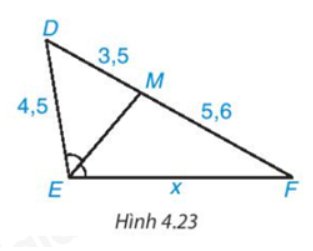
Trong Hình 4.23 có \(\widehat {DME} = \widehat {MEF}\) nên EM là tia phân giác của \(\widehat {{\rm{DEF}}}\).
Áp dụng tính chất đường phân giác của tam giác, ta có:
\(\dfrac{{E{\rm{D}}}}{{EF}} = \dfrac{{M{\rm{D}}}}{{MF}}\) hay \(\dfrac{{4,5}}{x} = \dfrac{{3,5}}{{5,6}}\)
Suy ra: \(x = \dfrac{{5,6.4,5}}{{3,5}} = 7,2\)(đvđd)
Vậy x = 7,2 (đvđd).
Trả lời lẹ giúp em đi ạ
a: MNPQ là hình bình hành
=>MQ//NP
=>MQ//IP
Xét tứ giác MIPQ có IP//MQ
nên MIPQ là hình thang
b: ΔMNP vuông cân tại N
=>MN=NP và \(\widehat{MNP}=90^0\)
Hình bình hành MNPQ có \(\widehat{MNP}=90^0\)
nên MNPQ là hình chữ nhật
=>\(\widehat{Q}=\widehat{P}=90^0\)
Xét ΔMNI vuông tại N có \(sinNMI=\dfrac{NI}{MN}=\dfrac{2}{3}\)
nên \(\widehat{NMI}\simeq42^0\)
\(\widehat{NMI}+\widehat{QMI}=\widehat{NMQ}=90^0\)
=>\(\widehat{QMI}+42^0=90^0\)
=>\(\widehat{QMI}=48^0\)
IP//MQ
=>\(\widehat{QMI}+\widehat{MIP}=180^0\)(hai góc trong cùng phía)
=>\(\widehat{MIP}+48^0=180^0\)
=>\(\widehat{MIP}=132^0\)