
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


65 - 5 . ( x + 2 ) = 15
5 . ( x + 2 ) = 65 - 15
5 . ( x + 2 ) = 50
( x + 2 ) = 50 : 5
x + 2 = 10
x = 8
Vậy x = 8
\(65-5.\left(x+2\right)=15\)
\(5\left(x+2\right)=50\)
\(x+2=10\)
\(x=8\)


3:
a: =8/24+9/24-14/24=3/24=1/8
b: =-12/56+35/56-28/56=-5/56
c: =9/36-24/36-22/36=-37/36
d: \(=\dfrac{6}{24}+\dfrac{10}{24}-\dfrac{21}{24}-\dfrac{1}{13}=\dfrac{-5}{24}-\dfrac{1}{13}=\dfrac{-89}{24\cdot13}\)

1/
$C=5+(5^2+5^3)+(5^4+5^5)+.....+(5^{2022}+5^{2023})$
$=5+5^2(1+5)+5^4(1+5)+....+5^{2022}(1+5)$
$=5+(1+5)(5^2+5^4+....+5^{2022})$
$=5+6(5^2+5^4+....+5^{2022})$
$\Rightarrow C$ chia $6$ dư $5$
$\Rightarrow C\not\vdots 6$
2/
$D=(1+2+2^2)+(2^3+2^4+2^5)+....+(2^{2019}+2^{2020}+2^{2021})$
$=(1+2+2^2)+2^3(1+2+2^2)+....+2^{2019}(1+2+2^2)$
$=(1+2+2^2)(1+2^3+...+2^{2019})$
$=7(1+2^3+...+2^{2019})\vdots 7$
Ta có đpcm.

Bài làm
Bạn hamlon bên dưới không làm được vậy để mình làm cho bạn .
\(\frac{3}{5}.\frac{3}{7}+\frac{3}{5}.\frac{2}{9}-\frac{3}{5}.\frac{3}{11}\)
\(=\frac{3}{5}\left(\frac{3}{7}+\frac{2}{9}-\frac{3}{11}\right)\)
\(=\frac{3}{5}.\left(\frac{297}{693}+\frac{154}{693}-\frac{189}{693}\right)\)
\(=\frac{3}{5}.\frac{262}{693}\)
\(=\frac{262}{1155}\)

a: Ư(8)={1;2;4;8}
Ư(12)={1;2;3;4;6;12}
UC(8;12)={1;2;4}
b: B(16)={0;16;32;...}
B(24)={0;24;48;...}
BC(16,24)={0;48;96;...}


Bài 1:
a. $-27+(-154)-(-27)+54$
$=(-27)-(-27)+(-154)+54=0-154+54=0-(154-54)=0-100=-100$
b.
$-35.127+(-35).(-27)+700$
$=(-35)(127-27)+700=-35.100+700=-3500+700=-2800$
c.
$-3^4-2[(-2023)^0+(-5)^2]=-81-2(1+25)=-81-2.26=-81-52$
$=-(81+52)=-133$
Bài 2:
a. $-34-2(7-x)=-10$
$2(7-x)=-34-(-10)=-24$
$7-x=-24:2=-12$
$x=7-(-12)=19$
b.
$x=ƯC(36,54,90)$
$\Rightarrow ƯCLN(36,54,90)\vdots x$
$\Rightarrow 18\vdots x$
$\Rightarrow x\in \left\{\pm 1; \pm 2; \pm 3; \pm 6; \pm 9; \pm 18\right\}$
Mà $x>5$ nên $x\in \left\{6; 9; 18\right\}$

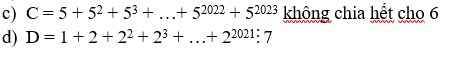
 giúp em với ạ em đang cần gấp cảm ơn ạ
giúp em với ạ em đang cần gấp cảm ơn ạ 
 Giúp em với ạ, em cần gấp trước 7h15 ạ. Em cảm ơn nhiều.
Giúp em với ạ, em cần gấp trước 7h15 ạ. Em cảm ơn nhiều.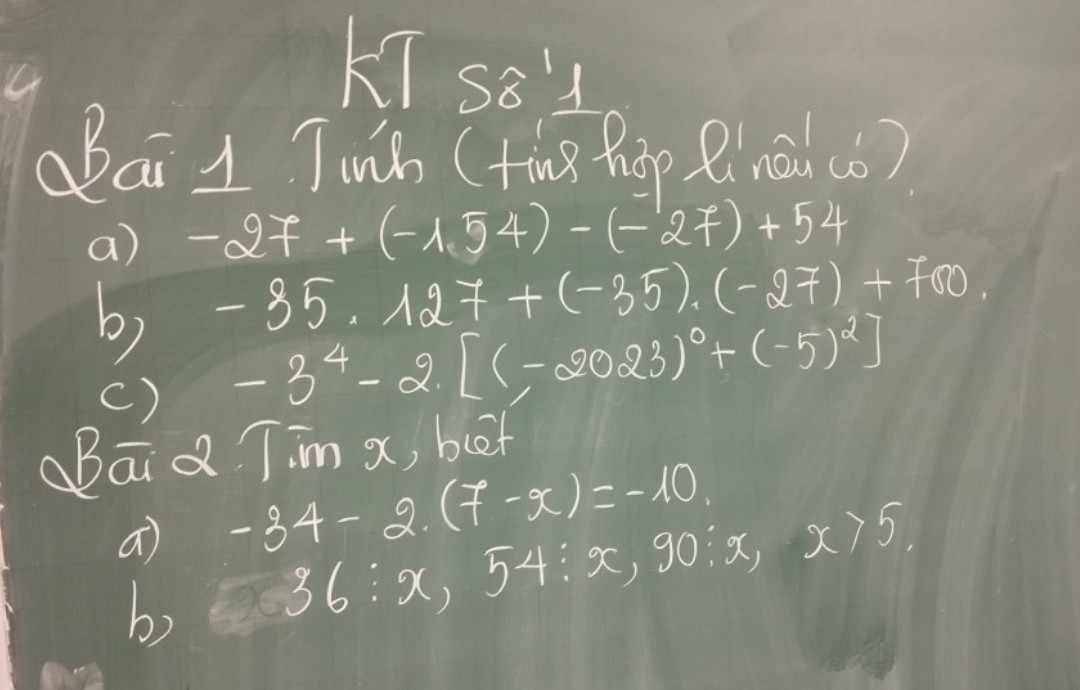
\(a^3b-ab^3=ab\left(a^2-b^2\right)=ab\left(a^2-ab+ab-b^2\right)=ab\left(a-b\right)\left(a+b\right)\)
Với a hoặc b chẵn \(\Leftrightarrow ab\left(a-b\right)\left(a+b\right)⋮2\)
Với a và b lẻ \(\Leftrightarrow\left(a-b\right)⋮2\Leftrightarrow ab\left(a-b\right)\left(a+b\right)⋮2\)
Vậy \(ab\left(a-b\right)\left(a+b\right)⋮2,\forall a,b\left(1\right)\)
Với a hoặc b chia hết cho 3 thì \(ab\left(a-b\right)\left(a+b\right)⋮3\)
Với \(a=3k+1;b=3q+1\Leftrightarrow\left(a-b\right)=3\left(k-q\right)⋮3\)
\(\Leftrightarrow ab\left(a-b\right)\left(a+b\right)⋮3\)
Với \(a=3k+1;b=3q+2\Leftrightarrow\left(a+b\right)=\left(3k+1+3q+2\right)=3\left(k+q+1\right)⋮3\)
\(\Leftrightarrow ab\left(a-b\right)\left(a+b\right)⋮3\)
Mà a,b có vai trò tương đương nên \(ab\left(a-b\right)\left(a+b\right)⋮3,\forall a,b\left(2\right)\)
\(\left(1\right)\left(2\right)\Leftrightarrowđpcm\)
Ta có : a3b -ab3
=a3b -ab -ab3 +ab
=ab (a2 -1) -ab (b2 -1)
=ab (a-1)(a+1) -ab (b-1)(b+1)
Vì a (a-1)(a+1) là 3 số tự nhiên liên tiếp nên chia hết cho 6 .Tương tự b (b-1)(b+1) cũng chia hết cho 6
=> a3b -ab3 chia hết cho 6 (đpcm )