Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

I là trọng tâm của ΔABC
=>\(\left\{{}\begin{matrix}x_A+x_B+x_C=3\cdot x_I\\y_A+y_B+y_C=3\cdot y_I\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}3+\left(-1\right)+x_C=3\cdot1=3\\-1+2+y_C=3\cdot1=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_C=3-2=1\\y_C=3-1=2\end{matrix}\right.\)
Vậy: C(1;2)
Ta có: A(3;-1); B(-1;2); C(1;2); D(x;y)
=>\(\overrightarrow{AB}=\left(-4;3\right);\overrightarrow{DC}=\left(1-x;2-y\right)\)
ABCD là hình bình hành
=>\(\overrightarrow{AB}=\overrightarrow{DC}\)
=>\(\left\{{}\begin{matrix}1-x=-4\\2-y=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=5\\y=-1\end{matrix}\right.\)
Vậy: D(5;-1)
Tâm O của hình bình hành ABCD sẽ là trung điểm của AC
A(3;-1); C(1;2); O(x;y)
=>\(\left\{{}\begin{matrix}x=\dfrac{3+1}{2}=\dfrac{4}{2}=2\\y=\dfrac{-1+2}{2}=\dfrac{1}{2}\end{matrix}\right.\)
Áp dụng công thức trọng tâm:
\(\left\{{}\begin{matrix}x_A+x_B+x_C=3x_I\\y_A+y_B+y_C=3y_I\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x_C=3x_I-\left(x_A+x_B\right)=1\\y_C=3y_I-\left(y_A+y_B\right)=2\end{matrix}\right.\)
\(\Rightarrow C\left(1;2\right)\)
Đặt tọa độ D là \(D\left(x;y\right)\Rightarrow\left\{{}\begin{matrix}\overrightarrow{AB}=\left(-4;3\right)\\\overrightarrow{DC}=\left(1-x;2-y\right)\end{matrix}\right.\)
ABCD là hình bình hành \(\Leftrightarrow\overrightarrow{AB}=\overrightarrow{DC}\)
\(\Rightarrow\left\{{}\begin{matrix}1-x=-4\\2-y=3\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x=5\\y=-1\end{matrix}\right.\) \(\Rightarrow D\left(5;-1\right)\)
Tâm O hình bình hành là trung điểm đường chéo AC nên áp dụng công thức trung điểm:
\(\left\{{}\begin{matrix}x_O=\dfrac{x_A+x_C}{2}=2\\y_O=\dfrac{y_A+y_C}{2}=\dfrac{1}{2}\end{matrix}\right.\) \(\Rightarrow O\left(2;\dfrac{1}{2}\right)\)

A(m-1;-1); B(2;2-2m); C(m+3;3)
\(\overrightarrow{AB}=\left(2-m+1;2-2m+1\right)\)
=>\(\overrightarrow{AB}=\left(3-m;3-2m\right)\)
\(\overrightarrow{AC}=\left(m+3-m+1;3+1\right)\)
=>\(\overrightarrow{AC}=\left(4;4\right)\)
Để A,B,C thẳng hàng thì \(\dfrac{3-m}{4}=\dfrac{3-2m}{4}\)
=>3-m=3-2m
=>m=0
\(\left\{{}\begin{matrix}\overrightarrow{AB}=\left(3-m;3-2m\right)\\\overrightarrow{AC}=\left(4;4\right)\end{matrix}\right.\)
3 điểm A;B;C thẳng hàng khi và chỉ khi \(\overrightarrow{AB}=k\overrightarrow{AC}\) với \(k\ne0\)
Hay \(\dfrac{3-m}{4}=\dfrac{3-2m}{4}\Rightarrow m=0\)

a/ Để chứng minh A,B,C là 3 đỉnh của tam giác cần chứng minh
\(\overrightarrow{AB}+\overrightarrow{BC}=\overrightarrow{AC}\)
Thật vậy: \(\left(x_B-x_A;y_B-y_A\right)+\left(x_C-x_B;y_C-y_B\right)=\left(x_C-x_A;y_C-y_A\right)\)
\(\Leftrightarrow\left(-3-6;6-3\right)+\left(1+3;-2-6\right)=\left(1-6;-2-3\right)\)
\(\Leftrightarrow\left(-5;-5\right)=\left(-5;-5\right)\)
Vậy ...
b/ Để A,B,D thẳng hàng<=> \(\overrightarrow{AB}=x\overrightarrow{AD}\)
Vì D nằm trên trục hoành nên yD= 0
\(\Leftrightarrow\left(x_B-x_A;y_B-y_A\right)=x\left(x_D-x_A;y_D-y_A\right)\)
\(\Leftrightarrow\left(-9;3\right)=x\left(x_D-6;y_D-3\right)\)
\(\left\{{}\begin{matrix}x\left(x_D-6\right)=-9\\-3x=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-1\\x_D=9+6=15\end{matrix}\right.\)
\(\Rightarrow D\left(15;0\right)\)
c/ \(E\in BC\Rightarrow\overrightarrow{BE}=2\overrightarrow{EC}\)
\(\Leftrightarrow\left(x_E-x_B;y_E-y_B\right)=2\left(x_C-x_E;y_C-y_E\right)\)
\(\Leftrightarrow\left(x_E+3;y_E-6\right)=2\left(1-x_E;-2-y_E\right)\)
\(\Leftrightarrow\left\{{}\begin{matrix}3+x_E=2-2x_E\\y_E-6=-4-2y_E\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_E=-\frac{1}{3}\\y_E=\frac{2}{3}\end{matrix}\right.\Rightarrow E\left(-\frac{1}{3};\frac{2}{3}\right)\)
d/ Gọi pt đt DE có dạng: \(\left(d_1\right)y=ax+b\)
Vì \(D,E\in\left(d_1\right)\Rightarrow\left\{{}\begin{matrix}15a+b=0\\-\frac{1}{3}a+b=\frac{2}{3}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=-\frac{1}{23}\\b=\frac{15}{23}\end{matrix}\right.\)
\(\Rightarrow\left(d_1\right)y=-\frac{1}{23}x+\frac{15}{23}\)
Gọi pt đt AC có dạng: \(\left(d_2\right)y=ax+b\)
Vì \(A,C\in\left(d_2\right)\Rightarrow\left\{{}\begin{matrix}6a+b=3\\a+b=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=1\\b=-3\end{matrix}\right.\)
\(\Rightarrow\left(d_2\right)y=x-3\)
Bạn tự xét PTHĐGĐ của (d1) và (d2)

a/ Gọi \(D\left(a;0\right)\) \(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{AB}=\left(-9;3\right)\\\overrightarrow{AD}=\left(a-6;-3\right)\end{matrix}\right.\)
Do A; B; D thẳng hàng \(\Leftrightarrow\frac{a-6}{-9}=\frac{-3}{3}\Rightarrow a=15\) \(\Rightarrow D\left(15;0\right)\)
b/ \(\overrightarrow{AB}=\left(-1;5\right);\) \(\overrightarrow{AD}=\left(-2;10\right)\)
\(\Rightarrow\overrightarrow{AD}=2\overrightarrow{AB}\Rightarrow A,B,D\) thẳng hàng
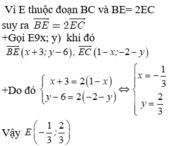

E trên trục hoành nên E(x;0)
A(6;3); B(-3;6); E(x;0)
\(\overrightarrow{AB}=\left(-9;3\right);\overrightarrow{AE}=\left(x-6;-3\right)\)
Để A,B,E thẳng hàng thì \(\dfrac{x-6}{-9}=\dfrac{-3}{3}=-1\)
=>x-6=9
=>x=15
Vậy: E(15;0)
Do E thuộc trục hoành nên tọa độ có dạng \(E\left(x;0\right)\)
\(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{AB}=\left(-9;3\right)\\\overrightarrow{AE}=\left(x-6;-3\right)\end{matrix}\right.\)
3 điểm A, B, E thẳng hàng khi:
\(\dfrac{x-6}{-9}=\dfrac{-3}{3}\Rightarrow x-6=9\)
\(\Rightarrow x=15\Rightarrow E\left(15;0\right)\)