K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
11 tháng 11 2021
a: \(\Leftrightarrow\left\{{}\begin{matrix}3x+6y=4\\x+4y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3x+6y=4\\3x+12y=6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-6y=-2\\x+4y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=\dfrac{1}{3}\\x=2-4y=2-4\cdot\dfrac{1}{3}=2-\dfrac{4}{3}=\dfrac{2}{3}\end{matrix}\right.\)
11 tháng 11 2021
a: \(\left\{{}\begin{matrix}3x+6y=4\\x+4y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3x+6y=4\\3x+12y=6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-6y=-2\\x+4y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=\dfrac{1}{3}\\x=\dfrac{2}{3}\end{matrix}\right.\)
4 tháng 9 2023
e:
\(E=\left(\dfrac{\sqrt{15}-\sqrt{20}}{2-\sqrt{3}}+\dfrac{\sqrt{21}-\sqrt{7}}{1-\sqrt{3}}\right):\dfrac{1}{\sqrt{7}-\sqrt{5}}\)
\(=\left(-\dfrac{\sqrt{5}\left(2-\sqrt{3}\right)}{2-\sqrt{3}}-\dfrac{\sqrt{7}\left(1-\sqrt{3}\right)}{1-\sqrt{3}}\right)\cdot\dfrac{\sqrt{7}-\sqrt{5}}{1}\)
\(=-\left(\sqrt{7}+\sqrt{5}\right)\left(\sqrt{7}-\sqrt{5}\right)\)
=-2
f: \(F=\sqrt{3}+1+2-\sqrt{3}=3\)
23 tháng 7 2021
Đặt \(\sqrt{x^2-x+1}=a>0;\sqrt{x^2+x+1}=b>0\).
\(PT\Leftrightarrow2a^2-b^2=-\dfrac{\sqrt{3}}{3}ab\)
\(\Leftrightarrow\left(a+\dfrac{\sqrt{3}}{2}b\right)\left(2a-\dfrac{2\sqrt{3}}{3}b\right)=0\)
\(\Leftrightarrow2a-\dfrac{2\sqrt{3}}{3}b=0\) (Do a, b > 0)
\(\Leftrightarrow2\sqrt{x^2-x+1}=\dfrac{2\sqrt{3}}{3}\sqrt{x^2+x+1}\)
\(\Leftrightarrow x^2-x+1=\dfrac{1}{3}\left(x^2+x+1\right)\Leftrightarrow2x^2-4x+2=0\Leftrightarrow x=1\).
Vậy x = 1
12 tháng 11 2021
a: \(\left\{{}\begin{matrix}3x+6y=4\\x+4y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3x+6y=4\\3x+12y=6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}y=\dfrac{1}{3}\\x=2-\dfrac{4}{3}=\dfrac{2}{3}\end{matrix}\right.\)
NT
3
NV
Nguyễn Việt Lâm
Giáo viên
11 tháng 9 2021
Qua A kẻ đường thẳng vuông góc AI cắt CD kéo dài tại E
Ta có \(\widehat{EAD}=\widehat{MAB}\) (cùng phụ \(\widehat{DAM}\))
\(\Rightarrow\Delta_vADE\sim\Delta_vABM\Rightarrow\dfrac{AE}{AM}=\dfrac{AD}{AB}=\dfrac{3}{4}\)
\(\Rightarrow\dfrac{1}{AE}=\dfrac{4}{3AM}\)
Áp dụng hệ thức lượng trong tam giác vuông AEI:
\(\dfrac{1}{AD^2}=\dfrac{1}{AE^2}+\dfrac{1}{AI^2}\Leftrightarrow\dfrac{1}{\left(\dfrac{3}{4}AB\right)^2}=\left(\dfrac{4}{3AM}\right)^2+\dfrac{1}{AI^2}\)
\(\Leftrightarrow\dfrac{1}{AB^2}=\dfrac{1}{AM^2}+\dfrac{9}{16AI^2}\)
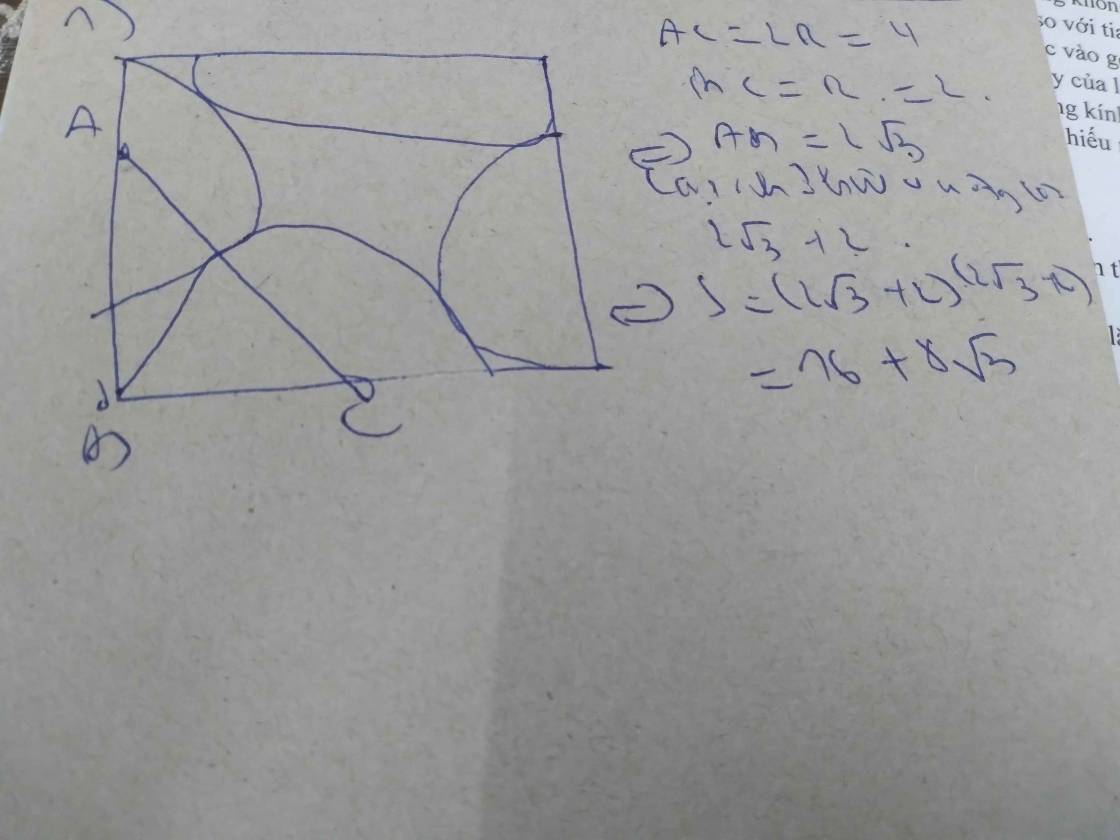
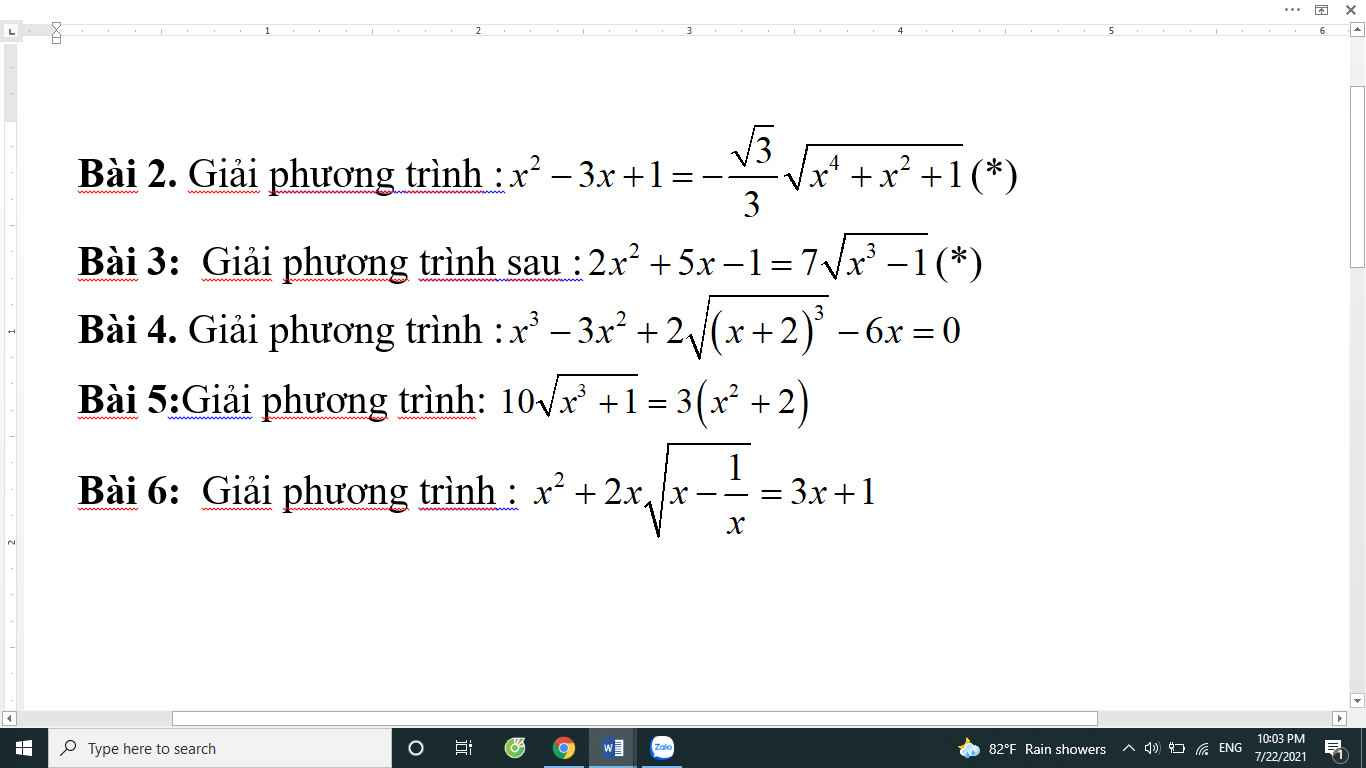
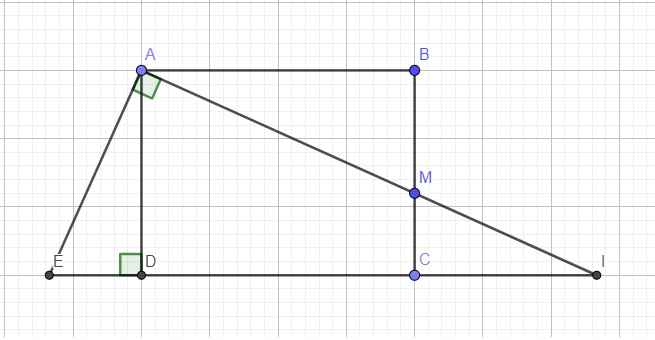
Mình nhìn hongg đc luôn á