
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


d: \(\Leftrightarrow x^2-x-1=x+2\)
\(\Leftrightarrow x^2-2x-3=0\)
=>(x-3)(x+1)=0
=>x=3 hoặc x=-1
e: \(\Leftrightarrow x^2-x-2+x-1=3x+4\)
\(\Leftrightarrow x^2-3-3x-4=0\)
\(\Leftrightarrow x^2-3x-7=0\)
\(\text{Δ}=\left(-3\right)^2-4\cdot1\cdot\left(-7\right)=37\)
Vì Δ>0 nên pt có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x_1=\dfrac{3-\sqrt{37}}{2}\\x_2=\dfrac{3+\sqrt{37}}{2}\end{matrix}\right.\)

3) Ta có: \(\text{Δ}=\left[-2\left(m-1\right)\right]^2-4\cdot1\cdot\left(m^2-6\right)\)
\(=\left(2m-2\right)^2-4\left(m^2-6\right)\)
\(=4m^2-8m+4-4m^2+24\)
\(=-8m+28\)
Để phương trình có hai nghiệm phân biệt x1;x2 thì Δ>0
\(\Leftrightarrow-8m+28>0\)
\(\Leftrightarrow-8m>-28\)
hay \(m< \dfrac{7}{2}\)
Áp dụng hệ thức Vi-et, ta được:
\(\left\{{}\begin{matrix}x_1+x_2=\dfrac{2\left(m-1\right)}{1}=2m-2\\x_1x_2=m^2-6\end{matrix}\right.\)
Ta có: \(x_1^2+x_2^2=16\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=16\)
\(\Leftrightarrow\left(2m-2\right)^2-2\left(m^2-6\right)-16=0\)
\(\Leftrightarrow4m^2-8m+4-2m^2+12-16=0\)
\(\Leftrightarrow2m^2-8m=0\)
\(\Leftrightarrow2m\left(m-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m=0\left(nhận\right)\\m=4\left(loại\right)\end{matrix}\right.\)


\(P=\dfrac{2\sqrt{x}}{\sqrt{x}+3}\left(x\ge0\right)\)
để P>\(\dfrac{1}{4}< =>\dfrac{2\sqrt{x}}{\sqrt{x}+3}>\dfrac{1}{4} < =>\dfrac{2\sqrt{x}}{\sqrt{x}+3}-\dfrac{1}{4}>0\)
<=>\(\dfrac{4.2\sqrt{x}}{4\left(\sqrt{x}+3\right)}-\dfrac{\sqrt{x}+3}{4\left(\sqrt{x}+3\right)}>0\)
<=>\(\dfrac{8\sqrt{x}-\sqrt{x}-3}{4\left(\sqrt{x}+3\right)}>0< =>\dfrac{7\sqrt{x}-3}{4\left(\sqrt{x}+3\right)}>0\)
ta có \(\sqrt{x}\ge0\left(\forall x\right)=>\sqrt{x}+3\ge3=>4\left(\sqrt{x}+3\right)>12\)
hay \(4\left(\sqrt{x}+3\right)>0\)
vậy để \(\dfrac{7\sqrt{x}-3}{4\left(\sqrt{x}+3\right)}>0< =>7\sqrt{x}-3>0< =>7\sqrt{x}>3< =>\sqrt{x}>\dfrac{3}{7}\)
<=>\(x>\dfrac{9}{49}\)
vậy x>9/49 thì pP>1/4



Với x >= 0 ; x khác 9
\(B=\dfrac{x-3\sqrt{x}-x-6\sqrt{x}-9+x+11\sqrt{x}+6}{x-9}\)
\(=\dfrac{x+2\sqrt{x}-3}{x-9}=\dfrac{\left(\sqrt{x}+3\right)\left(\sqrt{x}-1\right)}{x-9}=\dfrac{\sqrt{x}-1}{\sqrt{x}-3}\)
\(\Rightarrow P=A:B=\dfrac{\sqrt{x}-1}{\sqrt{x}+3}:\dfrac{\sqrt{x}-1}{\sqrt{x}-3}=\dfrac{\sqrt{x}-3}{\sqrt{x}+3}\)





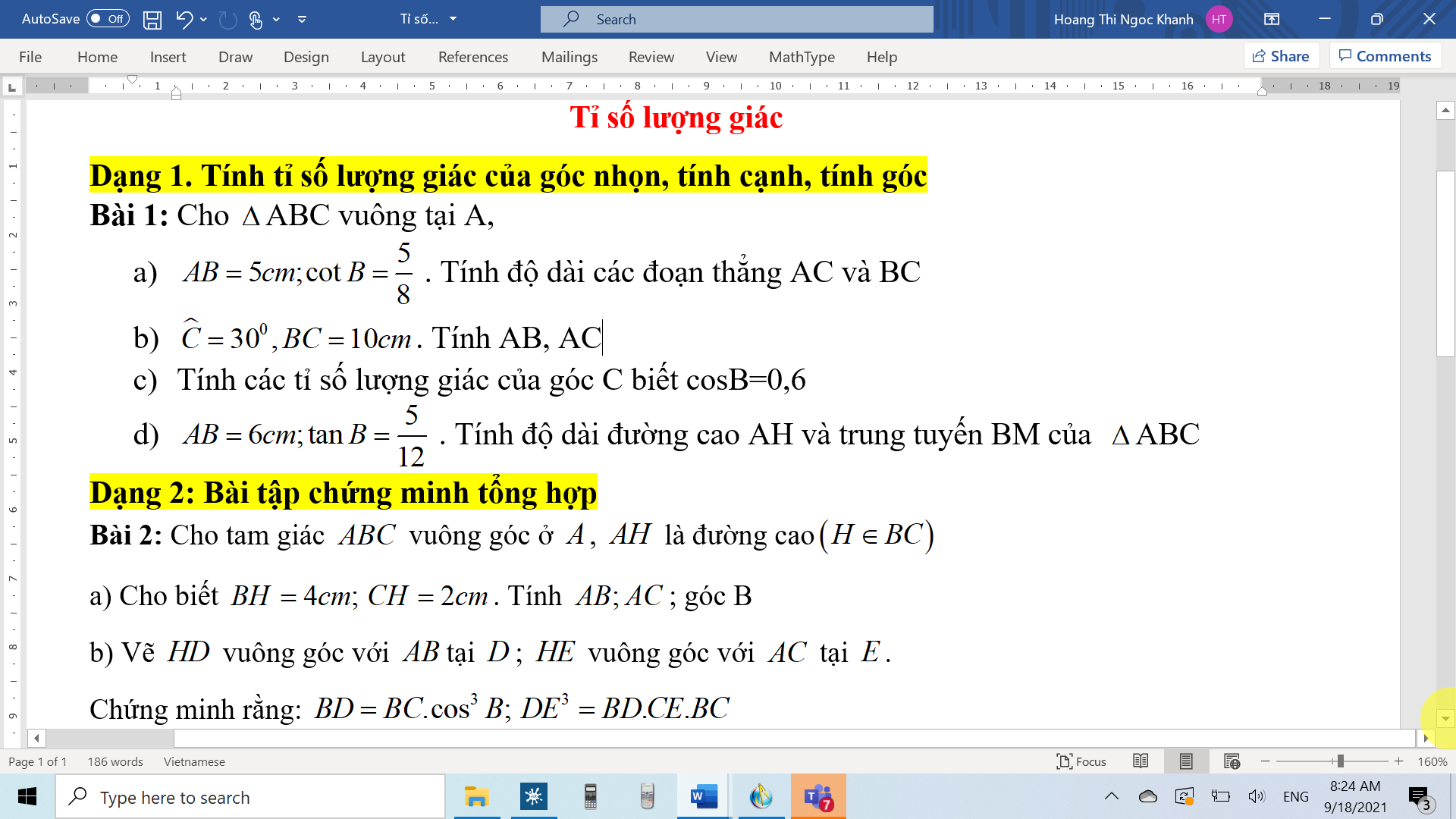
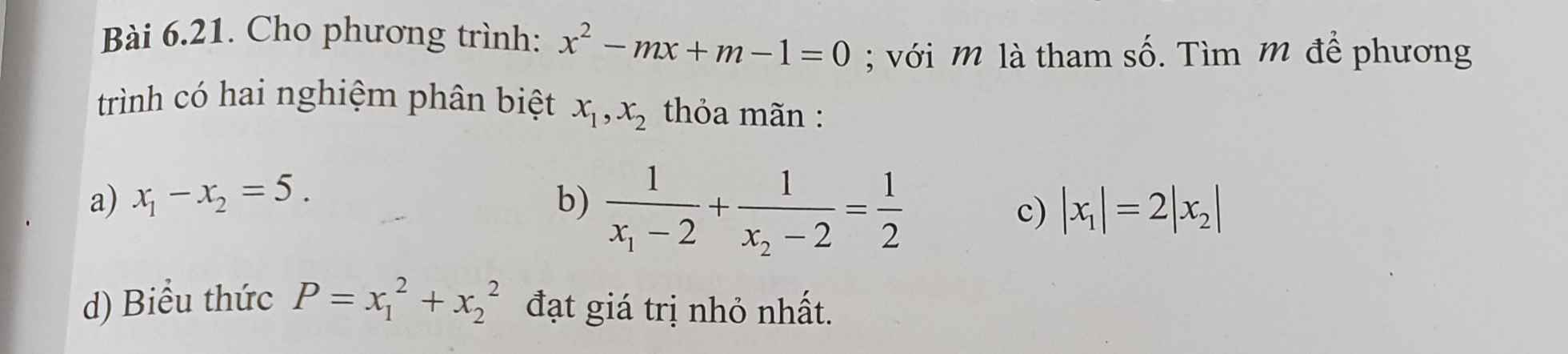




- Chụp thiếu rồi bạn ơi...
Bài 2 ý 1 ạ