NT
0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PT
1
CM
6 tháng 4 2017
(x – 1)(x2 + 3x – 2) – (x3 – 1) = 0
⇔ (x – 1)(x2 + 3x - 2) - (x - 1)(x2 + x + 1) = 0
⇔ (x – 1)[(x2 + 3x - 2) - (x2 + x + 1)] = 0
⇔ (x – 1). (x2 + 3x - 2 - x2 - x - 1) = 0
⇔ (x – 1)(2x - 3) = 0
⇔ x - 1 = 0 hoặc 2x - 3 = 0
+) Nếu x - 1 = 0 ⇔x = 1
+) Nếu 2x - 3 = 0 ⇔x = 3/2
Vậy tập nghiệm của phương trình là S = {1;3/2}
PT
1
CM
30 tháng 1 2019
(x – 1)( x 2 + 5x – 2) – ( x 3 – 1) = 0
⇔ (x – 1)( x 2 + 5x – 2) – (x – 1)( x 2 + x + 1) = 0
⇔ (x – 1)[( x 2 + 5x – 2) – ( x 2 + x + 1)] = 0
⇔ (x – 1)( x 2 + 5x – 2 – x 2 – x – 1) = 0
⇔ (x – 1)(4x – 3) = 0 ⇔ x – 1 = 0 hoặc 4x – 3 = 0
x – 1 = 0 ⇔ x = 1
4x – 3 = 0 ⇔ x = 0,75
Vậy phương trình có nghiệm x = 1 hoặc x = 0,75
CM
19 tháng 1 2019
Ta thấy x = 1 không phải nghiệm của phương trình nên nhân 2 vế của phương trình với x - 1 ta có:
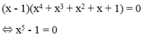
⇔ x = 1(KTM)
Vậy phương trình đã cho vô nghiệm.
CM
10 tháng 1 2017
(x3 + x2) + (x2 + x) = 0
⇔x2 (x + 1) + x(x + 1) = 0
⇔(x2 + x)(x + 1) = 0
⇔x(x + 1)(x + 1) = 0
⇔x = 0 hoặc x + 1 = 0
⇔x = 0 hoặc x = -1
Vậy tập nghiệm của phương trình là : S = {0; -1}
28 tháng 2 2021
a. (3x - 1)2 - (x + 3)2 = 0
\(\Leftrightarrow\left(3x-1+x+3\right)\left(3x-1-x-3\right)=0\)
\(\Leftrightarrow\left(4x+2\right)\left(2x-4\right)=0\)
\(\Leftrightarrow4x+2=0\) hoặc \(2x-4=0\)
1. \(4x+2=0\Leftrightarrow4x=-2\Leftrightarrow x=-\dfrac{1}{2}\)
2. \(2x-4=0\Leftrightarrow2x=4\Leftrightarrow x=2\)
S=\(\left\{-\dfrac{1}{2};2\right\}\)
28 tháng 2 2021
b. \(x^3=\dfrac{x}{49}\)
\(\Leftrightarrow49x^3=x\)
\(\Leftrightarrow49x^3-x=0\)
\(\Leftrightarrow x\left(49x^2-1\right)=0\)
\(\Leftrightarrow x\left(7x+1\right)\left(7x-1\right)=0\)
\(\Leftrightarrow x=0\) hoặc \(7x+1=0\) hoặc \(7x-1=0\)
1. x=0
2. \(7x+1=0\Leftrightarrow7x=-1\Leftrightarrow x=-\dfrac{1}{7}\)
3. \(7x-1=0\Leftrightarrow7x=1\Leftrightarrow x=\dfrac{1}{7}\)
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 3 2020
Bài 1
a/ \(x\left(x^2+1\right)+2\left(x^2+1\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(x^2+1\right)=0\Rightarrow x=-2\)
b/
\(\Leftrightarrow x^3-6x^2+9x+5x^2-30x+45=0\)
\(\Leftrightarrow x\left(x-3\right)^2+5\left(x-3\right)^2=0\)
\(\Leftrightarrow\left(x+5\right)\left(x-3\right)^2=0\)
\(\Rightarrow\left[{}\begin{matrix}x=-5\\x=3\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 3 2020
1.
c/ \(\Leftrightarrow x^3+2x^2+2x+x^2+2x+2=0\)
\(\Leftrightarrow x\left(x^2+2x+2\right)+x^2+2x+2=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^2+2x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x^2+2x+2=0\left(vn\right)\end{matrix}\right.\)
d/
\(\Leftrightarrow x^4+x^3-2x^2-x^3-x^2+2x+4x^2+4x-8=0\)
\(\Leftrightarrow x^2\left(x^2+x-2\right)-x\left(x^2+x-2\right)+4\left(x^2+x-2\right)=0\)
\(\Leftrightarrow\left(x^2-x+4\right)\left(x^2+x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-x+4=0\left(vn\right)\\x^2+x-2=0\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=1\\x=-2\end{matrix}\right.\)
LN
1
NV
Nguyễn Việt Lâm
Giáo viên
10 tháng 4 2022
Không dich được đề bài, đề là:
\(\dfrac{2x^2-1}{x^3+1}+\dfrac{1}{x+1}=2x\left(\dfrac{1-x^2-x}{x^2-x+1}\right)\)
Hay: \(...=2\left(1-x^2-\dfrac{x}{x^2-x+1}\right)\)