K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PT
1
NV
Nguyễn Việt Lâm
Giáo viên
30 tháng 7 2021
2.1
a.
\(\Leftrightarrow sinx-cosx=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow\sqrt{2}sin\left(x-\dfrac{\pi}{4}\right)=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow sin\left(x-\dfrac{\pi}{4}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{\pi}{4}=\dfrac{\pi}{6}+k2\pi\\x-\dfrac{\pi}{4}=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5\pi}{12}+k2\pi\\x=\dfrac{13\pi}{12}+k2\pi\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
30 tháng 7 2021
b.
\(cosx-\sqrt{3}sinx=1\)
\(\Leftrightarrow\dfrac{1}{2}cosx-\dfrac{\sqrt{3}}{2}sinx=\dfrac{1}{2}\)
\(\Leftrightarrow cos\left(x+\dfrac{\pi}{3}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{\pi}{3}=\dfrac{\pi}{3}+k2\pi\\x+\dfrac{\pi}{3}=-\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k2\pi\\x=-\dfrac{2\pi}{3}+k2\pi\end{matrix}\right.\)
HP
13 tháng 8 2021
1.
a, Phương trình có nghiệm khi:
\(\left(m+2\right)^2+m^2\ge4\)
\(\Leftrightarrow m^2+4m+4+m^2\ge4\)
\(\Leftrightarrow2m^2+4m\ge0\)
\(\Leftrightarrow\left[{}\begin{matrix}m\ge0\\m\le-2\end{matrix}\right.\)
b, Phương trình có nghiệm khi:
\(m^2+\left(m-1\right)^2\ge\left(2m+1\right)^2\)
\(\Leftrightarrow2m^2+6m\le0\)
\(\Leftrightarrow-3\le m\le0\)
HP
13 tháng 8 2021
2.
a, Phương trình vô nghiệm khi:
\(\left(2m-1\right)^2+\left(m-1\right)^2< \left(m-3\right)^2\)
\(\Leftrightarrow4m^2-4m+1+m^2-2m+1< m^2-6m+9\)
\(\Leftrightarrow4m^2-7< 0\)
\(\Leftrightarrow-\dfrac{\sqrt{7}}{2}< m< \dfrac{\sqrt{7}}{2}\)
b, \(2sinx+cosx=m\left(sinx-2cosx+3\right)\)
\(\Leftrightarrow\left(m-2\right)sinx-\left(2m+1\right)cosx=-3m\)
Phương trình vô nghiệm khi:
\(\left(m-2\right)^2+\left(2m+1\right)^2< 9m^2\)
\(\Leftrightarrow m^2-4m+4+4m^2+4m+1< 9m^2\)
\(\Leftrightarrow m^2-1>0\)
\(\Leftrightarrow\left[{}\begin{matrix}m>1\\m< -1\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
6 tháng 10 2020
a.
\(\Leftrightarrow sin2x+cos2x=3sinx+cosx+2\)
\(\Leftrightarrow2sinx.cosx-3sinx+2cos^2x-cosx-3=0=0\)
\(\Leftrightarrow sinx\left(2cosx-3\right)+\left(cosx+1\right)\left(2cosx-3\right)=0\)
\(\Leftrightarrow\left(sinx+cosx+1\right)\left(2cosx-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx+cosx=-1\\2cosx-3=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}sin\left(x+\frac{\pi}{4}\right)=-\frac{\sqrt{2}}{2}\\cosx=\frac{3}{2}\left(vn\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\frac{\pi}{4}=-\frac{\pi}{4}+k2\pi\\x+\frac{\pi}{4}=\frac{5\pi}{4}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow...\)
NV
Nguyễn Việt Lâm
Giáo viên
6 tháng 10 2020
b.
\(\Leftrightarrow1+sinx+cosx+2sinx.cosx+2cos^2x-1=0\)
\(\Leftrightarrow sinx\left(2cosx+1\right)+cosx\left(2cosx+1\right)=0\)
\(\Leftrightarrow\left(sinx+cosx\right)\left(2cosx+1\right)=0\)
\(\Leftrightarrow\sqrt{2}sin\left(x+\frac{\pi}{4}\right)\left(2cosx+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sin\left(x+\frac{\pi}{4}\right)=0\\cosx=-\frac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\frac{\pi}{4}+k\pi\\x=\frac{2\pi}{3}+k2\pi\\x=-\frac{2\pi}{3}+k2\pi\end{matrix}\right.\)
YP
20 tháng 8 2018
a.\(\dfrac{sin2x+cosx-\sqrt{3}\left(cos2x+sinx\right)}{2sin2x-\sqrt{3}}=1\left(1\right)\)
ĐKXĐ: sin2x≠\(\dfrac{\sqrt{3}}{2}\)
(1) ⇔ sin2x + cosx - \(\sqrt{3}\) ( cos2x + sinx) = 2sin2x - \(\sqrt{3}\)
⇔cosx - \(\sqrt{3}\) sinx = \(\sqrt{3}\) cos2x + sin2x +\(\sqrt{3}\)
⇔\(\dfrac{1}{2}cosx-\dfrac{\sqrt{3}}{2}sinx=\dfrac{\sqrt{3}}{2}cos2x+\dfrac{1}{2}sin2x+\dfrac{\sqrt{3}}{2}\)
⇔\(sin\left(\dfrac{\Pi}{6}-x\right)=sin\left(2x+\dfrac{\Pi}{3}\right)-sin\dfrac{\Pi}{3}\)
⇔\(sin\left(\dfrac{\Pi}{6}-x\right)=2cos\left(x+\dfrac{\Pi}{3}\right)sinx\)
⇔\(sin\left(\dfrac{\Pi}{6}-x\right)=2sin\left(\dfrac{\Pi}{6}-x\right)sinx\)
⇔\(sin\left(\dfrac{\Pi}{6}-x\right)\left(2sinx-1\right)=0\)
Đến đây tự giải tiếp nha nhớ đối chiếu đk.
YP
20 tháng 8 2018
b.\(\left(2cosx-1\right)cotx=\dfrac{3}{sinx}+\dfrac{2sinx}{cosx-1}\left(1\right)\)
ĐKXĐ: sinx≠0 và cosx≠1
(1)⇔\(\left(2cosx-1\right)\dfrac{cosx}{sinx}=\dfrac{3}{sinx}+\dfrac{2sinx}{cosx-1}\)
⇔cosx(2cosx-1)(cosx-1) = 3(cosx-1) + 2sin2x
⇔2cos3x - cos2x - 2cosx +1 = 0
⇔ (cosx-1)(cosx+1)(2cosx-1)=0
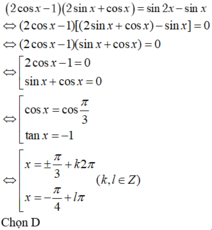
Lời giải:
PT $\Leftrightarrow (2\cos x-1)(2\sin x+\cos x)=2\sin x\cos x-\sin x$
$\Leftrightarrow (2\cos x-1)(2\sin x+\cos x)=\sin x(2\cos x-1)$
$\Leftrightarrow (2\cos x-1)(\sin x+\cos x)=0$
$\Rightarrow 2\cos x=1$ hoặc $\sin x=-\cos x=\cos (\pi -x)=\sin (x-\frac{\pi}{2})$
Đến đây thì đơn giản rồi.
Nkjuiopmli Sv5: Bạn chuyển vế sin x(2cos x-1) sang vế trái thì vế phải còn 0 đó.