
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


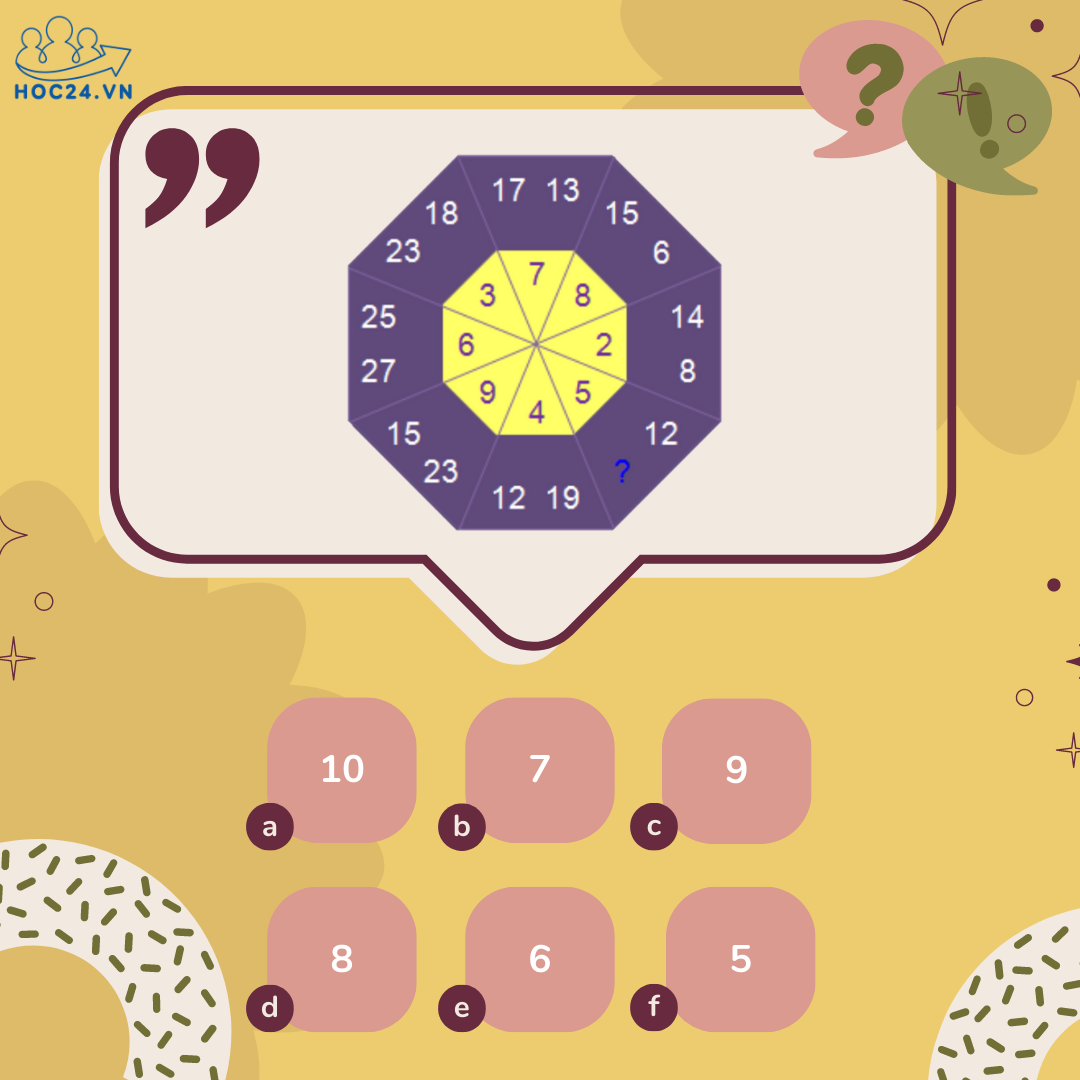
Quy luật: Hiệu của số lớn hơn trừ cho số nhỏ hơn trong mổi ô chính là kết quả của ô màu vàng đối diện
17-13=4
15-6=9
14-8=6
19-12=7
23-15=8
27-25=2
23-18=5
Suy ra: 12-x=3
=> x=12-3=9
Đáp án C
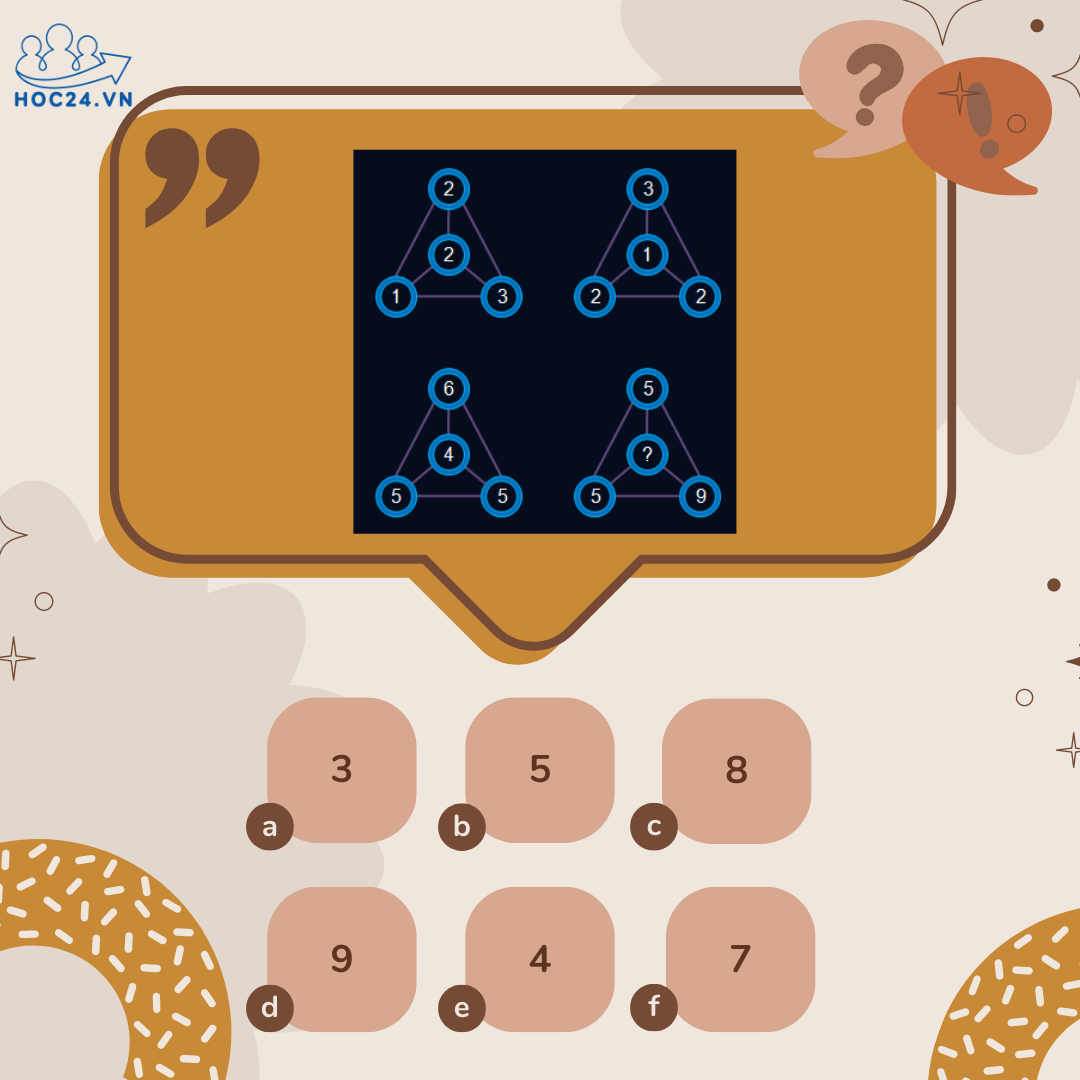
Giải thích: Mỗi số trong hình tam giác màu vàng bằng số lớn hơn của hình bình hành đối diện trừ đi số bé hơn ở hình bình hành đối diện.
=> ? - 12 = 3 hoặc 12 - ? = 3
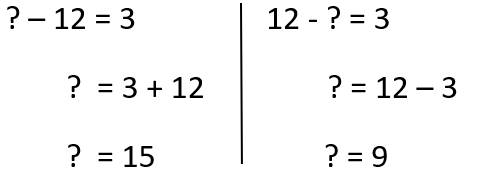
=> Đáp án là 15 hoặc 9
Đáp án: c
Bổ sung: Đáp án cũng có thể là 15

Ta có các quy luật sau:
\(\left(1+3\right)-2=2\)
\(\left(2+2\right)-3=1\)
\(\left(5+5\right)-6=4\)
Vậy dòng cuối là:
\(\left(5+9\right)-5=9\)
Số điền vào là 9
(Quy luật: lấy 2 số phía dưới cộng với nhau rồi trừ cho số phía trên sẽ ra được số ở giữa)

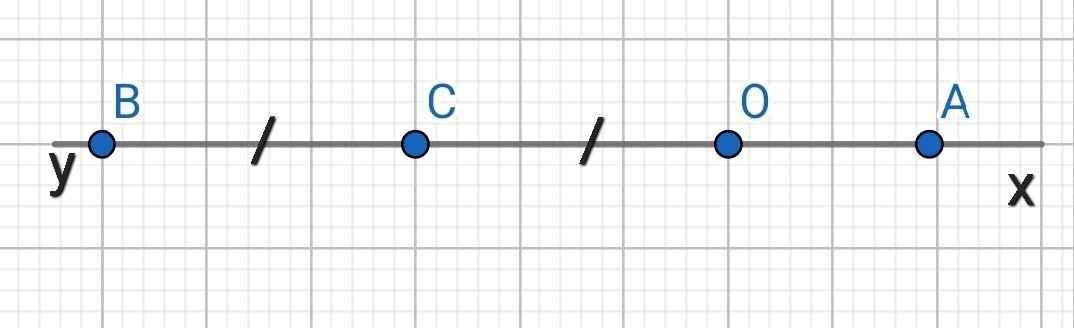 Do C là trung điểm của OB
Do C là trung điểm của OB
⇒ OC = OB : 2 = 6 : 2 = 3 (cm)
⇒ OC > OA
⇒ O không là trung điểm của AC

Bài 5:
a. Gọi $d=ƯCLN(n-2, n+1)$
$\Rightarrow n-2\vdots d; n+1\vdots d$
$\Rightarrow (n+1)-(n-2)\vdots d$
$\Rightarrow 3\vdots d\Rightarrow d\in \left\{1; 3\right\}$
Để ps tối giản thì $n-2\not\vdots 3$
$\Leftrightarrow n\neq 3k+2$ với $k$ là số tự nhiên bất kỳ.
b.
Gọi $d=ƯCLN(n+5, n-2)$
$\Rightarrow n+5\vdots d; n-2\vdots d$
$\Rightarrow (n+5)-(n-2)\vdots d$
$\Rightarrow 7\vdots d$
$\Rightarrow d\in \left\{1; 7\right\}$
Để ps tối giản thì $n-2\not\vdots 7$
$\Rightarrow n\neq 7k+2$ với $k$ là số tự nhiên bất kỳ.

Bài 4:
a; \(\dfrac{1}{4}\) - \(\dfrac{1}{5}\) = \(\dfrac{5}{20}\) - \(\dfrac{4}{20}\) = \(\dfrac{1}{20}\)
b; \(\dfrac{3}{5}\) - \(\dfrac{-1}{2}\) = \(\dfrac{6}{10}\) + \(\dfrac{5}{10}\) = \(\dfrac{11}{10}\)
c; \(\dfrac{3}{5}\) - \(\dfrac{-1}{3}\) = \(\dfrac{9}{15}\) + \(\dfrac{5}{15}\) = \(\dfrac{14}{15}\)
d; \(\dfrac{-5}{7}\) - \(\dfrac{1}{3}\)= \(\dfrac{-15}{21}\) - \(\dfrac{7}{21}\)= \(\dfrac{-22}{21}\)
Bài 5
a; 1 + \(\dfrac{3}{4}\) = \(\dfrac{4}{4}\) + \(\dfrac{3}{4}\) = \(\dfrac{7}{4}\) b; 1 - \(\dfrac{1}{2}\) = \(\dfrac{2}{2}\) - \(\dfrac{1}{2}\) = \(\dfrac{1}{2}\)
c; \(\dfrac{1}{5}\) - 2 = \(\dfrac{1}{5}\) - \(\dfrac{10}{5}\) = \(\dfrac{-9}{5}\) d; -5 - \(\dfrac{1}{6}\) = \(\dfrac{-30}{6}\) - \(\dfrac{1}{6}\) = \(\dfrac{-31}{6}\)
e; - 3 - \(\dfrac{2}{7}\)= \(\dfrac{-21}{7}\) - \(\dfrac{2}{7}\)= \(\dfrac{-23}{7}\) f; - 3 + \(\dfrac{2}{5}\) = \(\dfrac{-15}{5}\) + \(\dfrac{2}{5}\)= - \(\dfrac{13}{5}\)
g; - 3 - \(\dfrac{2}{3}\) = \(\dfrac{-9}{3}\) - \(\dfrac{2}{3}\) = \(\dfrac{-11}{3}\) h; - 4 - \(\dfrac{-5}{7}\) = \(\dfrac{-28}{7}\)+ \(\dfrac{5}{7}\) = - \(\dfrac{23}{7}\)

1; \(\dfrac{7}{15}\) + \(\dfrac{8}{15}\) = \(\dfrac{7+8}{15}\) = \(\dfrac{15}{15}\) = 1
2; \(\dfrac{1}{2}\) - \(\dfrac{1}{14}\) = \(\dfrac{1.7}{2.7}\) - \(\dfrac{1}{14}\) = \(\dfrac{7-1}{14}\) = \(\dfrac{6}{14}\) = \(\dfrac{3}{7}\)
3; \(\dfrac{8}{28}\) + \(\dfrac{-21}{35}\) = \(\dfrac{2}{7}\) + \(\dfrac{-21}{35}\)= \(\dfrac{10}{35}\) + \(\dfrac{-21}{35}\) = \(\dfrac{-11}{35}\)
4; \(\dfrac{3}{4}\) + \(\dfrac{2}{3}\) - \(\dfrac{9}{6}\) = \(\dfrac{9}{12}\) + \(\dfrac{8}{12}\) - \(\dfrac{18}{12}\) = \(\dfrac{9+8-18}{12}\) = \(\dfrac{-1}{12}\)
5; \(\dfrac{11}{36}\)- \(\dfrac{-7}{-24}\) = \(\dfrac{22}{72}\) + \(\dfrac{21}{72}\) = \(\dfrac{53}{72}\)
6; \(\dfrac{4}{15}\) + \(\dfrac{9}{5}\) - \(\dfrac{7}{3}\) = \(\dfrac{4}{15}\) + \(\dfrac{27}{15}\) - \(\dfrac{35}{15}\) = \(\dfrac{-4}{15}\)

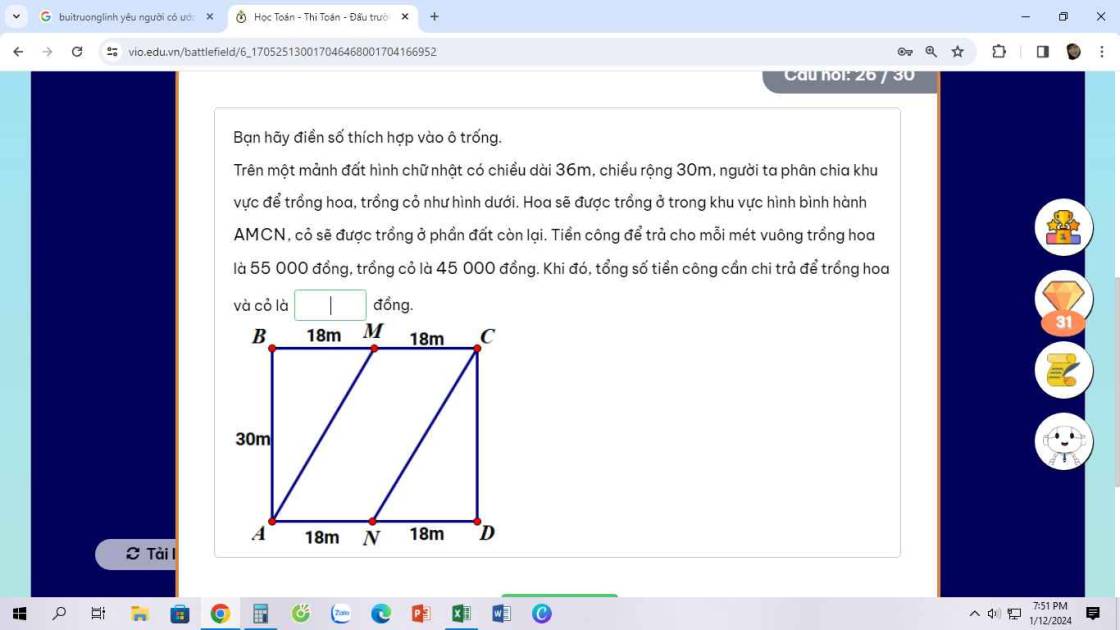
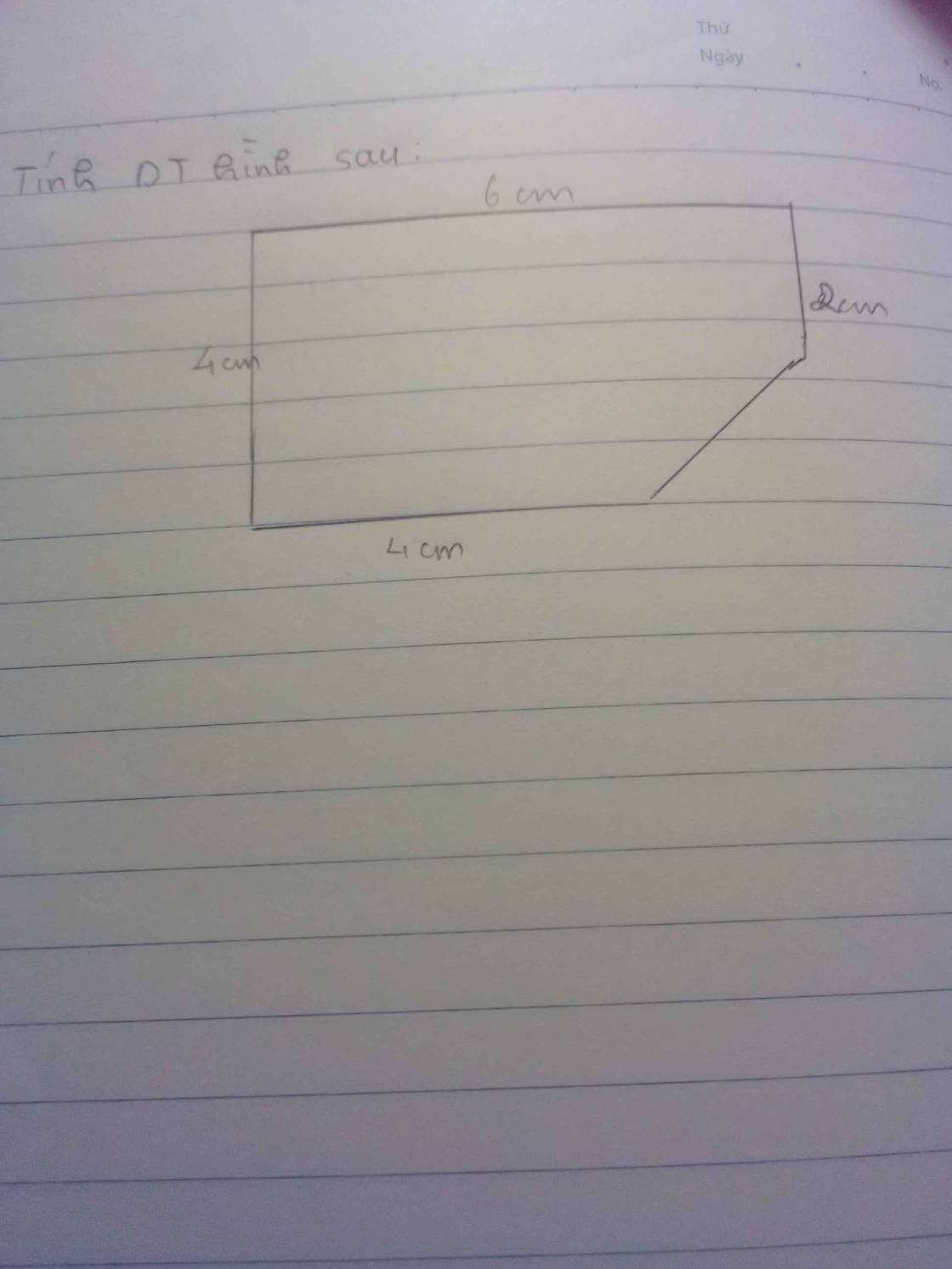
Diện tích mảnh đất là:
\(30\times\left(18+18\right)=1080\left(m^2\right)\)
Diện tích trồng hoa là:
\(30\times18=540\left(m^2\right)\)
Diện tích trồng cỏ là:
\(1080-540=540\left(m^2\right)\)
Tổng tiền cần chi trả là:
\(55000\times540+45000\times540=54000000\) (đồng)
Giải
Diện tích mảnh đất là:
30x(18+18)=1080(m vuông)
Diện tích trồng hoa là:
30x18=540(m vuông)
Diện tích trồng cỏ là:1080-540=540(m vuông)
Tổng số tiền cần chị trả là:
55000x540+45000x540=54000000(đồng)
Chúc bạn học tốt!

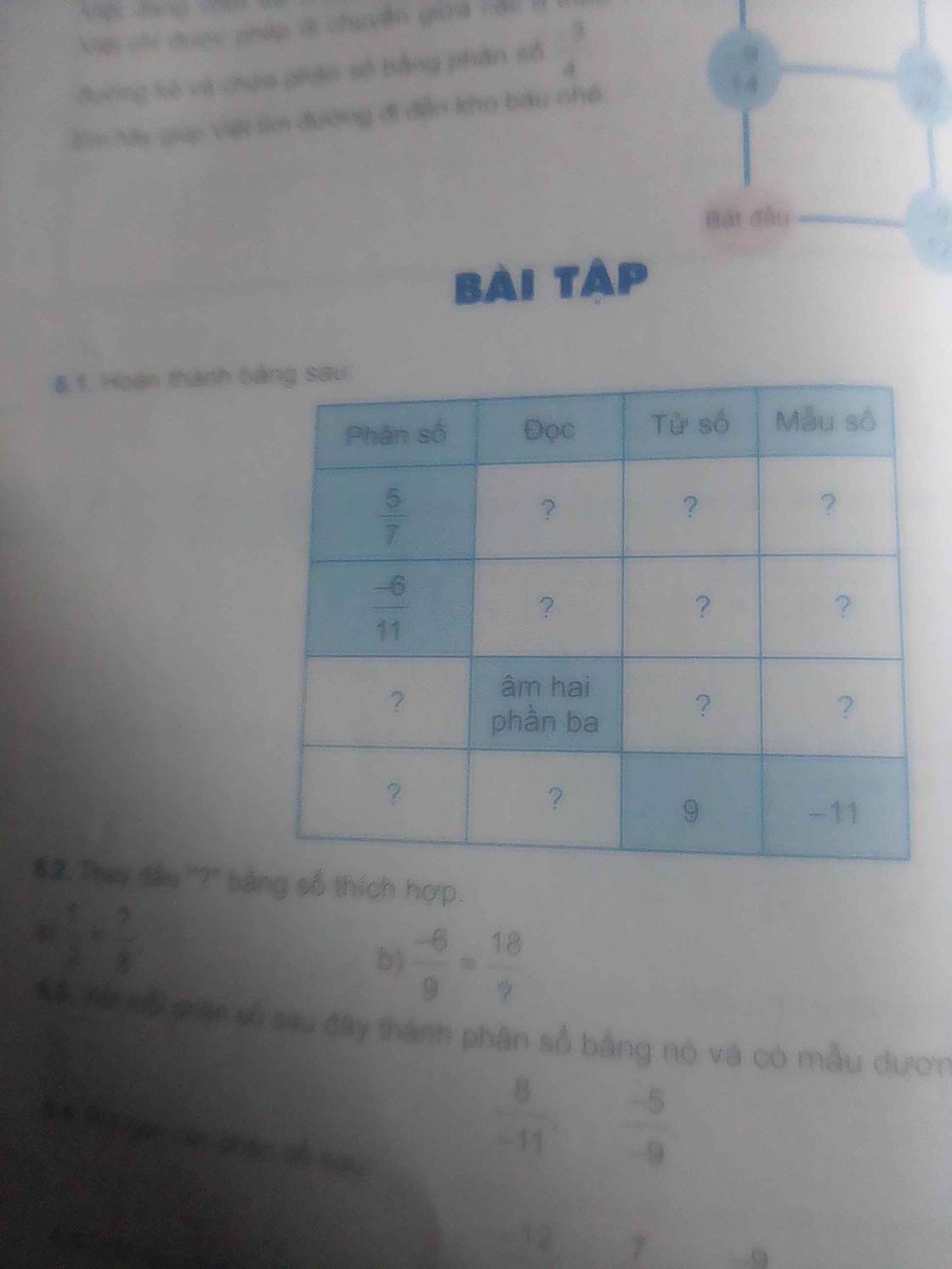
| Phân số | Đọc | Tử Số | Mẫu số |
| \(\dfrac{5}{7}\) | Năm phần bẩy | 5 | 7 |
| \(\dfrac{-6}{11}\) | âm sáu phần mười một | -6 | 11 |
| \(\dfrac{-2}{13}\) | âm hai phần ba | -2 | 13 |
| \(\dfrac{9}{-11}\) | chín phần âm mười một | 9 | -11 |

Bài 4:
\(a,\dfrac{-12}{16}=\dfrac{-12:4}{16:4}=\dfrac{-3}{4};\\ \dfrac{6}{-8}=\dfrac{6:\left(-2\right)}{-8:\left(-2\right)}=\dfrac{-3}{4}\\ Vì:-\dfrac{3}{4}=-\dfrac{3}{4}.Nên:\dfrac{-12}{16}=\dfrac{6}{-8}\\ ---\\ b,.\dfrac{33}{88}=\dfrac{33:11}{88:11}=\dfrac{3}{8}>0;\dfrac{-17}{76}< 0.Nên:-\dfrac{17}{76}< 0< \dfrac{33}{88}.Vậy:\dfrac{-17}{76}\ne\dfrac{33}{88}\)
Mỗi giờ máy bơm thứ nhất bơm vào 1/3 thể tích bể, đồng thời mỗi giờ máy bơm thứ hai hút ra được 1/5 thể tích bể:
Ta có: 1/3 - 1/5 = 5/15 - 3/15 = 2/15 (thể tích bể)
Vậy nếu dùng 2 máy bơm để cùng cấp và thoát nước trong bể 1 giờ thì bể thêm được thể tích là 2/15 bể. Dùng phân số dương nhé!



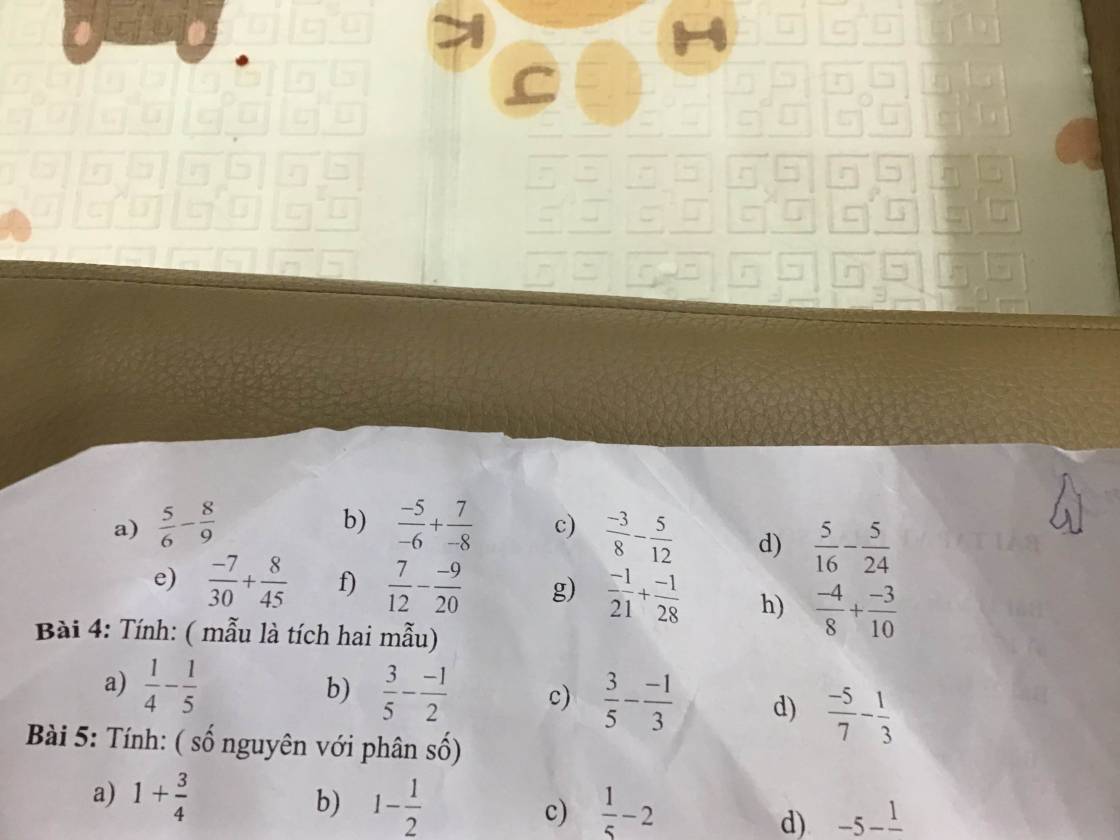
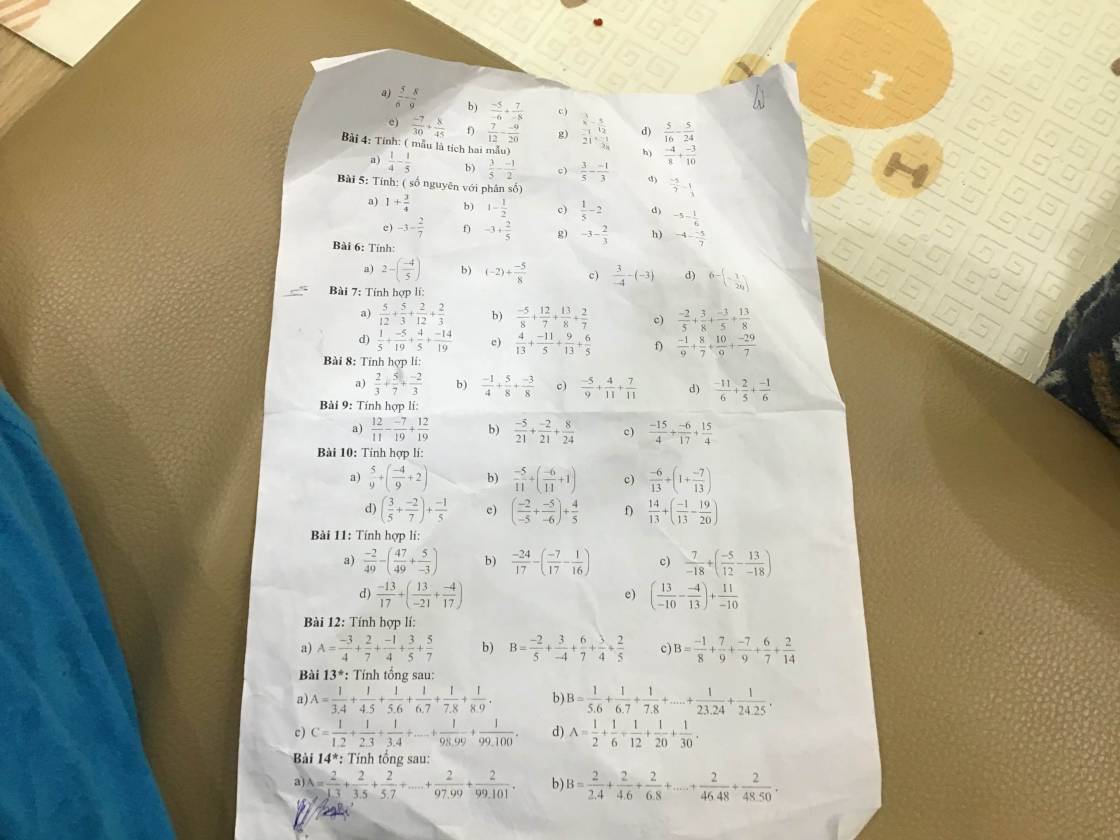
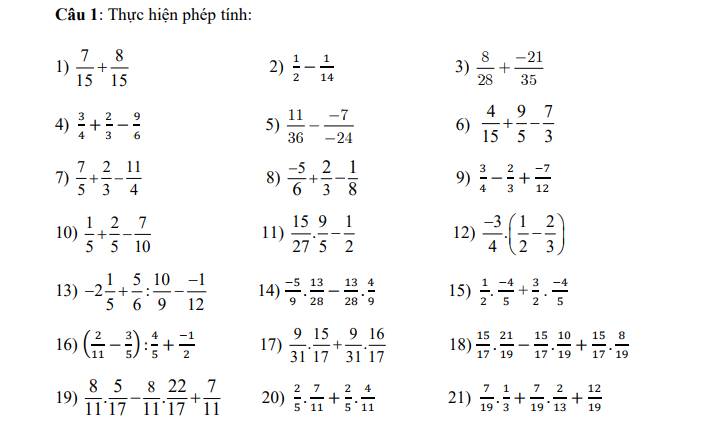

Bài 5:
3xy+6x=1-y
=>\(3x\left(y+2\right)-1+y=0\)
=>\(3x\left(y+2\right)+y+2-3=0\)
=>\(3x\left(y+2\right)+\left(y+2\right)=3\)
=>(y+2)(3x+1)=3
=>\(\left(3x+1\right)\cdot\left(y+2\right)=1\cdot3=3\cdot1=\left(-1\right)\cdot\left(-3\right)=\left(-3\right)\cdot\left(-1\right)\)
=>\(\left(3x+1;y+2\right)\in\left\{\left(1;3\right);\left(3;1\right);\left(-1;-3\right);\left(-3;-1\right)\right\}\)
=>\(\left(3x,y\right)\in\left\{\left(0;1\right);\left(2;-1\right);\left(-2;-5\right);\left(-4;-3\right)\right\}\)
=>\(\left(x,y\right)\in\left\{\left(0;1\right);\left(\dfrac{2}{3};-1\right);\left(-\dfrac{2}{3};-5\right);\left(-\dfrac{4}{3};-3\right)\right\}\)
mà x,y nguyên
nên \(\left(x,y\right)\in\left(0;1\right)\)
Bài 4.2 đâu ạ