K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NT
3
11 tháng 1 2023
a: Xét tứ giác DIHK có
góc DIH=góc DKH=góc KDI=90 độ
nên DIHK là hình chữ nhật
b: Xét tứ giác IHAK có
IH//AK
IH=AK
Do đó: IHAK là hình bình hành
=>B là trung điểm chung của IA và HK
Xét ΔIKA có IC/IK=IB/IA
nên BC//KA
Xét ΔIDA có IB/IA=IM/ID
nên BM//DA
=>B,C,M thẳng hàng
QT
1
HQ
Hà Quang Minh
Giáo viên
11 tháng 9 2023
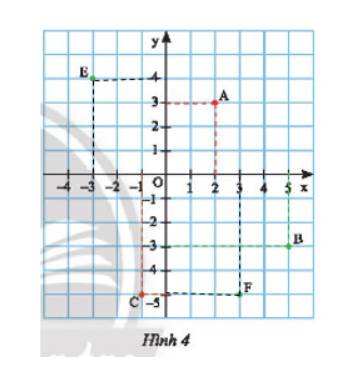
Điểm \(O\) là gốc tọa độ nên \(O\left( {0;0} \right)\)
Từ điểm \(E\) ta vẽ vuông góc với \(Ox;Oy\) cắt \(Ox\) tại – 3 và cắt \(Oy\) tại 4 nên \(E\left( { - 3;4} \right)\).
Từ điểm \(F\) ta vẽ vuông góc với \(Ox;Oy\) cắt \(Ox\) tại 3 và cắt \(Oy\) tại – 5 nên \(E\left( {3; - 5} \right)\).
trong công cụ 
để kiểm tra trung điểm AC và BD có trùng nhau không.
QT
Quoc Tran Anh Le
Giáo viên
14 tháng 1 2024
a) Dùng 
trong công cụ 
để kiểm tra trung điểm AC và BD, ta thấy trung điểm AC và BD trùng nhau.
b) Lưu hình vẽ ở HĐ2 thành tệp hbh.png.
Vào Hồ sơ → Chọn Xuất bản → Chọn PNG image (.png).
Ta đổi tên tệp thành hbh (như hình vẽ), sau đó chọn xuất bản.
Bước 1. Vẽ đoạn thẳng AB và có độ dài 4 cm tương tự như Bước 1 của HĐ1.
Bước 2. Vẽ điểm C sao cho BC = 4 cm.
Chọn công cụ 
→ Chọn 
→ Nháy chuột vào điểm B, nhập bán kính bằng 4.
Chọn công cụ 
→ Chọn 
→ Chọn điểm C bất kỳ nằm trên đường tròn tâm B.
Chọn công cụ 
→ Chọn 
→ Nháy chuột vào điểm C, nhập bán kính bằng 4.
Chọn công cụ 
→ Chọn 
→ Lần lượt nháy chuột đường tròn tâm A và đường tròn C.
Chọn công cụ 
để nối B với C, C với D, D với A.
Bước 3. Ẩn đường tròn và thu được hình thoi ABCD.
11 tháng 8
1: \(\frac{1-a\cdot\sqrt{a}}{1-\sqrt{a}}=\frac{\left(1-\sqrt{a}\right)\left(1+\sqrt{a}+a\right)^{}}{1-\sqrt{a}}=1+\sqrt{a}+a\)
2: \(\frac{\sqrt{x+3}+\sqrt{x-3}}{\sqrt{x+3}-\sqrt{x-3}}=\frac{\left(\sqrt{x+3}+\sqrt{x-3}\right)\left(\sqrt{x+3}+\sqrt{x-3}\right)}{\left(\sqrt{x+3}-\sqrt{x-3}\right)\left(\sqrt{x+3}+\sqrt{x-3}\right)}\)
\(=\frac{\left(\sqrt{x+3}+\sqrt{x-3}\right)^2}{x+3-\left(x-3\right)}=\frac{x+3+x-3+2\sqrt{\left(x+3\right)\left(x-3\right)}}{6}\)
\(=\frac{2x+2\sqrt{x^2-9}}{6}=\frac{x+\sqrt{x^2-9}}{3}\)
4: \(\frac{3}{2\sqrt{9x}}=\frac{3}{2\cdot3\sqrt{x}}=\frac{1}{2\sqrt{x}}=\frac{\sqrt{x}}{2}\)
5: \(\frac{1}{2\sqrt{x}}=\frac{1\cdot\sqrt{x}}{2\sqrt{x}\cdot\sqrt{x}}=\frac{\sqrt{x}}{2x}\)
7: \(\frac{\sqrt{a^3}+a}{\sqrt{a}-1}=\frac{a\cdot\sqrt{a}+a}{\sqrt{a}-1}=\frac{a\left(\sqrt{a}+1\right)}{\sqrt{a}-1}=\frac{a\left(\sqrt{a}+1\right)\left(\sqrt{a}+1\right)}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}\)
\(=\frac{a\left(a+2\sqrt{a}+1\right)}{a-1}=\frac{a^2+2a\cdot\sqrt{a}+a}{a-1}\)
8: \(\frac{2}{\sqrt{a}+\sqrt{2b}}=\frac{2\cdot\left(\sqrt{a}-\sqrt{2b}\right)}{\left(\sqrt{a}+\sqrt{2b}\right)\left(\sqrt{a}-\sqrt{2b}\right)}=\frac{2\sqrt{a}-2\sqrt{2b}}{a-2b}\)
10: \(\frac{25}{\sqrt{a}-\sqrt{b}}=\frac{25\left(\sqrt{a}+\sqrt{b}\right)}{\left(\sqrt{a}-\sqrt{b}\right)\left(\sqrt{a}+\sqrt{b}\right)}=\frac{25\sqrt{a}+25\sqrt{b}}{a-b}\)
11: \(-\frac{ab}{\sqrt{a}-\sqrt{b}}=-\frac{ab\left(\sqrt{a}+\sqrt{b}\right)}{\left(\sqrt{a}-\sqrt{b}\right)\left(\sqrt{a}+\sqrt{b}\right)}=\frac{-ab\cdot\sqrt{a}-ab\cdot\sqrt{b}}{a-b}\)
QT
1
HQ
Hà Quang Minh
Giáo viên
14 tháng 9 2023
- Xét hình 9a và hình 9b ta có:
Tỉ lệ của chiều dài – chiều dài và chiều rộng – chiều rộng của hình 9a và hình 9b lần lượt là:
\(\frac{9}{{7,5}} = 1,2;\frac{5}{5} = 1\). Do đó, không tồn tại hình động dạng phối cảnh nào của hình 9a để bằng hình 9b. Do đó, hình 9a và hình 9b không đồng dạng với nhau.
- Xét hình 9a và hình 9c ta có:
Tỉ lệ của chiều dài – chiều dài và chiều rộng – chiều rộng của hình 9a và hình 9c lần lượt là:
\(\frac{9}{{4,5}} = 2;\frac{5}{{2,5}} = 2\). Do đó, tồn tại hình động dạng phối cảnh của hình 9a bằng hình 9c (hình 9a thu nhỏ với tỉ số 2). Do đó, hình 9a và hình 9c đồng dạng với nhau.
- Xét hình 9a và hình 9d ta có:
Tỉ lệ của chiều dài – chiều dài và chiều rộng – chiều rộng của hình 9a và hình 9d lần lượt là:
\(\frac{9}{{12}} = 0,75;\frac{5}{4} = 1,25\). Do đó, không tồn tại hình động dạng phối cảnh nào của hình 9a để bằng hình 9b. Do đó, hình 9a và hình 9b không đồng dạng với nhau.
+Dùng +trong+công+cụ +để+kiểm+tra+trung+điểm+AC+và+BD+có+trùng+nhau+không.b)+Lưu+hình+vẽ+ở+HĐ2+thành+tệp+hbh.png.c)+Tương+tự,+hãy+vẽ+một+hình+thoi+ABCD+có+cạnh+4+cm.){kind=link}
14 tháng 8
a: Xét ΔABC có
AF,BE,CD là các đường trung tuyến
G là trọng tâm
Do đó: AF,BE,CD đồng quy tại G
Xét tứ giác AGBK có
D là trung điểm chung của AB và KG
=>AGBK là hình bình hành
=>AG//BK và AG=BK
Xét tứ giác AGCH có
E là trung điểm chung của AC và GH
=>AGCH là hình bình hành
=>AG//CH và AG=CH
Ta có: AG//BK
AG//CH
Do đó: BK//CH
ta có: AG=BK
AG=CH
Do đó: BK=CH
Xét tứ giác BKHC có
BK//HC
BK=HC
Do đó: BKHC là hình bình hành
b: Ta có: C,G,D thẳng hàng
G,D,K thẳng hàng
Do đó: C,G,D,K thẳng hàng
=>CK đi qua G
Ta có: B,G,E thẳng hàng
G,E,H thẳng hàng
Do đó: B,G,E,H thẳng hàng
=>BH đi qua G
BCHK là hình bình hành
=>BH cắt CK tại trung điểm của mỗi đường
=>G là trung điểm chung của BH và CK
Hình bình hành BCHK trở thành hình chữ nhật khi KB⊥BC
=>AG⊥BC
=>AF⊥BC
Xét ΔABC có
AF là đường cao
AF là đường trung tuyến
Do đó: ΔABC cân tại A
=>AB=AC
c: Xét ΔANB có
EM//NB
E là trung điểm của AB
=>M là trung điểm của AN
=>AM=MN
Xét ΔDMC có
F là trung điểm của CD
FN//DM
=>N là trung điểm của CM
=>CN=NM=AM
AM+MO=AO
CN+NO=CO
mà AO=CO và AM=Cn
nên MO=NO
=>O là trung điểm của MN
=>M đối xứng N qua O