Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) Hai mặt phẳng cắt nhau, vì 1: 2: (-1) ≠ 2: 3: (-7)
b) Hai mặt phẳng cắt nhau, vì: 1: (-2): 1 ≠ 2: (-1): 4
c) Hai mặt phẳng song song, vì: 1/2=1/2=1/2 ≠ -1/3
d) Hai mạt phẳng cắt nhau, vì: 3: (-2): 3 ≠ 9: (-6): (-9)
e) Hai mặt phẳng trung nhau, vì: 1/10=-1/(-10)=2/20=-4/(-40).
#rin

a) Điều kiện: \(\left\{{}\begin{matrix}4x+2>0\\x-1>0\\x>0\end{matrix}\right.\)
Hay là: \(x>1\)
Khi đó biến đổi pương trình như sau:
\(\ln\dfrac{4x+2}{x-1}=\ln x\)
\(\Leftrightarrow\dfrac{4x+2}{x-1}=x\)
\(\Leftrightarrow4x+2=x\left(x-1\right)\)
\(\Leftrightarrow x^2-5x-2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x_1=\dfrac{5+\sqrt{33}}{2}\\x_2=\dfrac{5-\sqrt{33}}{2}\left(loại\right)\end{matrix}\right.\)
Vậy nghiệm của phương trình là: \(x=\dfrac{5+\sqrt{33}}{2}\)
b) Điều kiện: \(\left\{{}\begin{matrix}3x+1>0\\x>0\end{matrix}\right.\)
Hay là: \(x>0\)
Biến đổi phương trình như sau:
\(\log_2\left(3x+1\right)\log_3x-2\log_2\left(3x+1\right)=0\)
\(\Leftrightarrow\log_2\left(3x+1\right)\left(\log_3x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\log_2\left(3x+1\right)=0\\\log_3x=2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}3x+1=2^0\\x=3^2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\left(loại\right)\\x=9\end{matrix}\right.\)
Vậy nghiệm là x = 9.

Số nghiệm của các phương trình đã cho chính là số giao điểm của đồ thị hàm số y = f(x) ở vế trái của phương trình cới trục hoành ở câu a), b) và với đường thẳng y = -1 ở câu c).
a) Xét hàm số y = x3 – 3x2 + 5 . Tập xác định : R.
y' = 3x2 - 6x = 3x(x - 2); y' = 0 ⇔ x = 0,x = 2.
Bảng biến thiên:

Đồ thị như hình bên.

Từ đồ thị ta thấy phương trình đã cho có nghiệm duy nhất .
b) Xét hàm số y = -2x3 + 3x2 - 2 . Tập xác định : R.
y' = -6x2 + 6x = -6x(x - 1); y' = 0 ⇔ x = 0,x = 1.

Đồ thị như hình bên. Từ đồ thị ta thấy phương trình đã cho có nghiệm duy nhất .
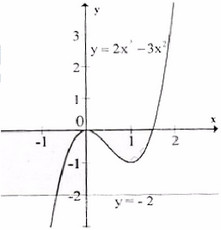
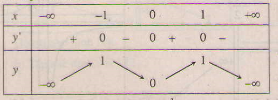
c) Xét hàm số y = f(x) = 2x2 - 2x4. Tập xác định : R.
y' = 4x - 4x3 = 4x(1 - x2); y' = 0 ⇔ x = 0,x = ±1.
Bảng biến thiên:
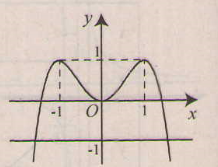
Đồ thị hàm số f(x) và đường thẳng y = -1 như hình bên.
Từ đồ thị ta thấy phương trình đã cho có hai nghiệm phân biệt.

a/ ĐKXĐ: \(x>\frac{1}{2}\)
\(\Leftrightarrow\frac{3x^2-1}{\sqrt{2x-1}}-\sqrt{2x-1}=mx\)
\(\Leftrightarrow\frac{3x^2-2x}{\sqrt{2x-1}}=mx\Leftrightarrow\frac{3x-2}{\sqrt{2x-1}}=m\)
Đặt \(\sqrt{2x-1}=a>0\Rightarrow x=\frac{a^2+1}{2}\Rightarrow\frac{3a^2-1}{2a}=m\)
Xét hàm \(f\left(a\right)=\frac{3a^2-1}{2a}\) với \(a>0\)
\(f'\left(a\right)=\frac{12a^2-2\left(3a^2-1\right)}{4a^2}=\frac{6a^2+2}{4a^2}>0\)
\(\Rightarrow f\left(a\right)\) đồng biến
Mặt khác \(\lim\limits_{a\rightarrow0^+}\frac{3a^2-1}{2a}=-\infty\); \(\lim\limits_{a\rightarrow+\infty}\frac{3a^2-1}{2a}=+\infty\)
\(\Rightarrow\) Phương trình đã cho luôn có nghiệm với mọi m
b/ ĐKXĐ: \(x\ge2\)
\(\Leftrightarrow\sqrt[4]{\left(x-1\right)^2}+4m\sqrt[4]{\left(x-1\right)\left(x-2\right)}+\left(m+3\right)\sqrt[4]{\left(x-2\right)^2}=0\)
Nhận thấy \(x=2\) không phải là nghiệm, chia 2 vế cho \(\sqrt[4]{\left(x-2\right)^2}\) ta được:
\(\sqrt[4]{\left(\frac{x-1}{x-2}\right)^2}+4m\sqrt[4]{\frac{x-1}{x-2}}+m+3=0\)
Đặt \(\sqrt[4]{\frac{x-1}{x-2}}=a\) pt trở thành: \(a^2+4m.a+m+3=0\) (1)
Xét \(f\left(x\right)=\frac{x-1}{x-2}\) khi \(x>0\)
\(f'\left(x\right)=\frac{-1}{\left(x-2\right)^2}< 0\Rightarrow f\left(x\right)\) nghịch biến
\(\lim\limits_{x\rightarrow2^+}\frac{x-1}{x-2}=+\infty\) ; \(\lim\limits_{x\rightarrow+\infty}\frac{x-1}{x-2}=1\) \(\Rightarrow f\left(x\right)>1\Rightarrow a>1\)
\(\left(1\right)\Leftrightarrow m\left(4a+1\right)=-a^2-3\Leftrightarrow m=\frac{-a^2-3}{4a+1}\)
Xét \(f\left(a\right)=\frac{-a^2-3}{4a+1}\) với \(a>1\)
\(f'\left(a\right)=\frac{-2a\left(4a+1\right)-4\left(-a^2-3\right)}{\left(4a+1\right)^2}=\frac{-4a^2-2a+12}{\left(4a+1\right)^2}=0\Rightarrow a=\frac{3}{2}\)
\(f\left(1\right)=-\frac{4}{5};f\left(\frac{3}{2}\right)=-\frac{3}{4};\) \(\lim\limits_{a\rightarrow+\infty}\frac{-a^2-3}{4a+1}=-\infty\)
\(\Rightarrow f\left(a\right)\le-\frac{3}{4}\Rightarrow m\le-\frac{3}{4}\)

a) Bất phương trình đã cho tương đương với hệ sau:
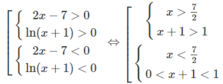
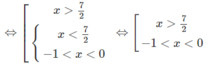
Vậy tập nghiệm là (−1;0) ∪ (7/2; + ∞ )
b) Tương tự câu a), tập nghiệm là (1/10; 5)
c) Đặt t = log 2 x , ta có bất phương trình 2 t 3 + 5 t 2 + t – 2 ≥ 0 hay (t + 2)(2 t 2 + t − 1) ≥ 0 có nghiệm −2 ≤ t ≤ −1 hoặc t ≥ 1/2
Suy ra 1/4 ≤ x ≤ 1/2 hoặc x ≥ 2
Vậy tập nghiệm của bất phương trình đã cho là: [1/4; 1/2] ∪ [ 2 ; + ∞ )
d) Bất phương trình đã cho tương đương với hệ:
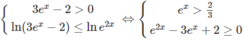
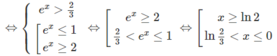
Vậy tập nghiệm là (ln(2/3); 0] ∪ [ln2; + ∞ )
b) |x + 4| = 2x - 5 ⇔ x + 4 = 2x - 5 khi x + 4 ≥ 0 ⇔ x ≥ -4
⇔ x = 9 ( thoả mãn điều kiện x ≥ -4)
|x + 4| = 2x - 5 ⇔ -x - 4 = 2x - 5 khi x + 4 < 0 ⇔ x < -4
⇔ 3x = 1
⇔ x = (không thoả mãn điều kiện x < -4)
(không thoả mãn điều kiện x < -4)
Vậy phương trình có nghiệm x = 9
d) |x - 4| + 3x = 5
|x - 4| + 3x = 5 ⇔ x - 4 + 3x = 5 khi x ≥ 4
⇔ 4x = 9
⇔ x = (không thoả mãn điều kiện x ≥ 4)
(không thoả mãn điều kiện x ≥ 4)
|x - 4| + 3x = 5 ⇔ -x + 4 + 3x = 5 khi x < 4
⇔ 2x = 1
⇔ x =