
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
PT
1

Các câu hỏi dưới đây có thể giống với câu hỏi trên
SG
1

17 tháng 5 2017
\(4\sin3x+\sin5x-2\sin x\cos2x=0\)
\(\Leftrightarrow\)\(4\sin3x+\sin5x-\sin3x+\sin x=0\)
\(\Leftrightarrow3\sin3x+\sin5x+\sin x=0\)
\(\Leftrightarrow3\sin3x+2\sin3x\cos2x=0\)
\(\Leftrightarrow\sin3x\left(3+2\cos2x\right)=0\)
Đáp số : \(x=k\dfrac{\pi}{3},k\in\mathbb{Z}\)
TD
17
PT
1
PT
1

CM
27 tháng 11 2017
sin3x + sin5x = 0
⇔ 2sin4x. cosx = 0
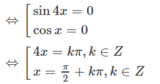
Vậy nghiệm của phương trình là:
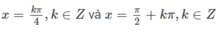
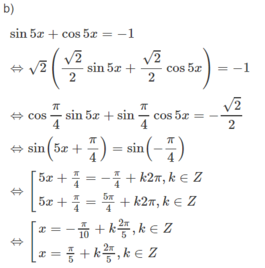








cosx.tan3x = sin5x
Điều kiện: cos3x ≠ 0. Khi đó,
(3)⇔ cosx.sin3x = cos3x.sin5x
Kết hợp với điều kiện ta được nghiệm của phương trình là: